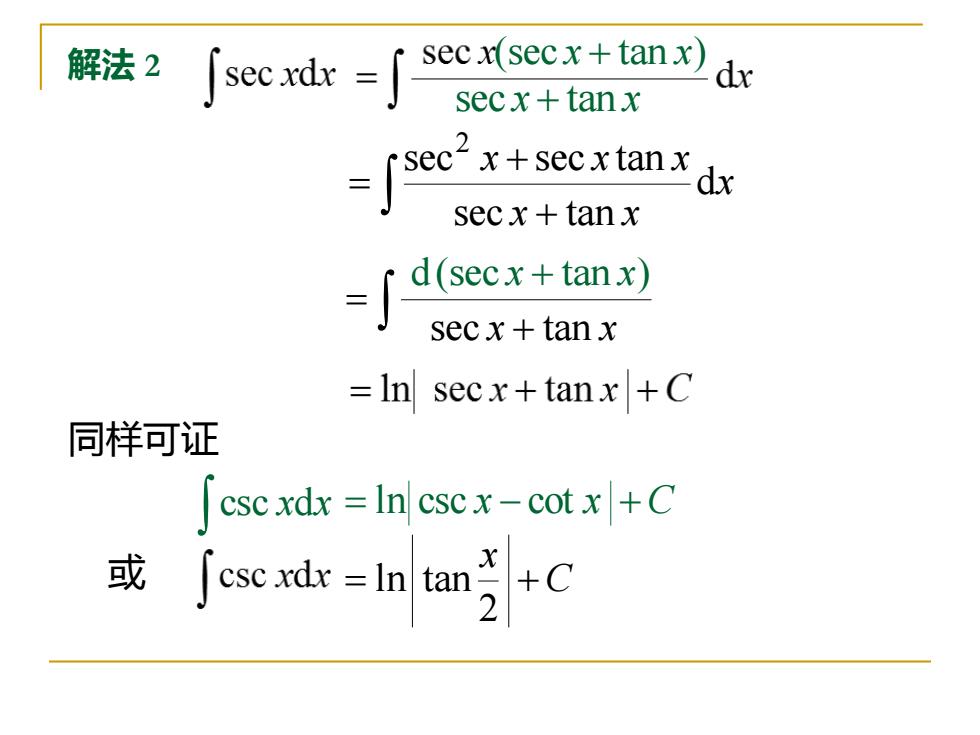
解法2 ∫secxx=∫ secx(secx+tanx) dx secx+tanx =∫Scf+secxtanx secx+tanx d(secx+tanx) secx+tanx =In secx+tanx +C 同样可证 csc xdx Incscx-cot x+C 或 ∫cd=lnam2+C
+ = sec x tan x 解法 2 sec x + tan x (sec x + tan x) x x x x x x d sec tan sec sec tan 2 + + = d(sec x + tan x) 同样可证 csc xdx = ln csc x − cot x +C 或 C x = + 2 ln tan

例13求∫tan3xsec3xdx 例14求「tanxdx. 例15设∫f(x)dx=arccosx+C,则 dc
例13 求 5 3 tan sec d x x x 例14 求 4 tan dx x . xf x x x C ( )d arccos = + 1 d ( ) x f x = 例15 设 ,则 . 3 2 2 1 (1 ) 3 − + x C