
定理1β与α是等价无穷小的的充分必要条件为β=α+o(α).称α是β 的主部证必要性设α~β,ββ-α-1=0,limlimααβ-α=o(α), 即β=α+o(α)充分性设β=α+o(α)α+ 0(α) = lim( 1 + 0(α)) ==1limlimαααα~β
( ) 1 o . 定理 与 是等价无穷小的的充分必要条件 为 称 是 的主部. 证 必要性 设 ~ , lim lim 1 0, o(),即 o(). 充分性 设 o(). ( ) lim lim o (1+ ) ( ) lim o 1, ~ .

意义:用等价无穷小可给出函数的近似表达式例如,当x→0时,sinx~x,1-cosx2sin x = x + o(x),1=2x2 + 0(x2).1-cosx=2y=1-cosx3常用等价无穷小:当x→0时x ~ sinx ~ tanx ~ arcsinx ~ arctanx ~ In(1+ x),(1+x)"-1~ax (a±0)x~e-1, 1-cosx~2
意义:用等价无穷小可给出函数的近似表达式. 例如, sin x x o(x), ( ). 2 1 1 cos 2 2 x x o x 当x 0时, y 1 cos x 2 2 1 y x 常用等价无穷小: 当x 0时, , (1 ) 1 ~ ( 0) 2 1 ~ 1, 1 cos ~ ~ sin ~ tan ~ arcsin ~ arctan ~ ln(1 ) 2 x e x x x ax a x x x x x x x a . 2 1 sin ~ , 1 cos ~ 2 x x x x
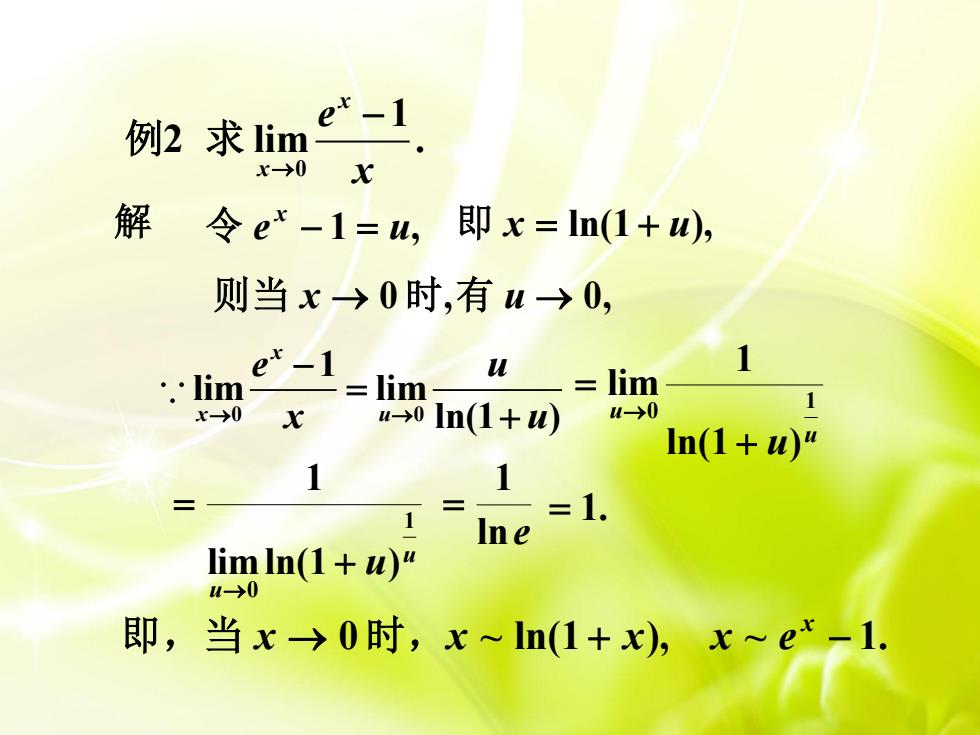
et例2 求 limx→0 x解 令e*-l=u,即 x = In(1 + u),则当x→0时,有u→0,1et-1ulimlimlim-1u-→0x-→0u-→0In(1 + u)xIn(1 + u)"111=1Inelim In(1 + u)"u-→0即,当x→0时,x~ln(1+x),x~e*-l
解 0 0 1 lim lim ln(1 ) x x u e u x u 0 1 2 lim . x x e x 例 求 e 1 u, x 令 即 x ln(1 u), 则当 x 0 时,有 u 0, u u u 1 0 ln(1 ) 1 lim u u u 1 0 limln(1 ) 1 lne 1 1. 0 ~ ln(1 ), ~ 1. x 即,当 x 时 ,x x x e

例3 证明:当x→0时,/1+x-1~1xnr/1+x -1证:lim1xx-0a" - b" = (a-b)(an-1 +an-2b+...+bn-1(/1+x)"-1= limx-→0x[("/1+x )"-+ +(/1+x )"- +..+1]n=1: 当x→0时,/1+x-1~=xn
证: 1 0 1 1 lim n x n x x 1 2 0 1 1 1 lim 1 1 1 n n n n x n n n x x x x L 1 1 0 , 1 1 n x x x n 当 时 : 1 2 1 ( )( ) n n n n n a b a b a a b b L 1 3 0 , 1 1 n x x x n 例 证明:当 时