
强数学归纳法(有效性) {neZ|n≥a}是良序的 口良序集:该集合的非空子集都有一个最小元素 口数学归纳法的有效性(归谬法) 口假设nP(nm)不成立,则3n(-P(n)成立. 口令S={n∈Z(n2m)AP(n)},S是非空子集. 口根据良序公理,S有最小元素,记为m,心u ▣a,,(m-1)eS,即P(a,,P(m-1)成立. 口根据归纳步骤,P(m成立,即m廷S,矛盾. a因此,nP(成立
{ nZ | n a }是良序的 良序集:该集合的非空子集都有一个最小元素 数学归纳法的有效性(归谬法) 假设n P(n)不成立,则n (P(n))成立. 令S={ n | (na) P(n) },S是非空子集. 根据良序公理,S有最小元素,记为m, m>a a, …, (m-1)S, 即P(a), …, P(m-1)成立. 根据归纳步骤,P(m)成立,即mS,矛盾. 因此,n P(n)成立. 强数学归纳法(有效性)

强数学归纳法(举例) 口任意整数n(n≥2)可分解为(若干个)素数的乘积 口n=2. 口考察n+1. 口用4分和5分就可以组成12分及以上的每种邮资. 口P(12),P(13),P(14),P(15). ▣对任意k≥15,P(12)Λ..∧P(→P(k+1)
任意整数n(n 2)可分解为(若干个)素数的乘积 n = 2. 考察 n+1. 用4分和5分就可以组成12分及以上的每种邮资. P(12), P(13), P(14), P(15). 对任意k 15, P(12)… P(k)→P(k+1) 强数学归纳法(举例)

Odd Pie Fights Placing an odd number of people in the plane,in such a way that every pair of people has a distinct distance between them.At a signal,each person will throw a pie at the closest other person. At least one person does not get hit with a pie?
Placing an odd number of people in the plane, in such a way that every pair of people has a distinct distance between them. At a signal, each person will throw a pie at the closest other person. At least one person does not get hit with a pie? Odd Pie Fights
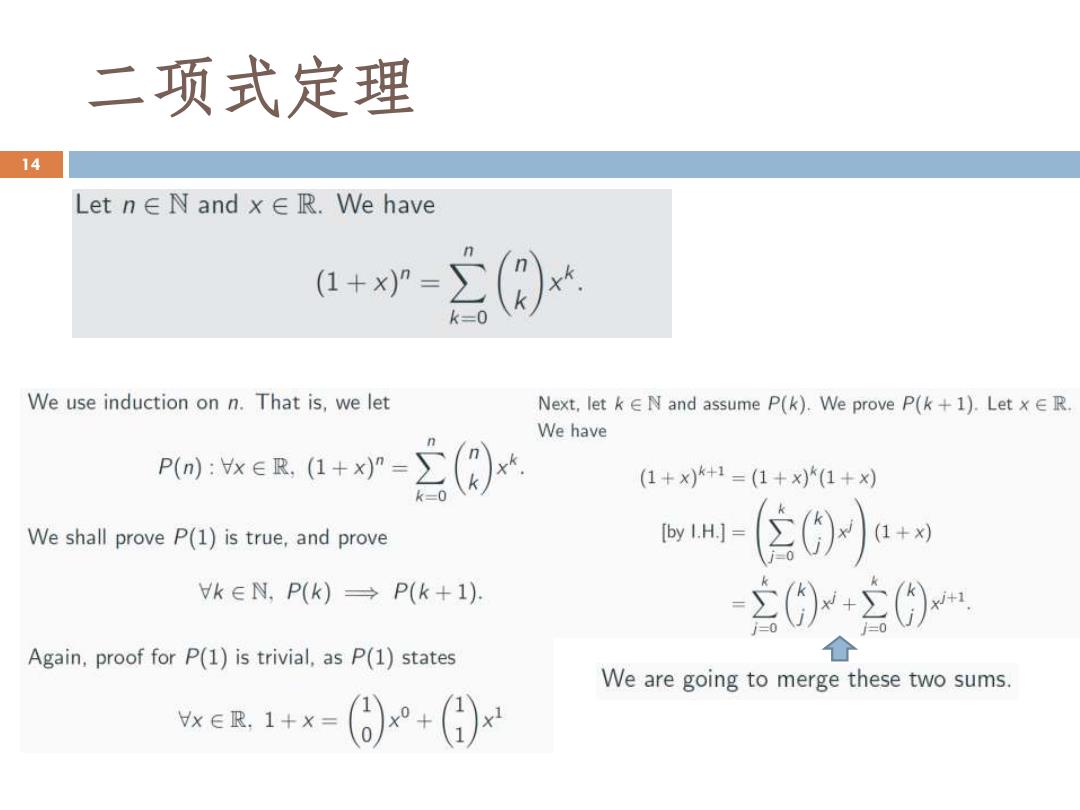
二项式定理 14 Letn∈N and x∈R.Ve have +=()× k=0 We use induction on n.That is,we let Next.let k EN and assume P(k).We prove P(k+1).Let x ER. We have Po:xe其a+r-() (1+x)*+1=(1+x)(1+x) We shall prove P(1)is true,and prove H-(C)a+ k∈N,P(k)→P(k+1) )* Again,proof for P(1)is trivial,as P(1)states We are going to merge these two sums. x∈R,1+×=
二项式定理 14

二项式定理 15 To get an idea,let us consider an explicit example when k=2: Keep in mind that we want to prove this is equal to which is 三0)+2C0 ()+ =[)+()x+)+[6x+)+) -+[份+(x+[)+)+份) Therefore,to finish the proof,it suffices to show that C)+()=( In general,we have )+0) C份)+()-+0-西 k k =1+C的+()+ k幻 k+1 =G-1川(k-k-j+可 =1+C份+(+ =()
二项式定理 15