
Introduction min50x+20x2+30x3+80x4 s.t.400x1+200x2+150x3+500x4 500 (Calorie constraint) 3x1+2x为2≥6(Chocolate constraint) 2x灯+2x2+4x3+4x4≥10(Sugar constraint) 2x1+4x2+x为+5x4≥8(Fat constraint) xi >0(i=1,2,3,4)(Sign restrictions) The optimal solution to this LP is x1 =x4 =0,x2 =3,x3 =1,with z=90.The optimal diet indicates that 200(3)+150(1)=750 calories 2(3)=6 oz of chocolate 2(3)+4(1)=10 oz of sugar 4(3)+1(1)=13 oz of fat Thus,the chocolate and sugar constraints are binding,but the calories and fat constraints are nonbinding. Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 16/148
Introduction min 50x1 + 20x2 + 30x3 + 80x4 s.t. 400x1 + 200x2 + 150x3 + 500x4 ≥ 500 (Calorie constraint) 3x1 + 2x2 ≥ 6 (Chocolate constraint) 2x1 + 2x2 + 4x3 + 4x4 ≥ 10 (Sugar constraint) 2x1 + 4x2 + x3 + 5x4 ≥ 8 (Fat constraint) xi ≥ 0 (i = 1, 2, 3, 4) (Sign restrictions) The optimal solution to this LP is x1 = x4 = 0, x2 = 3, x3 = 1, with z = 90. The optimal diet indicates that 200(3) + 150(1) = 750 calories 2(3) = 6 oz of chocolate 2(3) + 4(1) = 10 oz of sugar 4(3) + 1(1) = 13 oz of fat Thus, the chocolate and sugar constraints are binding, but the calories and fat constraints are nonbinding. Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 16 / 148
Introduction Example 4(A Work-Scheduling Problem) Self-learning Example 5(A Capital Budgeting Problem) Self-learning Example 6(Short-Term Financial Planning) Self-learning Example 7(Blending Problems) Self-learning Example 8(Production Process Models) Self-learning 4口,4+4立4至,三只0 Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 17/148
Introduction Example 4 (A Work-Scheduling Problem) Self-learning Example 5 (A Capital Budgeting Problem) Self-learning Example 6 (Short-Term Financial Planning) Self-learning Example 7 (Blending Problems) Self-learning Example 8 (Production Process Models) Self-learning Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 17 / 148
Introduction Example 9(An Inventory Model) Self-learning Example 10(Multiperiod Financial Models) Self-learning Example 11(Multiperiod Work Scheduling) Self-learning 4口4+4三4至,至)只0 Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 18/148
Introduction Example 9 (An Inventory Model) Self-learning Example 10 (Multiperiod Financial Models) Self-learning Example 11 (Multiperiod Work Scheduling) Self-learning Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 18 / 148

The Simplex Method Introduction ②The Simplex Method Sensitivity Analysis Duality Theory 4口,4得+4艺至,三)风0 Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 19/148
The Simplex Method 1 Introduction 2 The Simplex Method 3 Sensitivity Analysis 4 Duality Theory Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 19 / 148
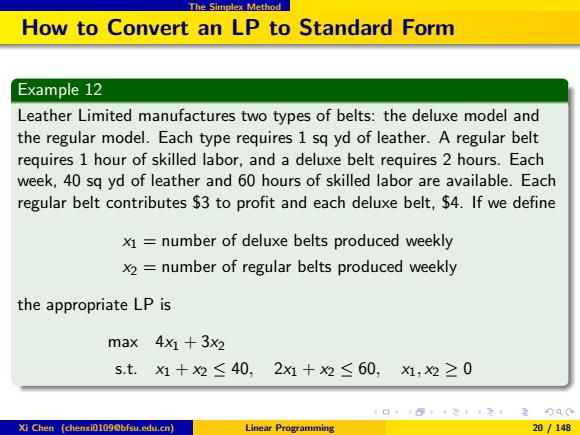
The Simplex Method How to Convert an LP to Standard Form Example 12 Leather Limited manufactures two types of belts:the deluxe model and the regular model.Each type requires 1 sq yd of leather.A regular belt requires 1 hour of skilled labor,and a deluxe belt requires 2 hours.Each week,40 sq yd of leather and 60 hours of skilled labor are available.Each regular belt contributes $3 to profit and each deluxe belt,$4.If we define x1=number of deluxe belts produced weekly x2=number of regular belts produced weekly the appropriate LP is max 4为+3x2 5.t.x1+2≤40,2x灯+x2≤60,x1,x2≥0 Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 20/148
The Simplex Method How to Convert an LP to Standard Form Example 12 Leather Limited manufactures two types of belts: the deluxe model and the regular model. Each type requires 1 sq yd of leather. A regular belt requires 1 hour of skilled labor, and a deluxe belt requires 2 hours. Each week, 40 sq yd of leather and 60 hours of skilled labor are available. Each regular belt contributes $3 to profit and each deluxe belt, $4. If we define x1 = number of deluxe belts produced weekly x2 = number of regular belts produced weekly the appropriate LP is max 4x1 + 3x2 s.t. x1 + x2 ≤ 40, 2x1 + x2 ≤ 60, x1, x2 ≥ 0 Xi Chen (chenxi0109@bfsu.edu.cn) Linear Programming 20 / 148