
电子种越女学 University of Electroe Scioncad TechofChina 986 Chapter 11 Scaling and Roundoff Noise Xiang LING National Key Lab of Science and Technology on Communications
Chapter 11 Scaling and Roundoff Noise Xiang LING National Key Lab of Science and Technology on Communications
11.1 Introduction 96 In a fixed-point digital filter implementation,the overall input-output behavior is non-ideal due to quantization of signals and coefficients. There are two basic types of quantization effects in any implementation. Coefficient quantization Signal rounding Limit-cycle oscillation ■Roundoff noise 2021年2月 2
2021年2月 2 11.1 Introduction In a fixed-point digital filter implementation, the overall input-output behavior is non-ideal due to quantization of signals and coefficients. There are two basic types of quantization effects in any implementation. Coefficient quantization Signal rounding Limit-cycle oscillation Roundoff noise
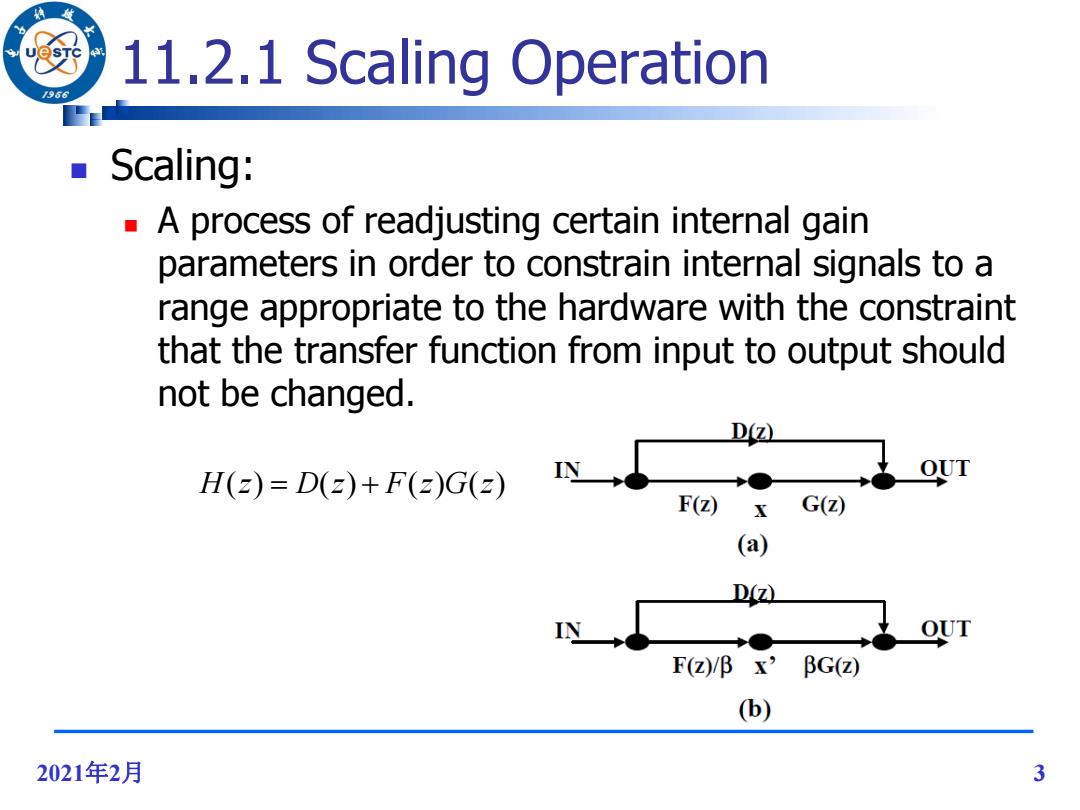
11.2.1 Scaling Operation /96 Scaling: -A process of readjusting certain internal gain parameters in order to constrain internal signals to a range appropriate to the hardware with the constraint that the transfer function from input to output should not be changed. D(Z) H()=D()+F()G() IN OUT F(Z) G(Z) (a) D(Z) OUT F(Z)/B x' BG(Z (b) 2021年2月 3
2021年2月 3 11.2.1 Scaling Operation Scaling: A process of readjusting certain internal gain parameters in order to constrain internal signals to a range appropriate to the hardware with the constraint that the transfer function from input to output should not be changed. H(z) D(z) F(z)G(z)
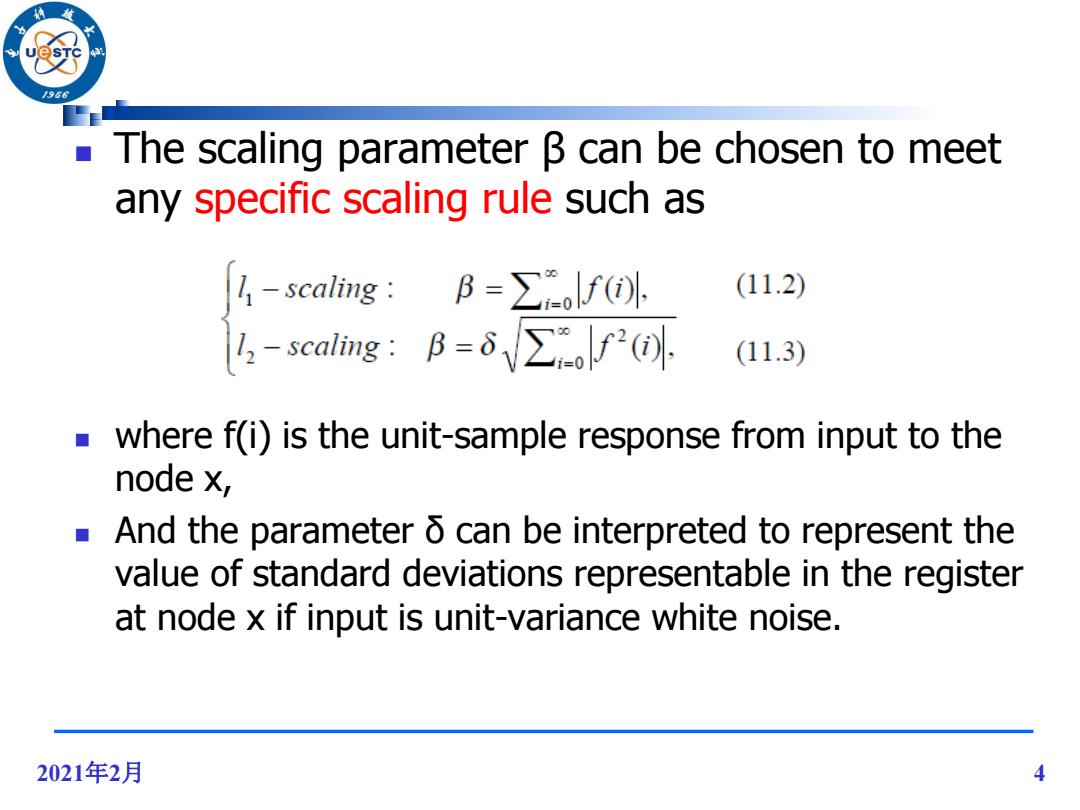
/966 The scaling parameter B can be chosen to meet any specific scaling rule such as h-scaling:B=∑of(l (11.2) 12-scaling:B=δV∑f2(l (11.3) where f(i)is the unit-sample response from input to the node x, ■ And the parameter 6 can be interpreted to represent the value of standard deviations representable in the register at node x if input is unit-variance white noise. 2021年2月 4
2021年2月 4 The scaling parameter β can be chosen to meet any specific scaling rule such as where f(i) is the unit-sample response from input to the node x, And the parameter δ can be interpreted to represent the value of standard deviations representable in the register at node x if input is unit-variance white noise
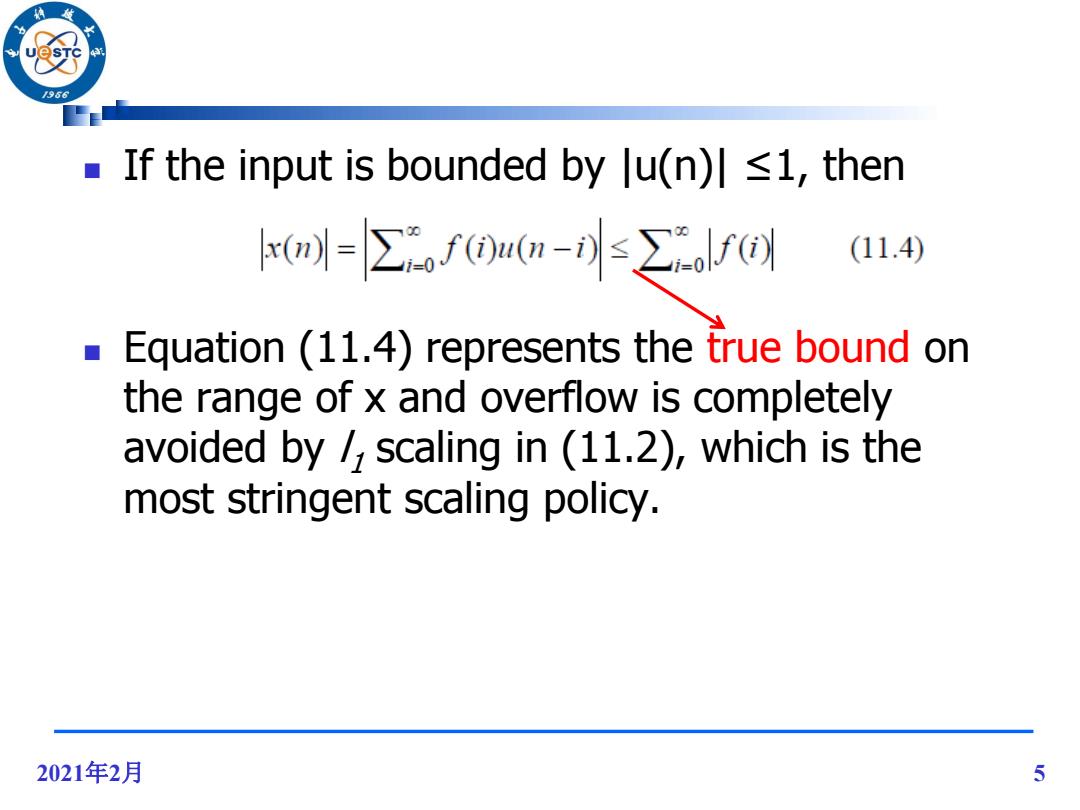
/96 ■If the input is bounded by |u(n)l≤l,then ron=|∑f0un-sofo间 (11.4) ■ Equation (11.4)represents the true bound on the range of x and overflow is completely avoided by /scaling in (11.2),which is the most stringent scaling policy. 2021年2月 5
2021年2月 5 If the input is bounded by |u(n)| ≤1, then Equation (11.4) represents the true bound on the range of x and overflow is completely avoided by l1 scaling in (11.2), which is the most stringent scaling policy