
Philosophy of science 24 for a radical divergence between the law and its instances.And so such a law is one which could not be a regularity of the sort required by the minimalist.This would appear to be a damning argument against minimalists,not even allowing for an amendment of their position by the addition of extra conditions.Nonetheless,I think the argument employed against the minimalist on this point could be seen as simply begging the question.The argument starts by considering an individual particle subject to a probabilistic law.The law might be that such particles have a half-life of time t.The argument points out that this law is consistent with the particle decaying at time t*after t. This is all true.The argument then proceeded to claim that any collection could, therefore,be such that all its particles decay after t,including the collection of all the particles over all time. The form of this argument is this:what is possible for any individual particle is possible for a collection of particles;or.more generally,if it is possible that X.and it is possible that Y,and it is possible that Z.and so on,then it is possible that X and Y and Z. etc.This form of argument certainly is not logically valid.Without looking outside,I think to myself it is possible that it is raining and it is possible that it is not raining,but I certainly should not conclude that it is possible that it is both raining and not raining. More pertinent to this issue is another counter-example.It is possible for one person to be taller than average,but it is not possible for everyone to be taller than average.This is because the notion of an average is logically related to the properties of a whole group. What this suggests is that the relevant step in the argument against the minimalist could only be valid if there is a logical gap between a law and the collection of its instances. But this is precisely what the minimalist denies.For,on the contrary,the minimalist claims that there is no gap;rather there is an intimate logical link in that the probabilistic law is some sort of sophisticated averaging out over its instances.So it turns out that the contentious step in the argument is invalid or.at best,question begging against the minimalist. Still,even if the argument is invalid,it does help alert our intuitions to an implausibility with minimalism.We may not be able to prove that there can be a gap between the chance of decay that each atom has and the proportion of particles actually decaying-but the fact that such a gap does seem possible is evidence against the minimalist. The systematic account of laws of nature We have seen so far that not all regularities are also laws,though it is possible for the minimalist to resist the argument that not all laws are regularities.What the minimalist needs to do is to find some further conditions,in addition to being a regularity,which will pare down the class of regularities to just those that are laws. Recall that the minimalist wants laws simply to generalize over their instances.Law statements will be summaries of the facts about those instances.If this is right we should not expect every regularity to be a law.For we can summarize a set of facts without having to mention every regularity that they display,and an efficient summary would only record sufficient regularities to capture all the facts in which we are interested.Such a summary would be systematic;rather than being a list of separate regularities,it would
for a radical divergence between the law and its instances. And so such a law is one which could not be a regularity of the sort required by the minimalist. This would appear to be a damning argument against minimalists, not even allowing for an amendment of their position by the addition of extra conditions. Nonetheless, I think the argument employed against the minimalist on this point could be seen as simply begging the question. The argument starts by considering an individual particle subject to a probabilistic law. The law might be that such particles have a half-life of time t. The argument points out that this law is consistent with the particle decaying at time t* after t. This is all true. The argument then proceeded to claim that any collection could, therefore, be such that all its particles decay after t, including the collection of all the particles over all time. The form of this argument is this: what is possible for any individual particle is possible for a collection of particles; or, more generally, if it is possible that X, and it is possible that Y, and it is possible that Z, and so on, then it is possible that X and Y and Z, etc. This form of argument certainly is not logically valid. Without looking outside, I think to myself it is possible that it is raining and it is possible that it is not raining, but I certainly should not conclude that it is possible that it is both raining and not raining. More pertinent to this issue is another counter-example. It is possible for one person to be taller than average, but it is not possible for everyone to be taller than average. This is because the notion of an average is logically related to the properties of a whole group. What this suggests is that the relevant step in the argument against the minimalist could only be valid if there is a logical gap between a law and the collection of its instances. But this is precisely what the minimalist denies. For, on the contrary, the minimalist claims that there is no gap; rather there is an intimate logical link in that the probabilistic law is some sort of sophisticated averaging out over its instances. So it turns out that the contentious step in the argument is invalid or, at best, question begging against the minimalist. Still, even if the argument is invalid, it does help alert our intuitions to an implausibility with minimalism. We may not be able to prove that there can be a gap between the chance of decay that each atom has and the proportion of particles actually decaying—but the fact that such a gap does seem possible is evidence against the minimalist. The systematic account of laws of nature We have seen so far that not all regularities are also laws, though it is possible for the minimalist to resist the argument that not all laws are regularities. What the minimalist needs to do is to find some further conditions, in addition to being a regularity, which will pare down the class of regularities to just those that are laws. Recall that the minimalist wants laws simply to generalize over their instances. Law statements will be summaries of the facts about those instances. If this is right we should not expect every regularity to be a law. For we can summarize a set of facts without having to mention every regularity that they display, and an efficient summary would only record sufficient regularities to capture all the facts in which we are interested. Such a summary would be systematic; rather than being a list of separate regularities, it would Philosophy of science 24
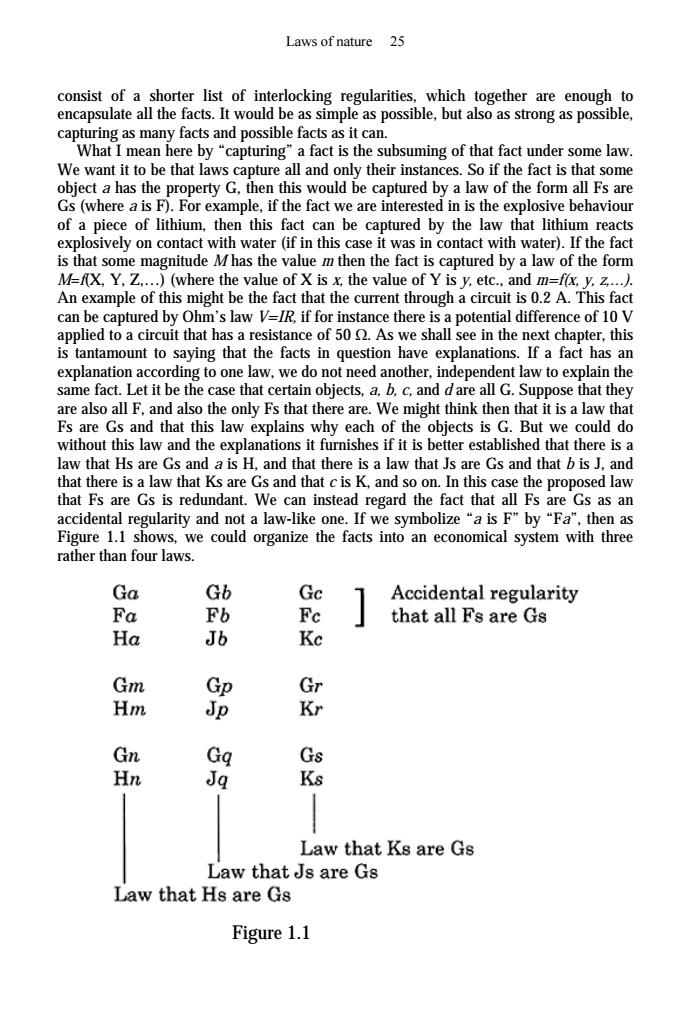
Laws of nature 25 consist of a shorter list of interlocking regularities,which together are enough to encapsulate all the facts.It would be as simple as possible,but also as strong as possible, capturing as many facts and possible facts as it can. What I mean here by"capturing"a fact is the subsuming of that fact under some law. We want it to be that laws capture all and only their instances.So if the fact is that some object a has the property G.then this would be captured by a law of the form all Fs are Gs(where a is F).For example,if the fact we are interested in is the explosive behaviour of a piece of lithium,then this fact can be captured by the law that lithium reacts explosively on contact with water(if in this case it was in contact with water).If the fact is that some magnitude Mhas the value m then the fact is captured by a law of the form M=AX,Y,Z....)(where the value of X is x the value of Y is y.etc.,and m=fx.y.z...). An example of this might be the fact that the current through a circuit is 0.2 A.This fact can be captured by Ohm's law V=IR,if for instance there is a potential difference of 10 V applied to a circuit that has a resistance of 50 As we shall see in the next chapter,this is tantamount to saying that the facts in question have explanations.If a fact has an explanation according to one law,we do not need another,independent law to explain the same fact.Let it be the case that certain objects,a,b,c,and dare all G.Suppose that they are also all F,and also the only Fs that there are.We might think then that it is a law that Fs are Gs and that this law explains why each of the objects is G.But we could do without this law and the explanations it furnishes if it is better established that there is a law that Hs are Gs and a is H,and that there is a law that Js are Gs and that b is J,and that there is a law that Ks are Gs and that c is K,and so on.In this case the proposed law that Fs are Gs is redundant.We can instead regard the fact that all Fs are Gs as an accidental regularity and not a law-like one.If we symbolize "a is F"by "Fa",then as Figure 1.1 shows,we could organize the facts into an economical system with three rather than four laws. Ga Gb Gc Accidental regularity Fa Fb Fc that all Fs are Gs Ha Jb Ke Gm Gp Gr Hm Jp Kr Gn Gq Gs Hn Jq Ks Law that Ks are Gs Law that Js are Gs Law that Hs are Gs Figure 1.1
consist of a shorter list of interlocking regularities, which together are enough to encapsulate all the facts. It would be as simple as possible, but also as strong as possible, capturing as many facts and possible facts as it can. What I mean here by “capturing” a fact is the subsuming of that fact under some law. We want it to be that laws capture all and only their instances. So if the fact is that some object a has the property G, then this would be captured by a law of the form all Fs are Gs (where a is F). For example, if the fact we are interested in is the explosive behaviour of a piece of lithium, then this fact can be captured by the law that lithium reacts explosively on contact with water (if in this case it was in contact with water). If the fact is that some magnitude M has the value m then the fact is captured by a law of the form M=f(X, Y, Z,…) (where the value of X is x, the value of Y is y, etc., and m=f(x, y, z,…). An example of this might be the fact that the current through a circuit is 0.2 A. This fact can be captured by Ohm’s law V=IR, if for instance there is a potential difference of 10 V applied to a circuit that has a resistance of 50 Ω. As we shall see in the next chapter, this is tantamount to saying that the facts in question have explanations. If a fact has an explanation according to one law, we do not need another, independent law to explain the same fact. Let it be the case that certain objects, a, b, c, and d are all G. Suppose that they are also all F, and also the only Fs that there are. We might think then that it is a law that Fs are Gs and that this law explains why each of the objects is G. But we could do without this law and the explanations it furnishes if it is better established that there is a law that Hs are Gs and a is H, and that there is a law that Js are Gs and that b is J, and that there is a law that Ks are Gs and that c is K, and so on. In this case the proposed law that Fs are Gs is redundant. We can instead regard the fact that all Fs are Gs as an accidental regularity and not a law-like one. If we symbolize “a is F” by “Fa”, then as Figure 1.1 shows, we could organize the facts into an economical system with three rather than four laws. Figure 1.1 Laws of nature 25

Philosophy of science 26 This organizing of facts into an economical system allows us to distinguish between accidental regularities and laws.This was one of the problems facing the SRT.The systematic approach does better.It also easily accommodates the other problem case. functional laws.The simplest way of systematizing a collection of distinct points on a graph is to draw the simplest line through them.And we will only want one line,as adding a second line through the same points will make the system much less simple without adding to the power of the system,because the first line already captures the facts we are interested in.Thus in a systematic version of minimalism we will have non-gappy functional laws that are unique in their domain. These are the virtues of the account of laws first given by Frank Ramsey and later developed by David Lewis.The idea is that we regard the system as axiomatized,that is to say we boil the system down to the most general principles from which the regularities that are laws follow.A collection of facts may be axiomatized in many (an infinite number of)ways.So the appropriate axiomatization will be that which,as discussed above,is as simple as possible and as strong as possible.The strength of a proposition can be regarded as its informativeness.So,considering (a)all emeralds are green,(b)all emeralds are coloured,and (c)all South American emeralds are green,(a)is more informative and so stronger than both (b)and(c). Formally,the systematic account says: A regularity is a law of nature if and only if it appears as a theorem or axiom in that true deductive system which achieves a best combination of simplicity and strength. Remember that this is a "God's eye"view,not ours,in the sense that we do not know all the facts that there are and so do not know in that direct way what the best axiomatization of them is.What we are saying is that this is what a law of nature is.Our ignorance of the best axiomatization of everything is identical to our ignorance of the laws of nature.Of course we are not entirely ignorant of them.If science is any good we know some of them,or approximations to them at any rate.And it can be said in support of Ramsey and Lewis,that the sorts of thing we look for in a theory which proposes a law of nature are precisely what they say something ought to have in order to be a law.First we look to see whether it is supported by evidence in the form of instances,i.e.whether it truly is a regularity.But we will also ask whether it is simple,powerful,and integrates with the other laws we believe to exist. The systematic view is not entirely without its problems.First,the notion of simplicity is important to the systematic characterization of law,yet simplicity is a notoriously difficult notion to pin down.In particular,one might think that simplicity is a concept which has a significant subjective component to it.What may appear simple to one person might look rather complex to another.In which case the concept of law would also have a subjective element that conflicts with our intuition that laws are objective and independent of our perspective.Another way of looking at the problem of simplicity is to return to Goodman's puzzle about the concept "grue".The fact that we can replace the simple looking "X is grue"by the complex looking "either X is green and observed before midnight on 31 December 2000 or X is blue and not observed before midnight on 31 December 2000",and vice versa,shows that we cannot be sure of depending merely
This organizing of facts into an economical system allows us to distinguish between accidental regularities and laws. This was one of the problems facing the SRT. The systematic approach does better. It also easily accommodates the other problem case, functional laws. The simplest way of systematizing a collection of distinct points on a graph is to draw the simplest line through them. And we will only want one line, as adding a second line through the same points will make the system much less simple without adding to the power of the system, because the first line already captures the facts we are interested in. Thus in a systematic version of minimalism we will have non-gappy functional laws that are unique in their domain. These are the virtues of the account of laws first given by Frank Ramsey and later developed by David Lewis.12 The idea is that we regard the system as axiomatized, that is to say we boil the system down to the most general principles from which the regularities that are laws follow. A collection of facts may be axiomatized in many (an infinite number of) ways. So the appropriate axiomatization will be that which, as discussed above, is as simple as possible and as strong as possible. The strength of a proposition can be regarded as its informativeness. So, considering (a) all emeralds are green, (b) all emeralds are coloured, and (c) all South American emeralds are green, (a) is more informative and so stronger than both (b) and (c). Formally, the systematic account says: A regularity is a law of nature if and only if it appears as a theorem or axiom in that true deductive system which achieves a best combination of simplicity and strength. Remember that this is a “God’s eye” view, not ours, in the sense that we do not know all the facts that there are and so do not know in that direct way what the best axiomatization of them is. What we are saying is that this is what a law of nature is. Our ignorance of the best axiomatization of everything is identical to our ignorance of the laws of nature. Of course we are not entirely ignorant of them. If science is any good we know some of them, or approximations to them at any rate. And it can be said in support of Ramsey and Lewis, that the sorts of thing we look for in a theory which proposes a law of nature are precisely what they say something ought to have in order to be a law. First we look to see whether it is supported by evidence in the form of instances, i.e. whether it truly is a regularity. But we will also ask whether it is simple, powerful, and integrates with the other laws we believe to exist. The systematic view is not entirely without its problems. First, the notion of simplicity is important to the systematic characterization of law, yet simplicity is a notoriously difficult notion to pin down. In particular, one might think that simplicity is a concept which has a significant subjective component to it. What may appear simple to one person might look rather complex to another. In which case the concept of law would also have a subjective element that conflicts with our intuition that laws are objective and independent of our perspective. Another way of looking at the problem of simplicity is to return to Goodman’s puzzle about the concept “grue”. The fact that we can replace the simple looking “X is grue” by the complex looking “either X is green and observed before midnight on 31 December 2000 or X is blue and not observed before midnight on 31 December 2000”, and vice versa, shows that we cannot be sure of depending merely Philosophy of science 26

Laws of nature 27 on linguistic appearance to tell us the difference.The simple law that emeralds are green appears to be the same as the complex law that emeralds are grue,if observed before midnight on 31 December 2000,or bleen if not observed before midnight on 31 December 2000.Without something like a solution to Goodman's problem we have no reason to prefer one set of concepts to another when trying to pin down the idea of simplicity. The second problem with the systematic view is that as I have presented it,i.e.it presumes there is precisely one system that optimally combines strength and simplicity. For a start it is not laid down how we are supposed to weigh simplicity and strength against one another.We could add more laws to capture more potential facts and thus gain strength,but with a loss in simplicity.Alternatively,we might favour a simpler system that has less strength.Again there is the suspicion that the minimalist's answer to this problem may be objectionably subjective.Even if there is a clear and objective way of balancing these two,it may yet turn out that two or more distinct systems do equally well.So which are our laws?Perhaps something is a law if it appears in any one of the optimal systems.But this will not do,because the different systems might have conflicting laws that lead to incompatible counterfactuals.On the other hand,we may be more restrictive and accept as laws only those regularities that appear in all the optimal systems.3 However,we may find that there are very few such common regularities,and perhaps none at all. Basic laws and derived laws It is a law-like and true (almost)generalization that all objects of 10 kg when subjected to a force of 40 N accelerate at 4 m s2.But this generalization would not feature as an axiom of our system,because it would be more efficient to have the generalization that the acceleration of a body is equal to the ratio of the resultant force acting upon it and its mass.By having that generalization we can dispense with the many generalizations that mention specific masses,forces,and accelerations.From the overarching generalization these more specific ones may be derived.That is to say,our system will be axiomatic;it will comprise the smallest set of laws from which it is possible to deduce all other laws. This shows an increase in simplicity-few generalizations instead of many-and in strength,as the overarching generalization will have consequences not entailed by the collection of specific generalizations.In the example there may be some values of the masses,forces,and accelerations for which there are no specific generalizations,just because nothing ever both had that mass and was subject to that force. This illustrates a distinction between more fundamental laws and those derived from them.Most basic of all are those laws that form the axioms of the optimal system.If the laws of all the sciences can be reduced to laws of physics,as some argue is true,at least for chemistry,then the basic laws will be the fundamental laws of physics.If,on the other hand,there is some field the laws of which are not reducible,then the basic laws will include laws of that field.It might be that we do not yet actually know any basic laws-all the laws we are acquainted with would then be derived laws
on linguistic appearance to tell us the difference. The simple law that emeralds are green appears to be the same as the complex law that emeralds are grue, if observed before midnight on 31 December 2000, or bleen if not observed before midnight on 31 December 2000. Without something like a solution to Goodman’s problem we have no reason to prefer one set of concepts to another when trying to pin down the idea of simplicity. The second problem with the systematic view is that as I have presented it, i.e. it presumes there is precisely one system that optimally combines strength and simplicity. For a start it is not laid down how we are supposed to weigh simplicity and strength against one another. We could add more laws to capture more potential facts and thus gain strength, but with a loss in simplicity. Alternatively, we might favour a simpler system that has less strength. Again there is the suspicion that the minimalist’s answer to this problem may be objectionably subjective. Even if there is a clear and objective way of balancing these two, it may yet turn out that two or more distinct systems do equally well. So which are our laws? Perhaps something is a law if it appears in any one of the optimal systems. But this will not do, because the different systems might have conflicting laws that lead to incompatible counterfactuals. On the other hand, we may be more restrictive and accept as laws only those regularities that appear in all the optimal systems.13 However, we may find that there are very few such common regularities, and perhaps none at all. Basic laws and derived laws It is a law-like and true (almost) generalization that all objects of 10 kg when subjected to a force of 40 N accelerate at 4 m s−2 . But this generalization would not feature as an axiom of our system, because it would be more efficient to have the generalization that the acceleration of a body is equal to the ratio of the resultant force acting upon it and its mass. By having that generalization we can dispense with the many generalizations that mention specific masses, forces, and accelerations. From the overarching generalization these more specific ones may be derived. That is to say, our system will be axiomatic; it will comprise the smallest set of laws from which it is possible to deduce all other laws. This shows an increase in simplicity—few generalizations instead of many—and in strength, as the overarching generalization will have consequences not entailed by the collection of specific generalizations. In the example there may be some values of the masses, forces, and accelerations for which there are no specific generalizations, just because nothing ever both had that mass and was subject to that force. This illustrates a distinction between more fundamental laws and those derived from them. Most basic of all are those laws that form the axioms of the optimal system. If the laws of all the sciences can be reduced to laws of physics, as some argue is true, at least for chemistry, then the basic laws will be the fundamental laws of physics. If, on the other hand, there is some field the laws of which are not reducible, then the basic laws will include laws of that field. It might be that we do not yet actually know any basic laws—all the laws we are acquainted with would then be derived laws. Laws of nature 27

Philosophy of science 28 Laws and accidents I hope that it should now appear that the systematic view of laws is a considerable improvement on the SRT.While it still has problems,the account looks very much as if it is materially adequate.An analysis of a concept is materially adeguate if it is true that were anything to be a case of the concept(the analysandum-the thing to be analysed)it would also be a case of the analysis,and vice versa.So "female fox"is a materially adequate analysis of "vixen"only when it is true that if something were a female fox it would also be a vixen,and if something were a vixen it would also be a female fox.The systematic account looks to be materially adequate because the regularities that are part of or consequences of the optimal system are precisely the regularities we would identify as nomic regularities (regularities of natural law). In this section I want to argue that this is an illusion,that it is perfectly possible that systematic regularities fail to correspond to the laws there are.And in the next section we will see that even if,as a matter of fact,laws and systematic regularities coincide,this is not because they are the same things.The point will be that there are certain things we use laws for,in particular for explaining,for which we cannot use regularities,however systematic.And towards the end of this chapter I shall explain what sort of connection there is between laws and systematic regularities,and why therefore the systematic account looks as if it is materially adequate. Earlier on we looked at the argument that probabilistic laws were a counter-example to minimalism.The idea was that there could be a divergence between the law and the corresponding regularity.Although the argument is not valid,I suggested that our intuitions should be against the minimalist.More generally,I believe that our intuitions suggest that there could be a divergence between the laws there are and the (systematic) regularities there are. Returning to the simple regularity theory for a moment,one reason why this theory could not be right is that there could be a simple regularity that is merely accidental.The fact that there is regularity is a coincidence and nothing more.These instances are not tied together by a law that explains them all.To some extent this can be catered for by the systematic account.For,if the regularity is a coincidence,then we might expect to find that the events making up this regularity each have alternative explanations in terms of a variety of other laws (i.e.each one falls under some other regularity).If this is the case, then the systematic account would not count this regularity as a law.For it would add little in the way of strength,since the events that fall under it also fall under other systematic regularities,while adding it would detract from the simplicity of the overall system. This raises a difficult issue for the systematic theorist,and indeed for minimalists more generally.Might not this way of excluding coincidental regularities get it wrong and exclude genuine laws while including accidents?It might be that there is a large number of laws each of which has only a small number of instances.These laws may throw up some extraordinary coincidences covering a large number of events.By the systematic account the coincidence would be counted as a law because it contributes significantly to the strength of the system,while at the same time the real laws are excluded because their work in systematizing the facts is made redundant by the accidental regularities.Figure 1.2 shows a world similar to the one discussed
Laws and accidents I hope that it should now appear that the systematic view of laws is a considerable improvement on the SRT. While it still has problems, the account looks very much as if it is materially adequate. An analysis of a concept is materially adequate if it is true that were anything to be a case of the concept (the analysandum—the thing to be analysed) it would also be a case of the analysis, and vice versa. So “female fox” is a materially adequate analysis of “vixen” only when it is true that if something were a female fox it would also be a vixen, and if something were a vixen it would also be a female fox. The systematic account looks to be materially adequate because the regularities that are part of or consequences of the optimal system are precisely the regularities we would identify as nomic regularities (regularities of natural law). In this section I want to argue that this is an illusion, that it is perfectly possible that systematic regularities fail to correspond to the laws there are. And in the next section we will see that even if, as a matter of fact, laws and systematic regularities coincide, this is not because they are the same things. The point will be that there are certain things we use laws for, in particular for explaining, for which we cannot use regularities, however systematic. And towards the end of this chapter I shall explain what sort of connection there is between laws and systematic regularities, and why therefore the systematic account looks as if it is materially adequate. Earlier on we looked at the argument that probabilistic laws were a counter-example to minimalism. The idea was that there could be a divergence between the law and the corresponding regularity. Although the argument is not valid, I suggested that our intuitions should be against the minimalist. More generally, I believe that our intuitions suggest that there could be a divergence between the laws there are and the (systematic) regularities there are. Returning to the simple regularity theory for a moment, one reason why this theory could not be right is that there could be a simple regularity that is merely accidental. The fact that there is regularity is a coincidence and nothing more. These instances are not tied together by a law that explains them all. To some extent this can be catered for by the systematic account. For, if the regularity is a coincidence, then we might expect to find that the events making up this regularity each have alternative explanations in terms of a variety of other laws (i.e. each one falls under some other regularity). If this is the case, then the systematic account would not count this regularity as a law. For it would add little in the way of strength, since the events that fall under it also fall under other systematic regularities, while adding it would detract from the simplicity of the overall system. This raises a difficult issue for the systematic theorist, and indeed for minimalists more generally. Might not this way of excluding coincidental regularities get it wrong and exclude genuine laws while including accidents? It might be that there is a large number of laws each of which has only a small number of instances. These laws may throw up some extraordinary coincidences covering a large number of events. By the systematic account the coincidence would be counted as a law because it contributes significantly to the strength of the system, while at the same time the real laws are excluded because their work in systematizing the facts is made redundant by the accidental regularities. Figure 1.2 shows a world similar to the one discussed Philosophy of science 28