
194 CHAPTER 9.SISO DESIGN FOR UNSTABLE SAMPLED-DATA SYSTEMS underdetermined and B,...,3can be obtained as the minimum norm solution. Note that for M=2 one should select w >2 in order to avoid the trivial solution f()=1. The case 6=1,1 was examined in detail in Sec.8.2.Let us now examine the common case where $1,but mi=1 for i=2,...,6.Then (9.3-1) is equivalent to: dk 1-je川=0,k=0,m1-1 (9.3-5a) f元:=1,i=2,,ξ (9.3-5b) The following theorem hoids: Theorem9.3-1.For:=1.S≥2,m:=1fori=2.,ξ,the coefficients 80.....3w must satisfy 9=-8-..-3w 9.3-6) )-1 ξ-1 {2)-1 八 -Q/(1-a) ≥Y 9.3-7) 0 n:-1 0 where 。。 = 9.3-8 and the elements vij of the m.-x w matriz Nw are defined by (8.2-6) 0 for i>j fori≤j (8.2-6) Proof.Follows directlyfrom T5.2-1,(9.3-5)and the fact that f(1)=1. Forξ=1,Thm.9.3-1 reduces :o Thm.8.2-1.Form:=1.the choice w=ξ-l. reduces (9.3-7)to the Vandende form (5.3-4).In general one should select w1=+m-2 and obian 3:.....as the minimum norm solution to (9.3-7小

9.3.THE DISCRETE IMC FILTER 195 =AT(AAT)-x (9.3-9) where 4(风) (9.3-10) Note that from a numerical point of view it is preferable to compute the pseudo- inverse in (9.3-9)from a singular value decomposition of N 9.3.2 Qualitative Interpretation of the Filter Function The discussion in this section is in the spirit of that in Sec.5.3.2 for continuous systems.With the filter f(=)and the IMC controller (=obtained in the first design step,the discrete coraplementary sensitivity function becomes for p"=p': ()=p'():)f) (9.3-11) For open-loop stable systeras.any stabie filter that satisfies the system type requirements described by Thm.7.5-1 is acceptable.For open-loop unstable sys- tems however,the filter has to be unity at the unstable system poles,which limits the range of filter parameters a that can be chosen for reasonable performance,as we shall show uext.For the effect of the unstable poles on n'to become negligible f(=)has to approach f(=):S.2-1).Specifically,it follows from (9.3-3)that (= has to approach unity.We will study the behavior of f)for uoo. Consider the system studied in Sec.9.2.4.iv.For internal stability and asymp- totically error-free disturbarce compensation we require f(1)=f(eor)=1 (9.3-12) In the notation of Sec.9.3.1.we have in this case =2.=1,2=eT.m= m2 =1.Hence f(z)is given by (9.3-3.4.6.9)where A={e-6r-1.eur-1) (9.3-13) t=fie7)1-1=a1-e如 1-a 9.3-14) From (9.3-9)it follows that 宫--源 (9.3-15)
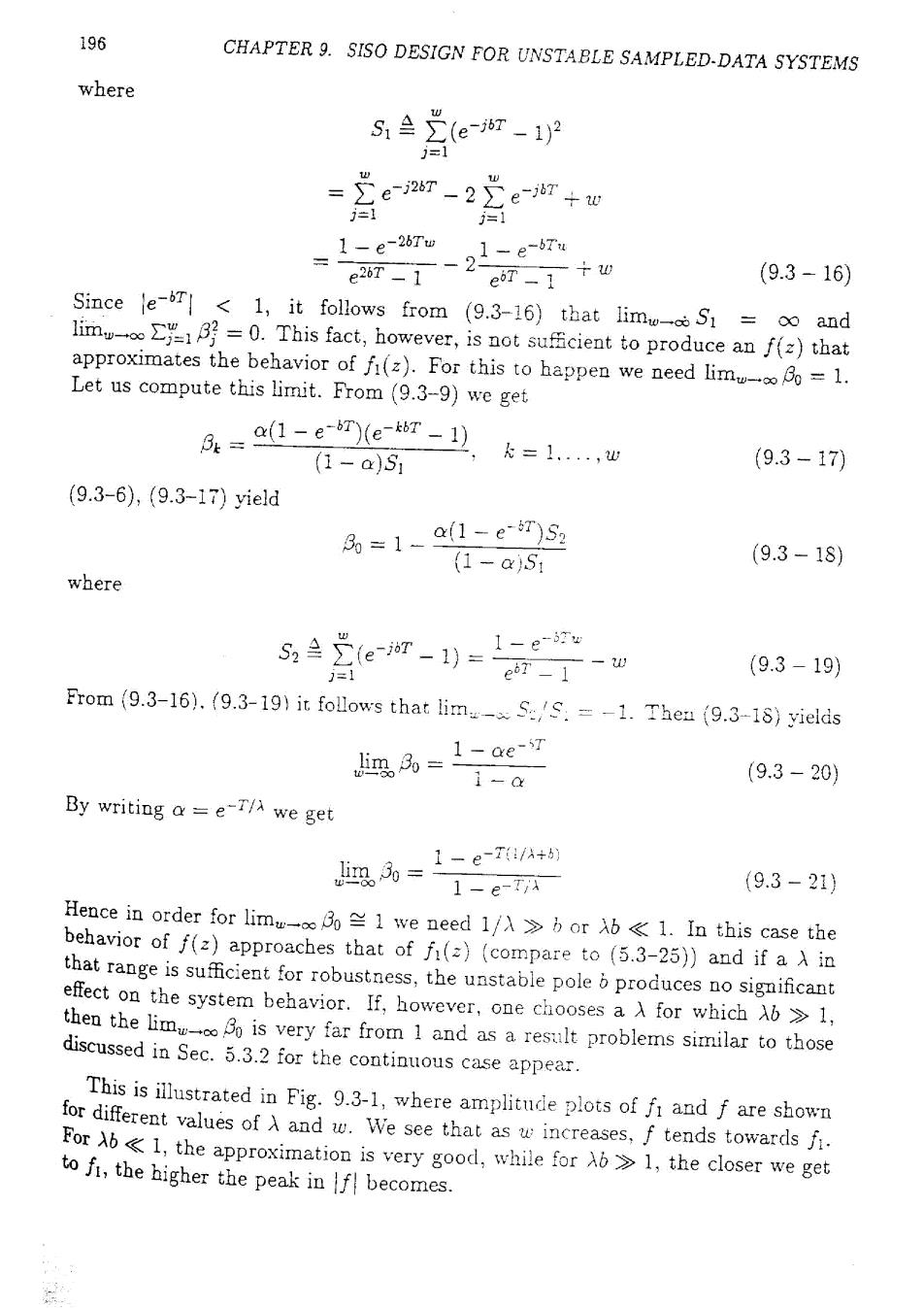
196 CHAPTER 9.SISO DESIGN FOR UNSTABLE SAMPLED-DATA SYSTEMS where 5∑(e7-1)2 J=i se-2&好-2e-6T+w 1=1 1-e-267w 1-e-Tu (9.3-16) Since e-T<1,it follows from (9.3-16)that limw-S oo and lim=0.This fact,however,is not sufficient to produce an f()that approximates the behavior of fi(z).For this to happen we need limo=1. Let us compute this limit.From(9.3-9)we get .=a1-e(et7-1 (1-a)S1 ,k=1w (9.3-17) (9.3-6),(9.3-17)yield 8=1- a(1-e-T)52 (1-a)S1 (9.3-18) where S2兰(eir-1)=e、m =1 (9.3-19) From (9.3-16).(9.3-19)it follows that limS./S.=-1.Then (9.3-18)yields 玛= 1-ae-ST 1- (9.3-20) By writing a=e-T/A we get 照=。源 1-e-T/A+ (9.3-21) Hence in order for lim we need 1/o1.In this case the behavior of f(z)approaches that of fi(=)(compare to (5.3-25))and if a in that range is sufficient for robustness,the unstable pole b produces no significant effect on the system behavior.If,however,one chooses a for which b1, then the li is very far from 1 and as a result problems similar to those discussed in Sec.5.3.2 for the continuous case appear. This is illustrated in Fig.9.3-1,where amplitude plots of f and f are shown for different values of and w.We see that as w increases,f tends towardsfi. For,the approximation is very good,while for1,the closer we get to fi,the higher the peak infbecomes

9.3.THE DISCRETE IMC FILTER 197 102 1 2 入▣0.1 201 102 103 1. 201 02 A 01 1. 入=10 102 102 10-1 1. 01 1c2 日 Figure 9.3-1.Effect of a RHP pole on the discrete IMC filter.T =0.1.6 =1.Solid:f1,Dash: ow=9,Dot-Dash:f1o,w=2

198 CHAPTER 9.SISO DESIGN FOR UNSTABLE SAMPLED-DATA SYSTEAS 9.4 Robust Stability For controllers designed via the IMC design procedure (=paf)Thm.7.6-1 becomes Cor.9.4-1. Corollary 9.4-1 (Robust Stability).Assume that all plants p in the family n ={p: p(i) (9-1) have the same number of RHP poles and that these poles do not become unob- servable after sampliig.Then the system is robusily stable if and only if the IAIC filter satisfies 胡< 0≤w≤π/T (9.-2 where a is a stabilizing controller for the nominal plant p. For stable systems f is arbitrary.Therefore.there always exists a flter f which satisfes (9.4-2:regardless of the magnitude of the uncertainty For unstable systems f is constrained to be unity at the poles of p"outside the ['C. Thus,depending onthere might not exist any filter parameter for which the constraint (9.4-2:is rnet.Indeed,there might not exist any filter--however complicated which satisfies (9.4-2).A minitnum amount of information is necessary or equivaleutly a maximum amount of uncertainty is allowed to stabilize an unstable systemn.The necessary information at=0 can be characterized easily. Corollary 9.4-2.Assume that a filter f is to be designed for a system or dis- turbance pole(s)at s=0-i.e.,f(1)=1.There exisis an f such thut ihe closed loop system is robusily stable for the jamily II described by (9.4-1)only if im(0)<1. Note that contrary to Cor.8.3-2.Cor.9.4-2 is only necessary.For unstabe systems,in general,the filter has to satisfy other constraints in addition to the one at z=1. 9.5 Robust Performance The results in Sec.7.7.2 hold for unstable systems if it is assumed that all pants in the family II have the same number of RHP poles and if the controller and filter f are stabilizing for the nominal plant p