
Seminar on Advanced Topics in Mathematics Solving Polynomial Equations 31 March 2008 Dr.Tuen Wai Ng,HKU
Seminar on Advanced Topics in Mathematics Solving Polynomial Equations 31 March 2008 Dr. Tuen Wai Ng, HKU

What is a polynomial anxn+·+a1x+a0 This modern notation was more or less developed by Rene Descartes (1596-1650)in his book La Geometrie [Geometry](1637). Before the 17th century,mathematicians usually did not use any particular notation. Before Descartes,Francois Viete (1540-1603)has already developed the basic idea of introducing arbitrary parameters into an equation and to distinguish these from the equation's variables. 1
What is a polynomial ? anx n + · · · + a1x + a0 This modern notation was more or less developed by Ren´e Descartes (1596-1650) in his book La G´eom´etrie [Geometry] (1637). Before the 17th century, mathematicians usually did not use any particular notation. Before Descartes, Fran¸cois Vi`ete (1540-1603) has already developed the basic idea of introducing arbitrary parameters into an equation and to distinguish these from the equation’s variables. 1

Francois Viete (1540-1603) Rene Descartes (1596-1650) He used consonant (B,C,D,....)to denote parameters and vowels (A,E,I,...)to denote variables. Certainly,letters had been used before Viete,but not in actual computations;one letter would be used for a certain quantity,another for its square,and so forth. 2
Fran¸cois Vi`ete (1540-1603) Ren´e Descartes (1596-1650) He used consonant (B, C, D, . . ..) to denote parameters and vowels (A, E, I, . . .) to denote variables. Certainly, letters had been used before Vi`ete, but not in actual computations; one letter would be used for a certain quantity, another for its square, and so forth. 2
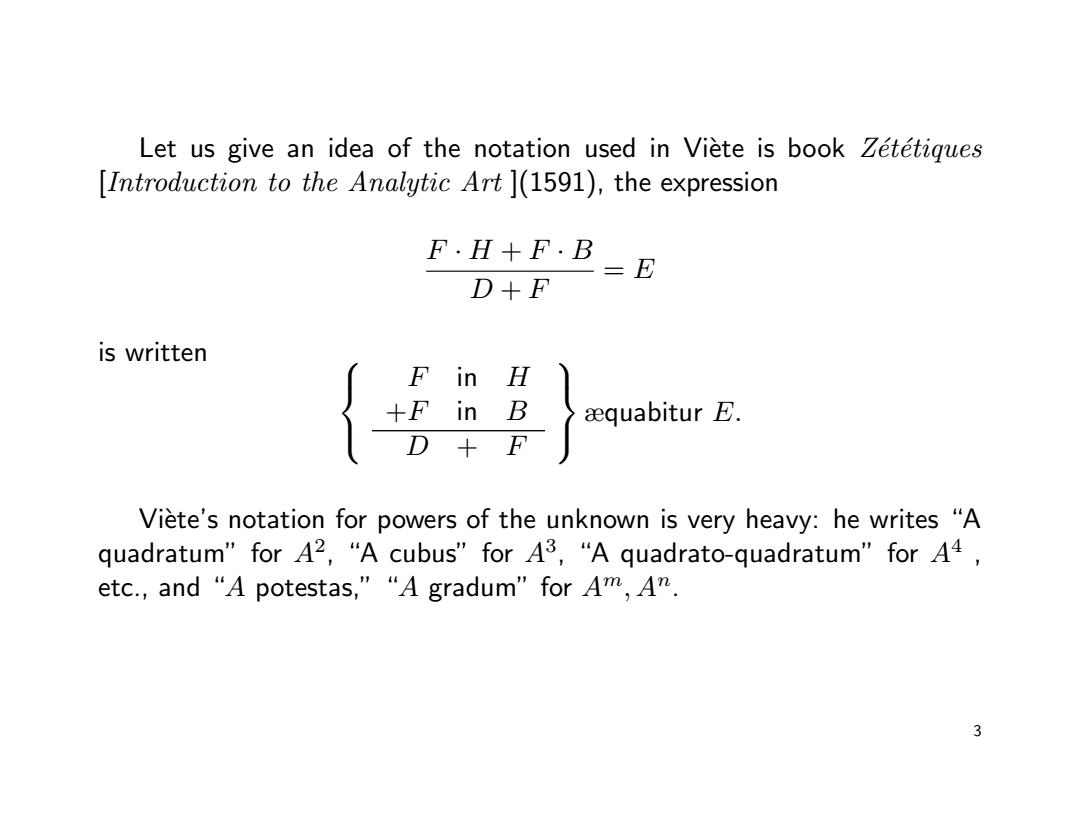
Let us give an idea of the notation used in Viete is book Zetetiques [Introduction to the Analytic Art ](1591),the expression F·H+F·B D+F 二E is written {} aequabitur E. Viete's notation for powers of the unknown is very heavy:he writes "A quadratum”forA2,“A cubus'”forA3,“A quadrato--quadratum”forA4, etc,and“A potestas,.”“A gradum"for Am,A". 3
Let us give an idea of the notation used in Vi`ete is book Z´et´etiques [Introduction to the Analytic Art ](1591), the expression F · H + F · B D + F = E is written F in H +F in B D + F æquabitur E. Vi`ete’s notation for powers of the unknown is very heavy: he writes “A quadratum” for A2 , “A cubus” for A3 , “A quadrato-quadratum” for A4 , etc., and “A potestas,” “A gradum” for Am, An. 3

His notation was only partly symbolic.For example,an equation in the unknown A,such as A3+3B2A =203 would be expressed by Viete as A cubus +B plano 3 in A aequari C solido 2. Note that Viete required "homogeneity"in algebraic expressions:all terms had to be of same degree (for example,all terms above are of the third degree). The requirement of homogeneity goes back to Greek antiquity,where geometry reigned supreme.To the Greek way of thinking,the product ab (say)denoted the area of a rectangle with sides a and b;similarly,abc denoted the volume of a cube.An expression such as ab+c had no meaning since one could not add length to area. This condition of homogeneity was definitively abandoned only around the time of Descartes. 4
His notation was only partly symbolic. For example, an equation in the unknown A, such as A3 + 3B2A = 2C 3 would be expressed by Vi`ete as A cubus +B plano 3 in A aequari C solido 2. Note that Vi`ete required “homogeneity” in algebraic expressions: all terms had to be of same degree (for example, all terms above are of the third degree). The requirement of homogeneity goes back to Greek antiquity, where geometry reigned supreme. To the Greek way of thinking, the product ab (say) denoted the area of a rectangle with sides a and b; similarly, abc denoted the volume of a cube. An expression such as ab+c had no meaning since one could not add length to area. This condition of homogeneity was definitively abandoned only around the time of Descartes. 4