
Seminar on Advanced Topics in Mathematics Solving Polynomial Equations 5 December 2006 Dr.Tuen Wai Ng,HKU
Seminar on Advanced Topics in Mathematics Solving Polynomial Equations 5 December 2006 Dr. Tuen Wai Ng, HKU

What do we mean by solving an equation Example 1.Solve the equation z2 -1. x2=1 x2-1=0 (x-1)(x+1) =0 x=10r=-1 Need to check that in fact (1)2=1 and (-1)2=1. Exercise.Solve the equation Vx+Vx-a=2 where a is a positive real number
What do we mean by solving an equation ? Example 1. Solve the equation x 2 = 1. x 2 = 1 x 2 − 1 = 0 (x − 1)(x + 1) = 0 x = 1 or = −1 • Need to check that in fact (1)2 = 1 and (−1)2 = 1. Exercise. Solve the equation √ x + √ x − a = 2 where a is a positive real number
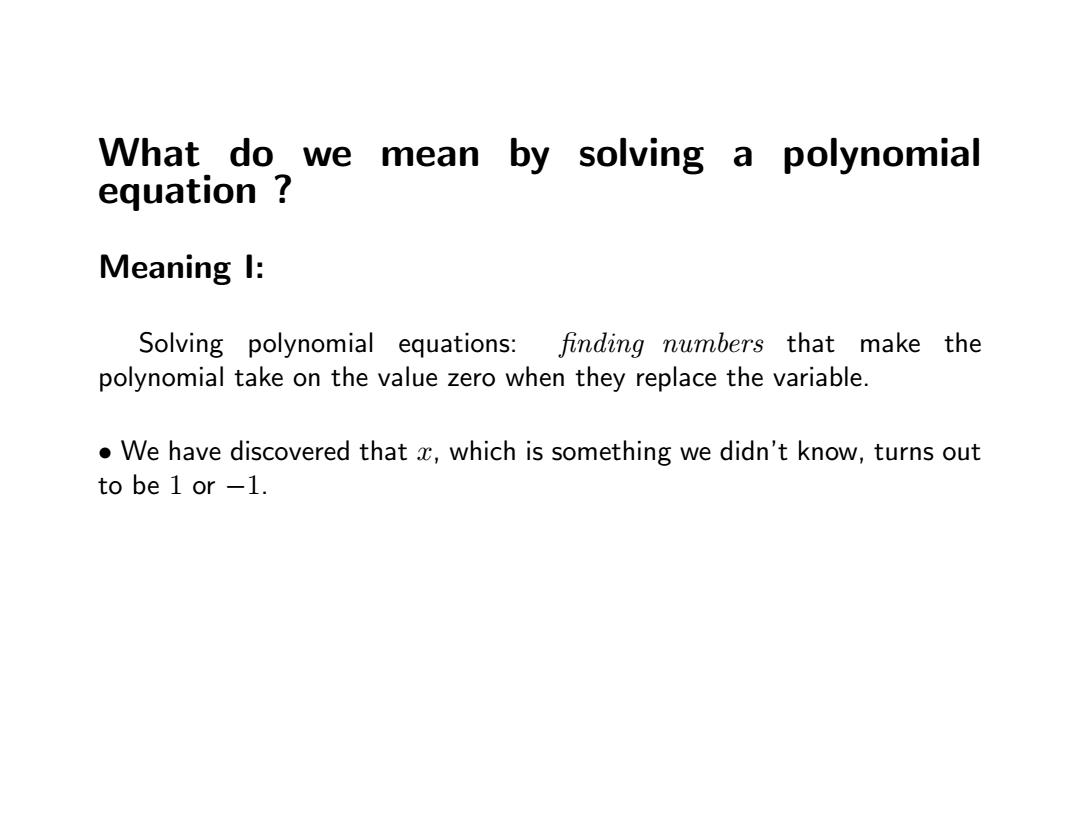
What do we i mean by solving a polynomial equation Meaning I: Solving polynomial equations:finding numbers that make the polynomial take on the value zero when they replace the variable. We have discovered that z,which is something we didn't know,turns out to be 1 or-1
What do we mean by solving a polynomial equation ? Meaning I: Solving polynomial equations: finding numbers that make the polynomial take on the value zero when they replace the variable. • We have discovered that x, which is something we didn’t know, turns out to be 1 or −1
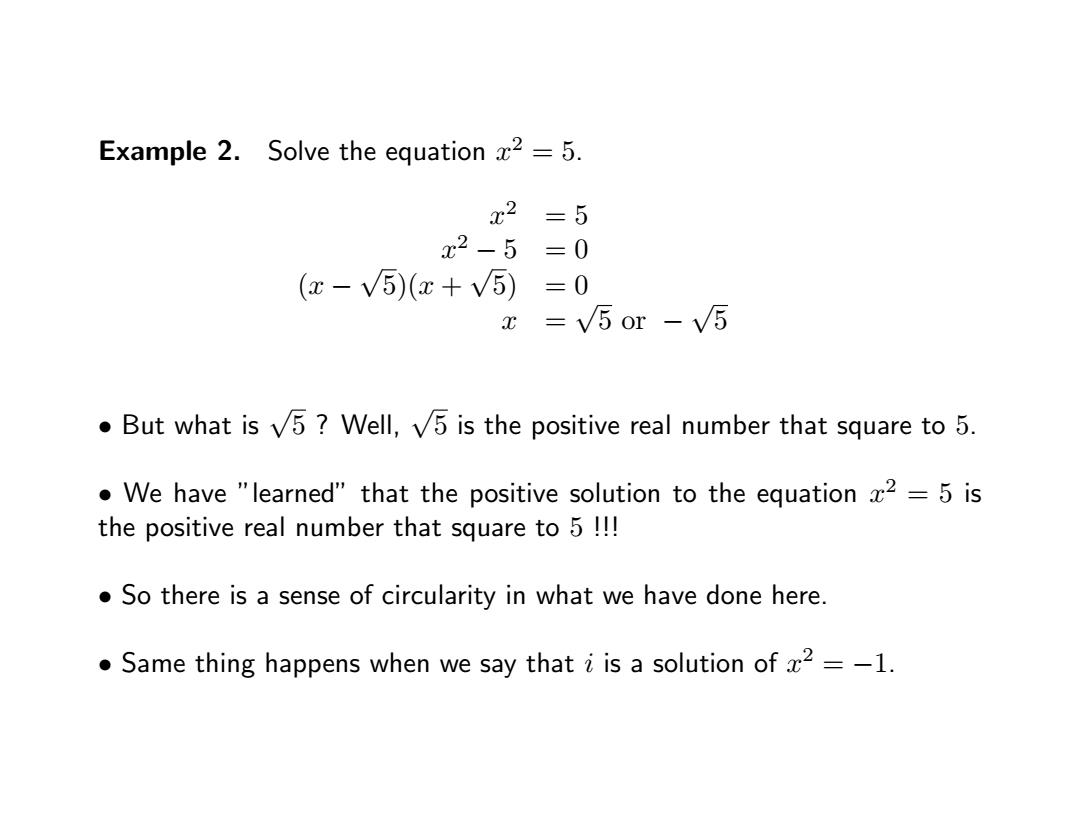
Example 2.Solve the equation z2=5. x2 =5 x2-5 =0 (x-V5)(x+V5) =0 =50r-vV5 C ●But what is√5?Well,V5 is the positive real number that square to5. We have "learned"that the positive solution to the equation z2-5 is the positive real number that square to 5 !! So there is a sense of circularity in what we have done here. Same thing happens when we say that i is a solution of x2=-1
Example 2. Solve the equation x 2 = 5. x 2 = 5 x 2 − 5 = 0 (x − √ 5)(x + √ 5) = 0 x = √ 5 or − √ 5 • But what is √ 5 ? Well, √ 5 is the positive real number that square to 5. • We have ”learned” that the positive solution to the equation x 2 = 5 is the positive real number that square to 5 !!! • So there is a sense of circularity in what we have done here. • Same thing happens when we say that i is a solution of x 2 = −1

What are "solved"when we s solve t these equations The equations z2=5 and x2=-1 draw the attention to an inadequacy in a certain number system (it does not contain a solution to the equation). One is therefore driven to extend the number system by introducing,or adjoining',a solution. Sometimes,the extended system has the good algebraic properties of the original one,e.g.addition and multiplication can be defined in a natural way. .These extended number systems (e.g.Q(v5)or Q(i))have the added advantage that more equations can be solved
What are “solved” when we solve these equations ? • The equations x 2 = 5 and x 2 = −1 draw the attention to an inadequacy in a certain number system (it does not contain a solution to the equation). • One is therefore driven to extend the number system by introducing, or ‘adjoining’, a solution. • Sometimes, the extended system has the good algebraic properties of the original one, e.g. addition and multiplication can be defined in a natural way. • These extended number systems (e.g. Q( √ 5) or Q(i)) have the added advantage that more equations can be solved