
Most of the shortcomings of Viete's notations were overcome by Descartes in his important book La Geometrie [Geometry,in which he expounded the basic elements of analytic geometry. Before Descartes,32 was written as 32 by Ralfaele Bombelli in 1572, whereas Simon Stevin wrote 3+50-40 for 3x3+5x2-4x in 1585. The exponential notation 22,23,etc.,came with Descartes,whose formulas are actually written in a notation very close to our own.For example,he used x,y,2,...for variables and a,b,c,for parameters. Most importantly,he introduced an "algebra of segments."That is,for any two line segments with lengths a and b,he constructed line segments with lengths a +b,a-b,a x b,and a/b. Thus homogeneity of algebraic expressions was no longer needed.For example,ab+c was now a legitimate expression,namely a line segment. 5
Most of the shortcomings of Vi`ete’s notations were overcome by Descartes in his important book La G´eom´etrie [Geometry], in which he expounded the basic elements of analytic geometry. Before Descartes, 3x 2 was written as 3 2 ^ by Ralfaele Bombelli in 1572, whereas Simon Stevin wrote 3°3 + 5°2 − 4°1 for 3x 3 + 5x 2 − 4x in 1585. The exponential notation x 2 , x3 , etc., came with Descartes, whose formulas are actually written in a notation very close to our own. For example, he used x, y, z, . . . for variables and a, b, c, for parameters. Most importantly, he introduced an “algebra of segments.” That is, for any two line segments with lengths a and b, he constructed line segments with lengths a + b, a − b, a × b, and a/b. Thus homogeneity of algebraic expressions was no longer needed. For example, ab + c was now a legitimate expression, namely a line segment. 5

So there is no need of geometry in algebra and algebra had now become considerably more abstract.It was well on its way toto play an important role in mathematics. Viete and Descartes's notations and ideas proved indispensable in the crucial developments of the seventeenth century:in analytic geometry, calculus,and mathematized science. Remarks on other notations. The signs and-were already in use around 1480 (was apparently a deformation of the symbol &)but by the beginning of the 17th century, they were used generally. 6
So there is no need of geometry in algebra and algebra had now become considerably more abstract. It was well on its way to to play an important role in mathematics. Vi`ete and Descartes’s notations and ideas proved indispensable in the crucial developments of the seventeenth century: in analytic geometry, calculus, and mathematized science. Remarks on other notations. The signs + and − were already in use around 1480 (+ was apparently a deformation of the symbol &), but by the beginning of the 17th century, they were used generally. 6

Multiplication was written as M by Michael Stifel (1545),and as in by Viete (1591);our current notation dates back to William Oughtred (1637) for the symbol x,and to Wilhelm Leibniz (1698)for the dot. The symbol used by Michel Recorde (1557)came to replace the symbol used by Descartes,an a written backward,toward the end of the 17th century,thanks to Leibniz. Albert Girard (1595-1632)introduced the notation v3,which he substituted for he also introduced the abbreviations for sine and tangent, and used the symbols <,like Harriot. The symbol was introduced by Leonhard Euler (1707-1783) These notations passed into general usage only during the 19th century. 7
Multiplication was written as M by Michael Stifel (1545), and as in by Vi`ete (1591); our current notation dates back to William Oughtred (1637) for the symbol ×, and to Wilhelm Leibniz (1698) for the dot. The symbol = used by Michel Recorde (1557) came to replace the symbol used by Descartes, an α written backward, toward the end of the 17th century, thanks to Leibniz. Albert Girard (1595-1632) introduced the notation √ 3, which he substituted for 1°3 ; he also introduced the abbreviations for sine and tangent, and used the symbols <, > like Harriot. The symbol P was introduced by Leonhard Euler (1707-1783). These notations passed into general usage only during the 19th century. 7
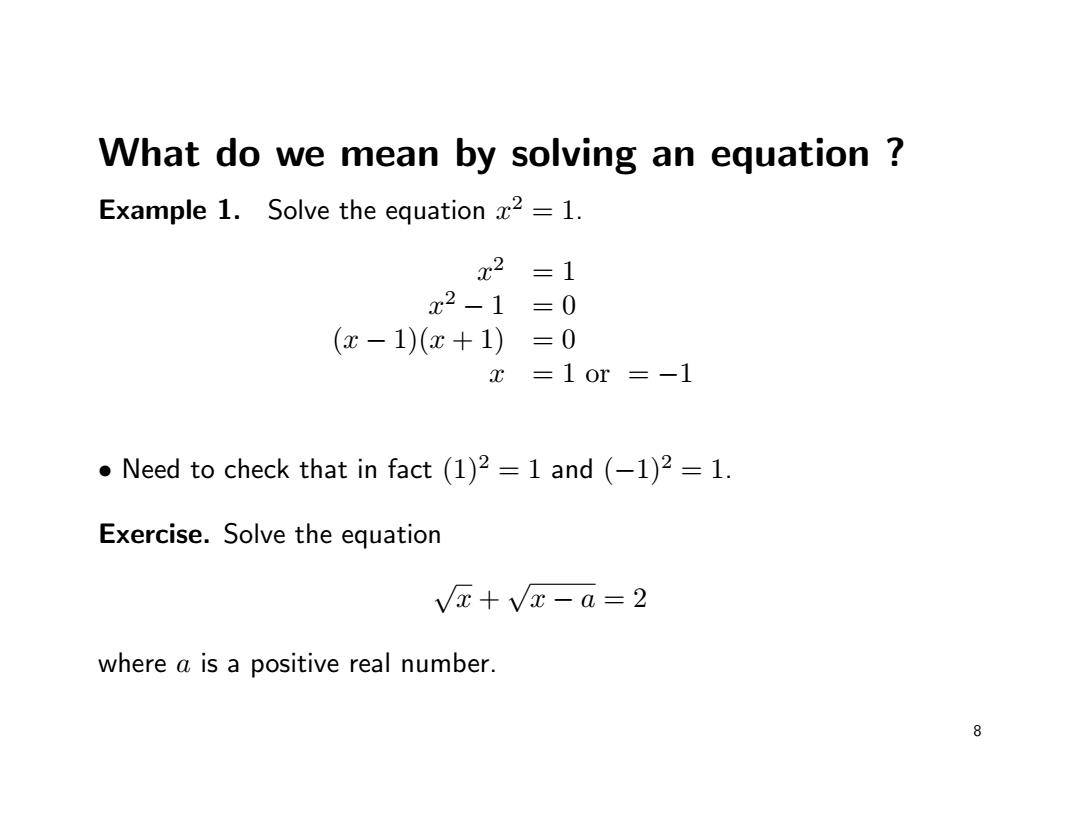
What do we mean by solving an equation Example 1.Solve the equation z2 -1. x2=1 x2-1=0 (x-1)(x+1) =0 x=10r=-1 Need to check that in fact (1)2=1 and (-1)2=1. Exercise.Solve the equation Vx+Vx-a=2 where a is a positive real number. 8
What do we mean by solving an equation ? Example 1. Solve the equation x 2 = 1. x 2 = 1 x 2 − 1 = 0 (x − 1)(x + 1) = 0 x = 1 or = −1 • Need to check that in fact (1)2 = 1 and (−1)2 = 1. Exercise. Solve the equation √ x + √ x − a = 2 where a is a positive real number. 8
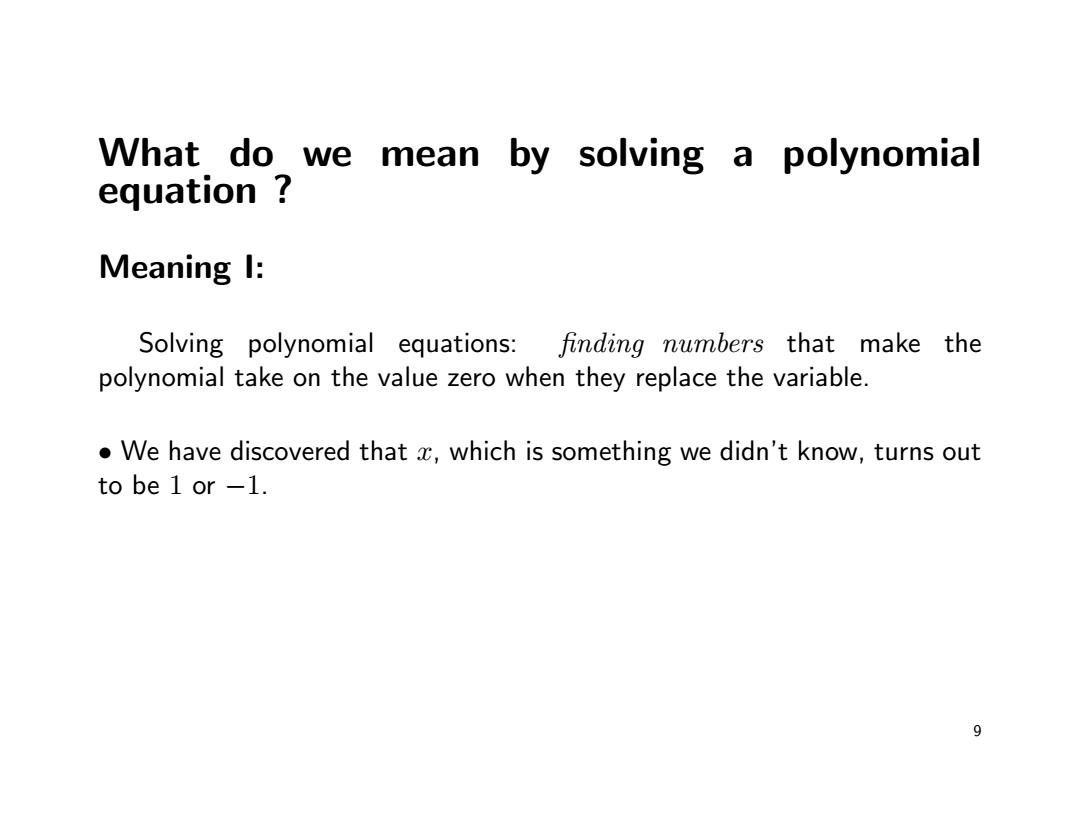
What do we i mean by solving a polynomial equation Meaning I: Solving polynomial equations:finding numbers that make the polynomial take on the value zero when they replace the variable. We have discovered that x,which is something we didn't know,turns out to be 1 or-1. 9
What do we mean by solving a polynomial equation ? Meaning I: Solving polynomial equations: finding numbers that make the polynomial take on the value zero when they replace the variable. • We have discovered that x, which is something we didn’t know, turns out to be 1 or −1. 9