
中图科亨技术大学数学系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS 对应于D=P"(x)=Span{L,x,x2,.x"} 则 = Πs-x≠0 x 0≤j<isn Vandermonde行列式
数 学 系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS ( ) {1, , , } n 2 n 对应于 = x = span x x x 则 0 1 1 0 0 = − jin i j n n n x x x x Vandermonde行列式

中图科亨技术大学数学系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS 多项式插值的Lagrange型 ●如何找? 在基函数上下功夫,取基函数为{L,(x)}”。P” 求)-成0i/则w-空(rx
数 学 系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS 多项式插值的Lagrange型 ⚫ 如何找? 在基函数上下功夫,取基函数为 n n i x i {l ( )} =0 要求 = = = i j i j l i xj i j 1, 0, ( ) 则 ( ) ( ) ( ) 0 i n i i g x l x f x = =
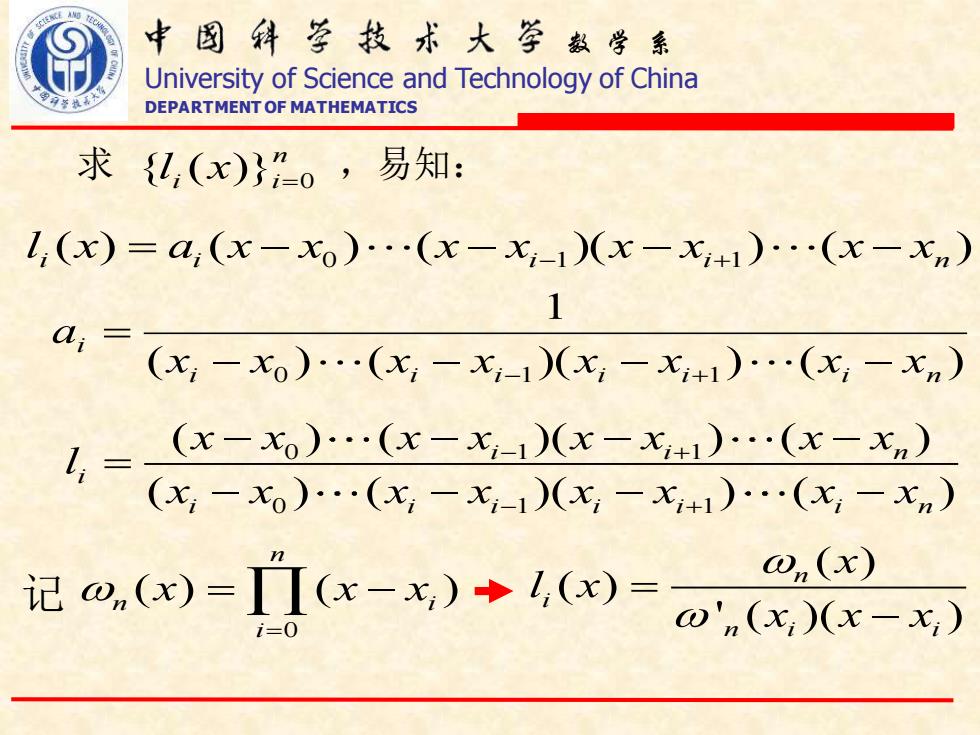
中图科亨技术大学数学系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS 求{L,(x)}”o,易知: L,(x)=a,(x-xo)(x-x,-1)(x-x+1)(x-xn) a, 1 (x,-xo)(x,-x,-1)(x-x+1)(x,-xn) (x-xo).(x-x,-1)(x-x+1)(x-xn) =-).(x-x4x-(x) i记o,(0)-Π(x-)+1,()=D,(xx-x) ⊙n(x)
数 学 系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS 求 n i x i l 0 { ( )} = ,易知: ( ) ( ) ( )( ) ( ) i x ai x x0 x xi 1 x xi 1 x xn l = − − − − + − ( ) ( )( ) ( ) 1 i 0 i i 1 i i 1 i n i x x x x x x x x a − − − − = − + 0 1 1 0 1 1 ( ) ( )( ) ( ) ( ) ( )( ) ( ) i i n i i i i i i i n x x x x x x x x l x x x x x x x x − + − + − − − − = − − − − 0 ( ) ( ) n n i i x x x = 记 = − ( ) ( ) ' ( )( ) n i n i i x l x x x x = −

中图科亨技术大学数学系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS ·线性插值 ()=x- ,1(x)=x- X0-X1 X1-X0 L (x)=f(o)(x)+f(x(x)
数 学 系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS ⚫线性插值 1 0 0 1 0 1 1 0 ( ) , ( ) x x x x l x x x x x l x − − = − − = ( ) ( ) ( ) ( ) ( ) 1 0 0 1 1 L x = f x l x + f x l x

中图科亨技术大学数学系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS ·二次插值 h(x)=x-x-x) 4(x)=x-x-x) (x-x)-x2) (x1-x0)x1-x2) (x)= (x-x)(x-x) x2-)(x2-x) L(x)=f(xo)(x)+f(x(x)+f(x(x)
数 学 系 University of Science and Technology of China DEPARTMENT OF MATHEMATICS ⚫二次插值 1 2 0 0 1 0 2 ( )( ) ( ) ( )( ) x x x x l x x x x x − − = − − 2 0 0 1 1 2 2 L x f x l x f x l x f x l x ( ) ( ) ( ) ( ) ( ) ( ) ( ) = + + 0 2 1 1 0 1 2 ( )( ) ( ) ( )( ) x x x x l x x x x x − − = − − 0 1 2 2 0 2 1 ( )( ) ( ) ( )( ) x x x x l x x x x x − − = − −