上海交通大学：《材料热力学 Thermodynamics of materials》课程教学资源（课件讲稿）lecture 6 property relation（2/2）

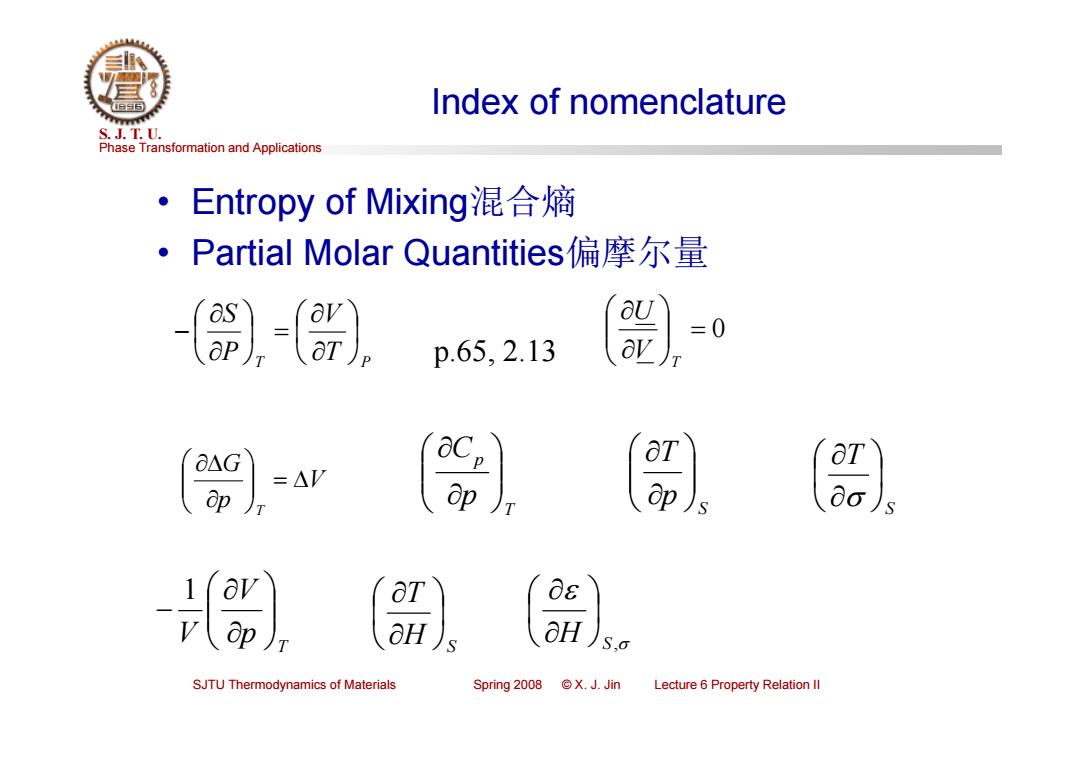
Index of nomenclature S.J.T.0. Phase Transformation and Applications Entropy of Mixing混合熵 ·Partial Molar Quantities偏摩尔量 p.65,2.13 -AV aT ap ap)s ap aH aH S,0 SJTU Thermodynamics of Materials Spring 2008 ©X.J.Jin Lecture 6 Property Relation Il

Phase Transformation and Applications S. J. T. U. SJTU Thermodynamics of Materials Spring 2008 © X. J. Jin Lecture 6 Property Relation II Index of nomenclature • Entropy of Mixing混合熵 • Partial Molar Quantities偏摩尔量 T T P V P S           0    T VU p.65, 2.13 V p G T      T p pC    S pT    S T    T p V V     1 H S T     H S ,   

Partial Molar Quantities S.J.T.0. Phase Transformation and Applications The partial derivative of that quantity with respect to mass(number of moles)at constant temperature and constant pressure,and the mass of all other materials in the system. The partial molar volume of material a in a solution. 7a= av Ona T,P,nb,nc,… Pure a > av The partial molar volume would be equal to the molar volume. Ona ). Constant T and P The chemical potential is the partial molar Gibbs free energy.(Only) na SJTU Thermodynamics of Materials Spring 2008 ©X.J.Jin Lecture 6 Property Relation Il

Phase Transformation and Applications S. J. T. U. SJTU Thermodynamics of Materials Spring 2008 © X. J. Jin Lecture 6 Property Relation II Partial Molar Quantities The partial derivative of that quantity with respect to mass (number of moles) at constant temperature and constant pressure, and the mass of all other materials in the system. a T ,P,nb ,nc , a n V V     The partial molar volume of material a in a solution. a T ,P,nb ,nc , a n V Slope V      na V Constant T and P Pure a The partial molar volume would be equal to the molar volume. The chemical potential is the partial molar Gibbs free energy. (Only)

Property Relations (1) S.J.T.0. Phase Transformation and Applications OM aN dz Mdx Ndy dU=TdS-Pdv dH TaS +Vap as av dF=-SdT-Pdv as p dG=-SdT+Vap as aP av at H as av SJTU Thermodynamics of Materials Spring2008©X.J.Jin Lecture 6 Property Relation Il

Phase Transformation and Applications S. J. T. U. SJTU Thermodynamics of Materials Spring 2008 © X. J. Jin Lecture 6 Property Relation II Property Relations (1) dz  Mdx  Ndy dU  TdS  PdV dH  TdS VdP dF  SdT  PdV dG  SdT VdP x y x N y M        S S V P V T          S S P V P T         T T V P V S         T T P V P S         

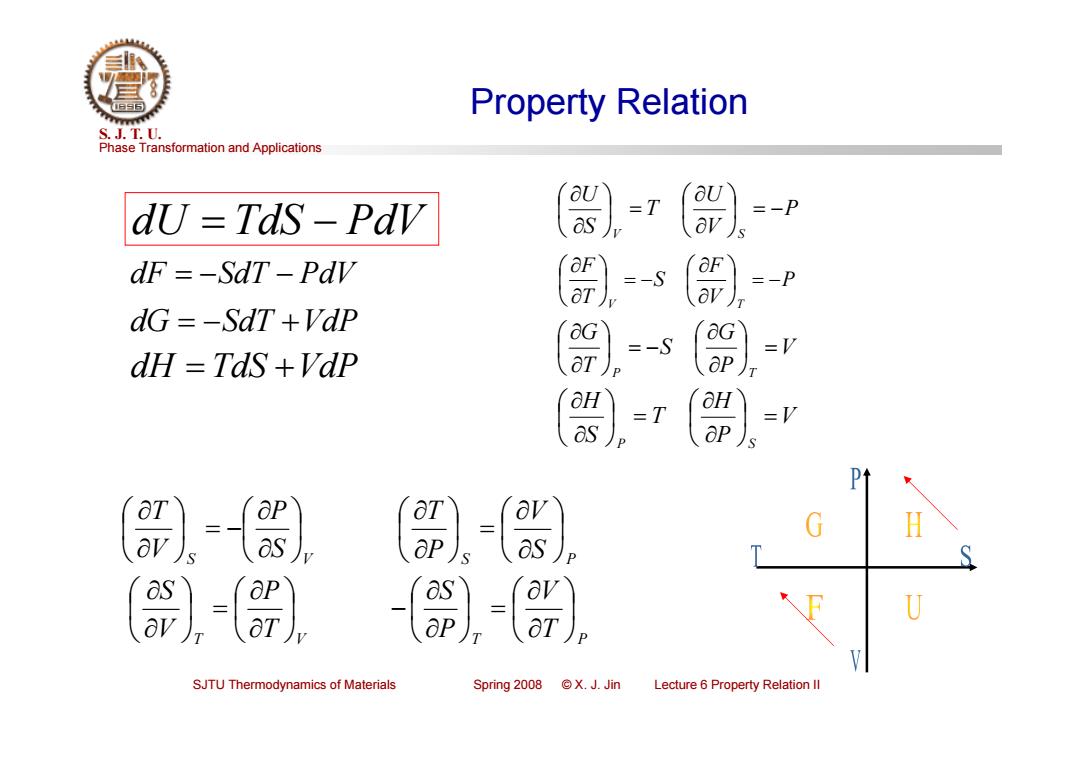
Property Relation S.J.T.0. Phase Transformation and Applications dU=TdS-PdV 〔器) -T =-P dF =-SdT-Pdv 〔) =-S =-P dG =-SdT +Vap =-S =V dH TaS +Vap 〔) -T ap a as aP)s as as U aT) SJTU Thermodynamics of Materials Spring 2008 ©X.J.Jin Lecture 6 Property Relation Il

Phase Transformation and Applications S. J. T. U. SJTU Thermodynamics of Materials Spring 2008 © X. J. Jin Lecture 6 Property Relation II Property Relation S S V P V T          S S P V PT         T T V P V S         T T P V PS          dU  TdS  PdV dF  SdT  PdV dG  SdT VdP dH  TdS VdP P V U T S U V S            P V F S T F V T             V P G S T G P T            V P H T S H P S          

恒温下熵变的计算(1) S.J.T.0. Phase Transformation and Applications p.65,2.13 s:-s.-fds--(r)dp EX:ideal gas PV=RT R-P ds=- 恒温下，当压力改变时，将引起熵变 For a change in pressure from 1 atm to 10 atm at constant temperature -019.4J/(mol-K) U SJTU Thermodynamics of Materials Spring2008©X.J.Jin Lecture 6 Property Relation Il

Phase Transformation and Applications S. J. T. U. SJTU Thermodynamics of Materials Spring 2008 © X. J. Jin Lecture 6 Property Relation II 恒温下熵变的计算(1) T T P V P S                   21 21 2 1 PP P dP TV S S d S EX: ideal gas dP P R d S P R P S P R T V PV RT P T               For a change in pressure from 1 atm to 10 atm at constant temperature R P R J   mol K PdP S RPP            ln ln10 19.14 / 101 21 恒温下，当压力改变时，将引起熵变 p.65, 2.13