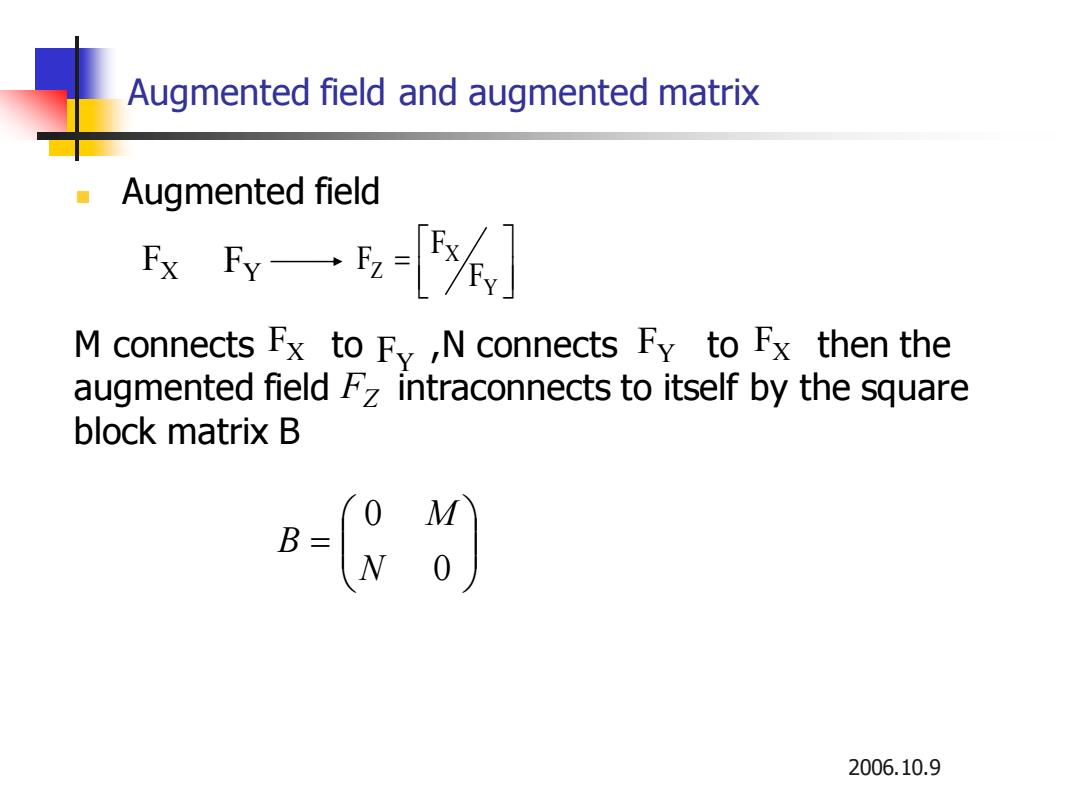
Augmented field and augmented matrix Augmented field Rv一,[K] M connects Fx to F,N connects Fy to Fx then the augmented field Fz intraconnects to itself by the square block matrix B 2006.10.9
2006.10.9 Augmented field and augmented matrix Augmented field M connects to ,N connects to then the augmented field intraconnects to itself by the square block matrix B = Y X Z F F F FX FY FZ FX FY FY FX = 0 0 N M B
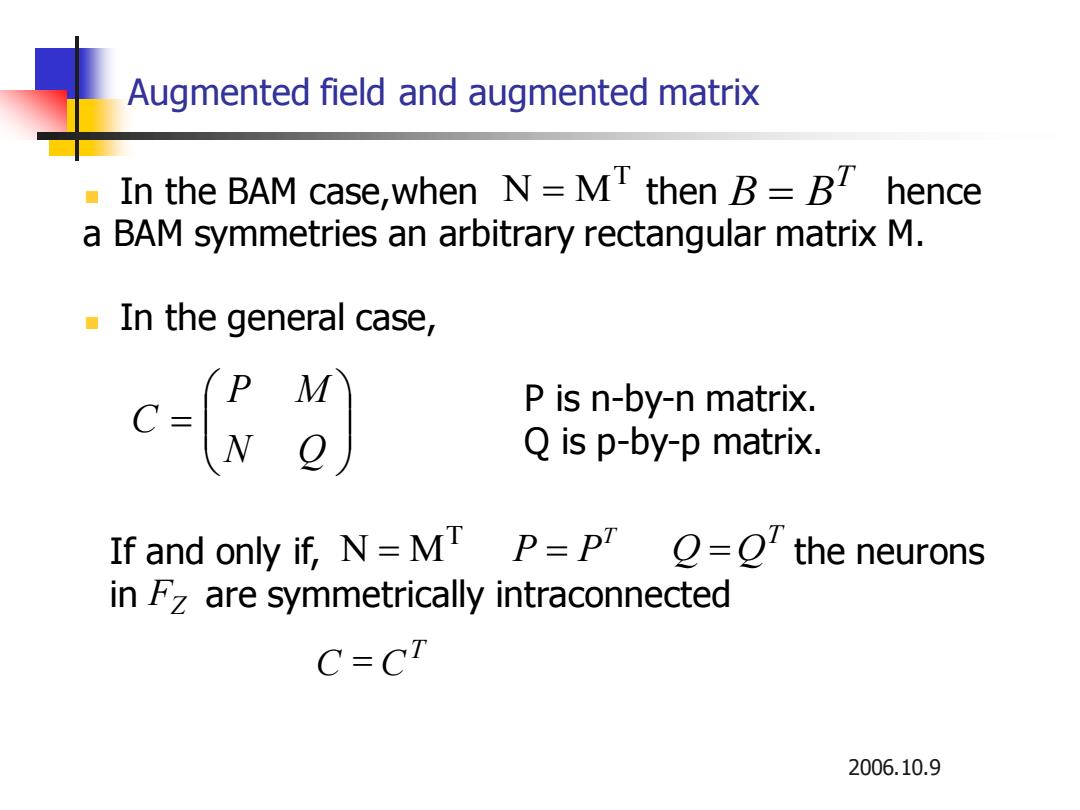
Augmented field and augmented matrix In the BAM case,when N=MT then B=BT hence a BAM symmetries an arbitrary rectangular matrix M. In the general case, c= P P is n-by-n matrix. Q is p-by-p matrix. If and only if,N=MT P=p?Q=0"t the neurons in Fz are symmetrically intraconnected C=C! 2006.10.9
2006.10.9 Augmented field and augmented matrix In the BAM case,when then hence a BAM symmetries an arbitrary rectangular matrix M. In the general case, P is n-by-n matrix. Q is p-by-p matrix. M T N = T B = B = N Q P M C If and only if, the neurons in are symmetrically intraconnected T N = M T P = P T Q = Q FZ T C = C
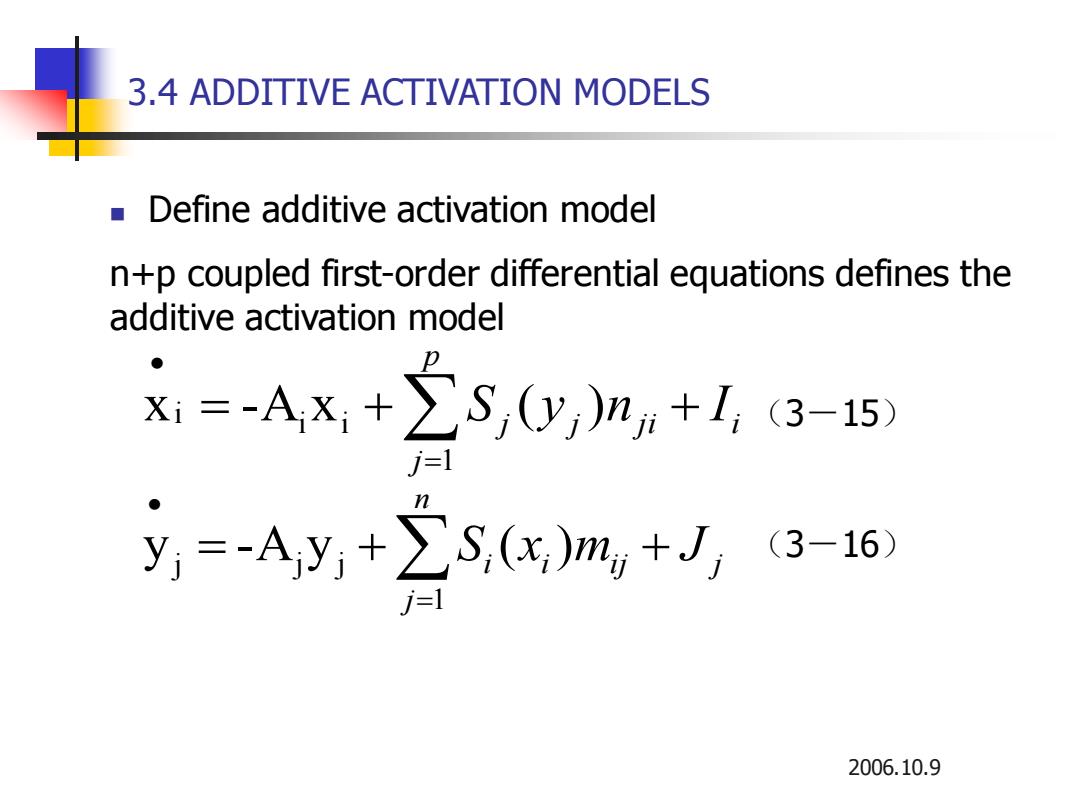
3.4 ADDITIVE ACTIVATION MODELS Define additive activation model n+p coupled first-order differential equations defines the additive activation model X=-AX+S,0,)nn+1,3-15 i=1 y,=-A,y+∑S,(cm,+J, (3-16) i=1 2006.10.9
2006.10.9 3.4 ADDITIVE ACTIVATION MODELS ◼ Define additive activation model n+p coupled first-order differential equations defines the additive activation model = • = + + p j j j j i i S y n I 1 i i xi - A x ( ) = • = + + n j i i i j j S x m J 1 j j j y - A y ( ) (3-15) (3-16)