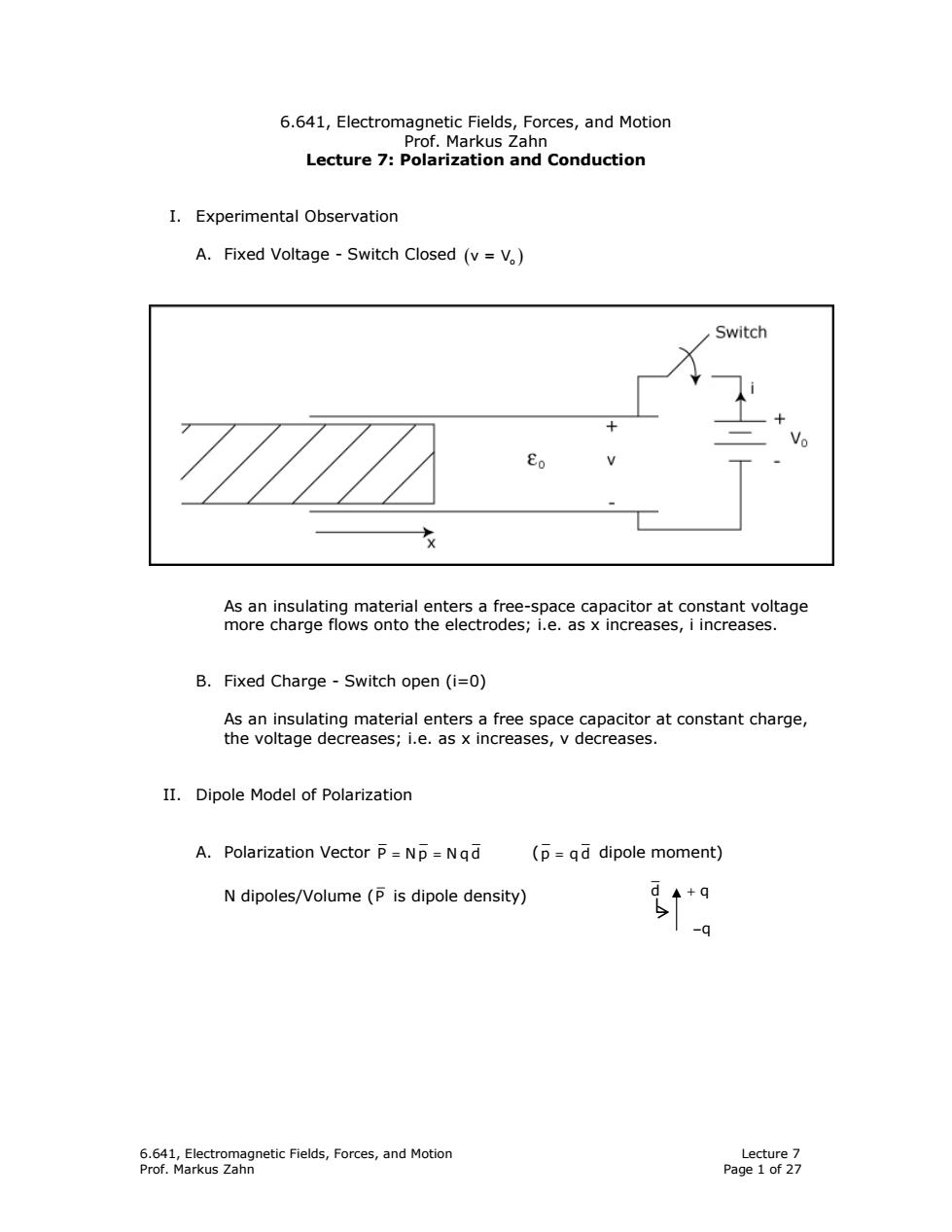
6.641,Electromagnetic Fields,Forces,and Motion Prof.Markus Zahn Lecture 7:Polarization and Conduction I.Experimental Observation A.Fixed Voltage-Switch Closed (v=V) Switch Eo As an insulating material enters a free-space capacitor at constant voltage more charge flows onto the electrodes;i.e.as x increases,i increases. B.Fixed Charge-Switch open(i=0) As an insulating material enters a free space capacitor at constant charge, the voltage decreases;i.e.as x increases,v decreases. II.Dipole Model of Polarization A.Polarization Vector P=Np =Nqd (p=gd dipole moment) N dipoles/Volume (P is dipole density) d+q \-q 6.641,Electromagnetic Fields,Forces,and Motion Lecture 7 Prof.Markus Zahn Page 1 of 27
6.641, Electromagnetic Fields, Forces, and Motion Lecture 7 Prof. Markus Zahn Page 1 of 27 6.641, Electromagnetic Fields, Forces, and Motion Prof. Markus Zahn Lecture 7: Polarization and Conduction I. Experimental Observation A. Fixed Voltage - Switch Closed (v=Vo ) As an insulating material enters a free-space capacitor at constant voltage more charge flows onto the electrodes; i.e. as x increases, i increases. B. Fixed Charge - Switch open (i=0) As an insulating material enters a free space capacitor at constant charge, the voltage decreases; i.e. as x increases, v decreases. II. Dipole Model of Polarization A. Polarization Vector P Np Nqd = = (p qd = dipole moment) N dipoles/Volume (P is dipole density) d q + −q
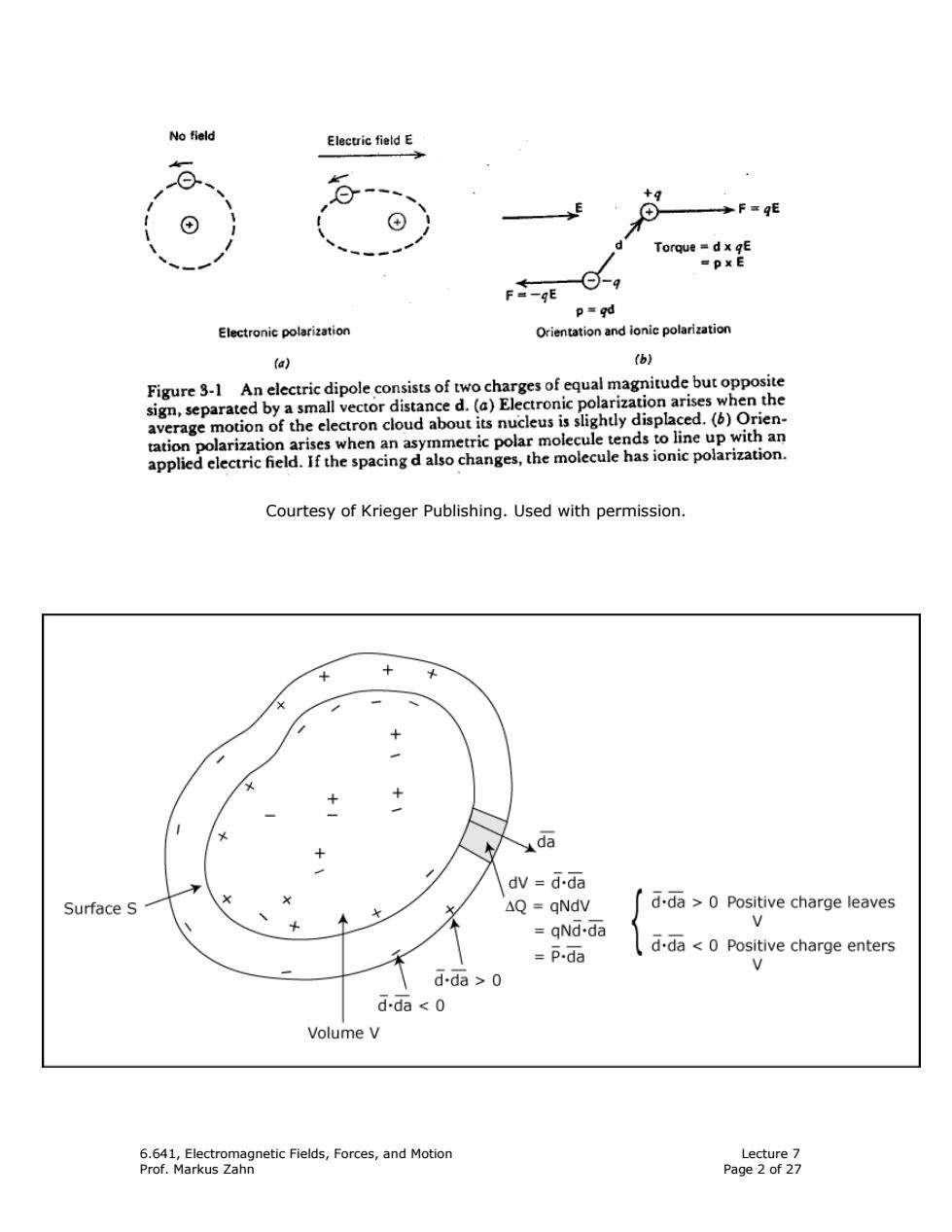
No field Electric field E +9 F=gE d Torque =dx gE =pxE p=gd Electronic polarization Orientation and ionic polarization (a) (b) Figure 3-1 An electric dipole consists of two charges of equal magnitude but opposite sign,separated by a small vector distance d.(a)Electronic polarization arises when the average motion of the electron cloud about its nucleus is slightly displaced.(b)Orien- tation polarization arises when an asymmetric polar molecule tends to line up with an applied electric field.If the spacing d also changes,the molecule has ionic polarization. Courtesy of Krieger Publishing.Used with permission. + da dV d-da Surface S + AQ qNdV d.da >0 Positive charge leaves qNd-da b =P.da d.da <0 Positive charge enters V d-da>o d.da <o Volume V 6.641,Electromagnetic Fields,Forces,and Motion Lecture 7 Prof.Markus Zahn Page 2 of 27
6.641, Electromagnetic Fields, Forces, and Motion Lecture 7 Prof. Markus Zahn Page 2 of 27 Courtesy of Krieger Publishing. Used with permission
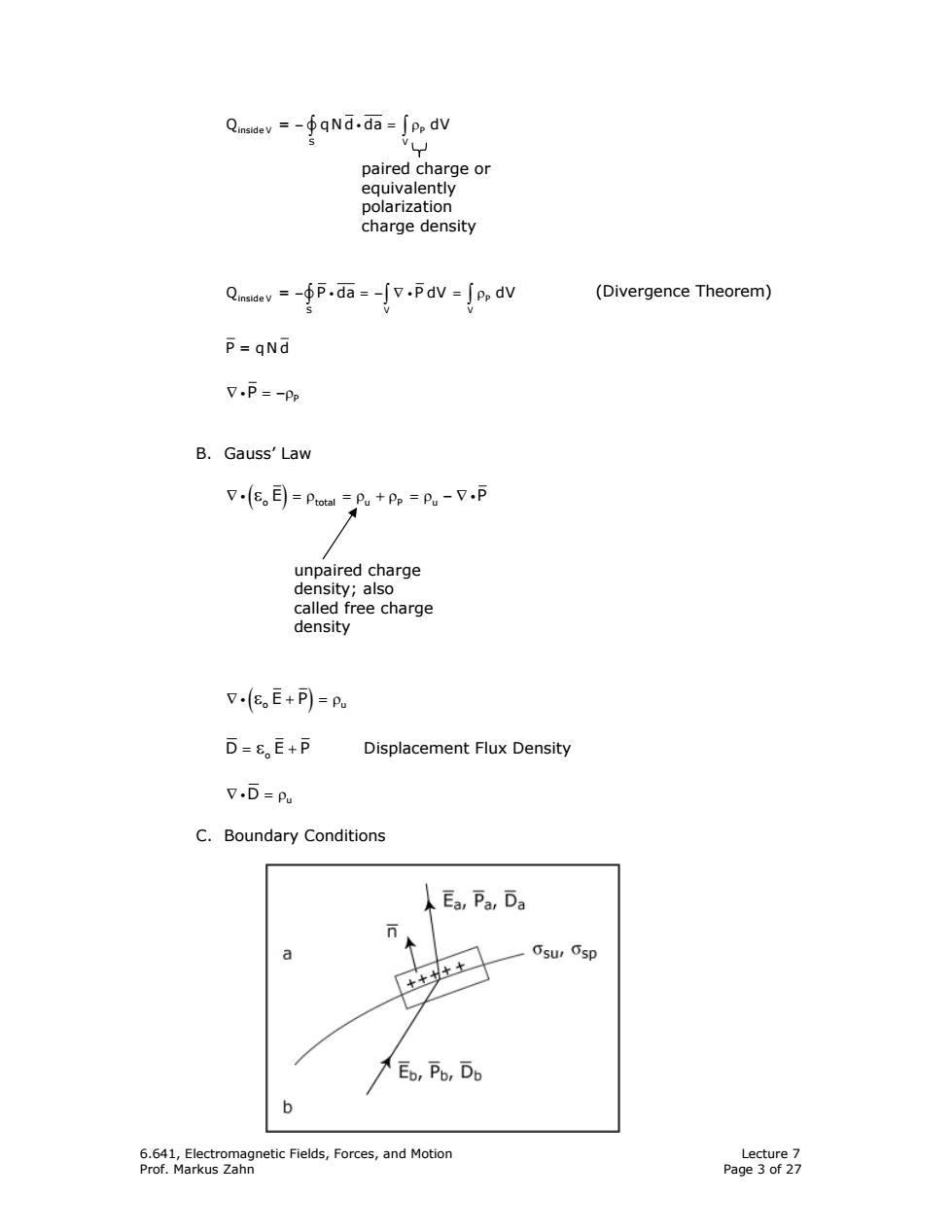
QinsldeV=-∮qNd.da-∫ppdV paired charge or equivalently polarization charge density Q--.da--v-dv-odV (Divergence Theorem) P=qNd V.P=-Pp B.Gauss'Law V,e。目=poa=putp=pu-V.p unpaired charge density;also called free charge density V(e。E+p=pu D=8。E+p Displacement Flux Density V.D=Pu C.Boundary Conditions Ea,Pa,Da ⊙ Osur Osp Eo,Po,Do b 6.641,Electromagnetic Fields,Forces,and Motion Lecture 7 Prof.Markus Zahn Page 3 of 27
6.641, Electromagnetic Fields, Forces, and Motion Lecture 7 Prof. Markus Zahn Page 3 of 27 − =ρ ∫ ∫ i inside V P v S V Q = qN d da dV − =− ∇ = ρ ∫∫ ∫ i i inside V P v SV V Q = P da P dV dV P = qNd (Divergence Theorem) ∇ = −ρ iP P B. Gauss’ Law ∇ =ρ =ρ +ρ =ρ −∇ i i ( ) εo total u P u E P ∇ + =ρ i( ) εo u E P D EP o = + ε Displacement Flux Density ∇ =ρ iD u C. Boundary Conditions paired charge or equivalently polarization charge density unpaired charge density; also called free charge density

7.d=p→∮D.da=jp.dv→n[ba-b]=ou p=-p,→∮p.da=-∫P,dv→n[p-p]=-op e,目)=p。+p→∮8E.da-p。+po)dV→n[。-Ed]=u+p D.Polarization Current Density △Q=qNdV=qNd.da=p.da [Amount of Charge passing through surface area element da] di,-AQ-OP.da [Current passing through surface ot ot area element da] =Jp.da polarization current density -爱 Ampere's law: vx斤=j。+jn+8。t =3+OP +t+8。t =i+e.E+p可 -i+阳 6.641,Electromagnetic Fields,Forces,and Motion Lecture 7 Prof.Markus Zahn Page 4 of 27
6.641, Electromagnetic Fields, Forces, and Motion Lecture 7 Prof. Markus Zahn Page 4 of 27 ∇ ρ ⇒ ρ ⇒ − =σ ⎡ ⎤ ∫ ∫ ⎣ ⎦ ii i v a b u u su S V D = D da = dV n D D ∇ −ρ ⇒ − ρ ⇒ − = −σ ⎡ ⎤ ∫ ∫ ⎣ ⎦ ii i v a b P = P da = dV n P P PP sp ( ) ( ) a b o u P o u P o su sp S V ∇ =ρ +ρ ⇒ = ρ +ρ ⇒ − σ +σ E E da dV n E E = ⎡ ⎤ ⎣ ⎦ εε ε ∫ ∫ i ii v D. Polarization Current Density ∆= = = Q qN dV qN d da P da i i [Amount of Charge passing through surface area element da ] ∂∆ ∂ = = ∂ ∂ i p Q P di da t t [Current passing through surface area element da ] = J da p i polarization current density ∂ = ∂ p P J t Ampere’s law: u p o E xH J J t ∂ ∇ =++ ∂ ε ∂ ∂ =+ + ∂ ∂ u εo P E J t t ( ) ∂ =+ + ∂ u εo J EP t u D J t ∂ = + ∂ S V

III.Equipotential Sphere in a Uniform Electric Field →0 十十 E=Eoiz Eolir cose-iesine] limΦ(r,0)=-E。rcos0 [Φ=-Ez=-E。rcos0] Φ(r=R,0)=0 R3 cose This solution is composed of the superposition of a uniform electric field plus the field due to a point electric dipole at the center of the sphere: aple=Cos with p=4πeE.R3 4πe。r2 This dipole is due to the surface charge distribution on the sphere. aΦ cos0 =3e.E。c0s0 6.641,Electromagnetic Fields,Forces,and Motion Lecture 7 Prof.Markus Zahn Page 5 of 27
6.641, Electromagnetic Fields, Forces, and Motion Lecture 7 Prof. Markus Zahn Page 5 of 27 III. Equipotential Sphere in a Uniform Electric Field ( ) o oo r lim r, E r cos E z E r cos → ∞ Φ θ =− θ Φ=− =− θ ⎡ ⎤ ⎣ ⎦ Φ = θ= ( ) r R, 0 ( ) 3 o 2 R r, E r cos r ⎡ ⎤ Φ θ =− − θ ⎢ ⎥ ⎣ ⎦ This solution is composed of the superposition of a uniform electric field plus the field due to a point electric dipole at the center of the sphere: dipole 2 o p cos 4 r θ Φ = πε with 3 p 4 ER o o = πε This dipole is due to the surface charge distribution on the sphere. ( )( ) 3 s or o oo 3 rR rR 2R r R, E r R, E 1 cos r r = = ∂Φ ⎡ ⎤ σ = θ = = θ =− = + θ ⎢ ⎥ ∂ ⎣ ⎦ ε εε 3 E cos o o = ε θ