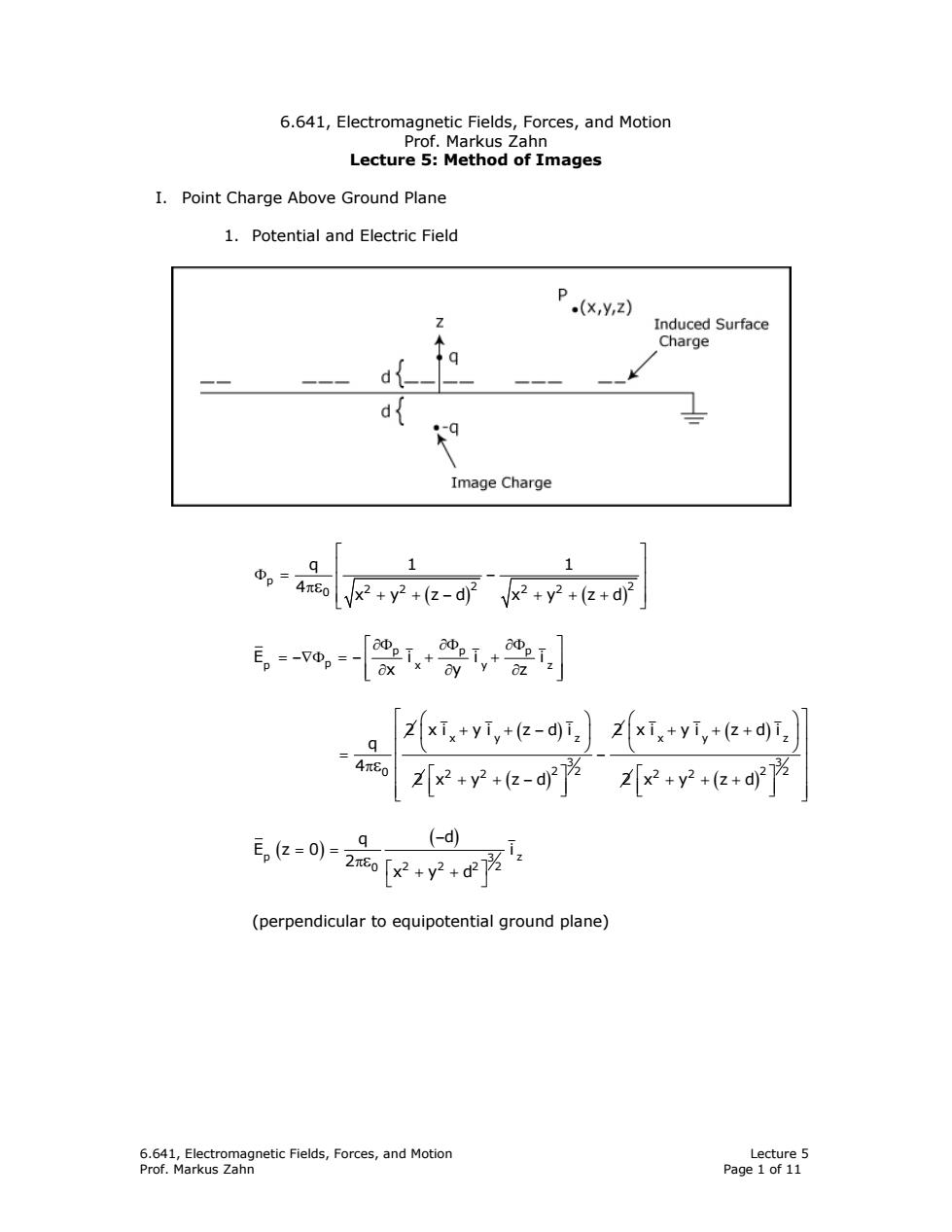
6.641,Electromagnetic Fields,Forces,and Motion Prof.Markus Zahn Lecture 5:Method of Images I.Point Charge Above Ground Plane 1.Potential and Electric Field 0 (x,y,z) Z Induced Surface Charge q d d - 个 Image Charge Φp= q 1 4πe0 ye-y ax 9 z(xI.tyi,e-0T.)Zxiyi(+ 40 z[2+y+-7z[x+y++形 q (-d) =eg (perpendicular to equipotential ground plane) 6.641,Electromagnetic Fields,Forces,and Motion Lecture 5 Prof.Markus Zahn Page 1 of 11
6.641, Electromagnetic Fields, Forces, and Motion Lecture 5 Prof. Markus Zahn Page 1 of 11 6.641, Electromagnetic Fields, Forces, and Motion Prof. Markus Zahn Lecture 5: Method of Images I. Point Charge Above Ground Plane 1. Potential and Electric Field ( ) ( ) p 22 22 2 2 0 q1 1 4 x y zd x y zd ⎡ ⎤ ⎢ ⎥ Φ= − π + +− + ++ ⎣ ⎦ ε ___ ppp E iii p xyz p xyz ⎡ ⎤ ∂Φ ∂Φ ∂Φ = −∇Φ = − + + ⎢ ⎥ ∂∂∂ ⎣ ⎦ 0 2 q 4 = πε ( ) __ _ xy z xi yi z d i 2 ⎛ ⎞ ⎜ ⎟ + +− ⎝ ⎠ ( ) 3 2 2 2 2 2 x y zd − ⎡ ⎤ + +− ⎢ ⎥ ⎣ ⎦ ( ) __ _ xy z xi yi z d i 2 ⎛ ⎞ ⎜ ⎟ + ++ ⎝ ⎠ ( ) 3 2 2 2 2 x y zd ⎡ ⎤ ⎢ ⎥ ⎢ ⎥ ⎢ ⎥ ⎢ ⎡ ⎤ + ++ ⎥ ⎢ ⎢ ⎥ ⎣ ⎦ ⎥ ⎣ ⎦ ( ) (− ) = = π ⎡ ⎤ + + ⎣ ⎦ ε _ p z 3 0 2 2 22 q d Ez0 i 2 xyd (perpendicular to equipotential ground plane)
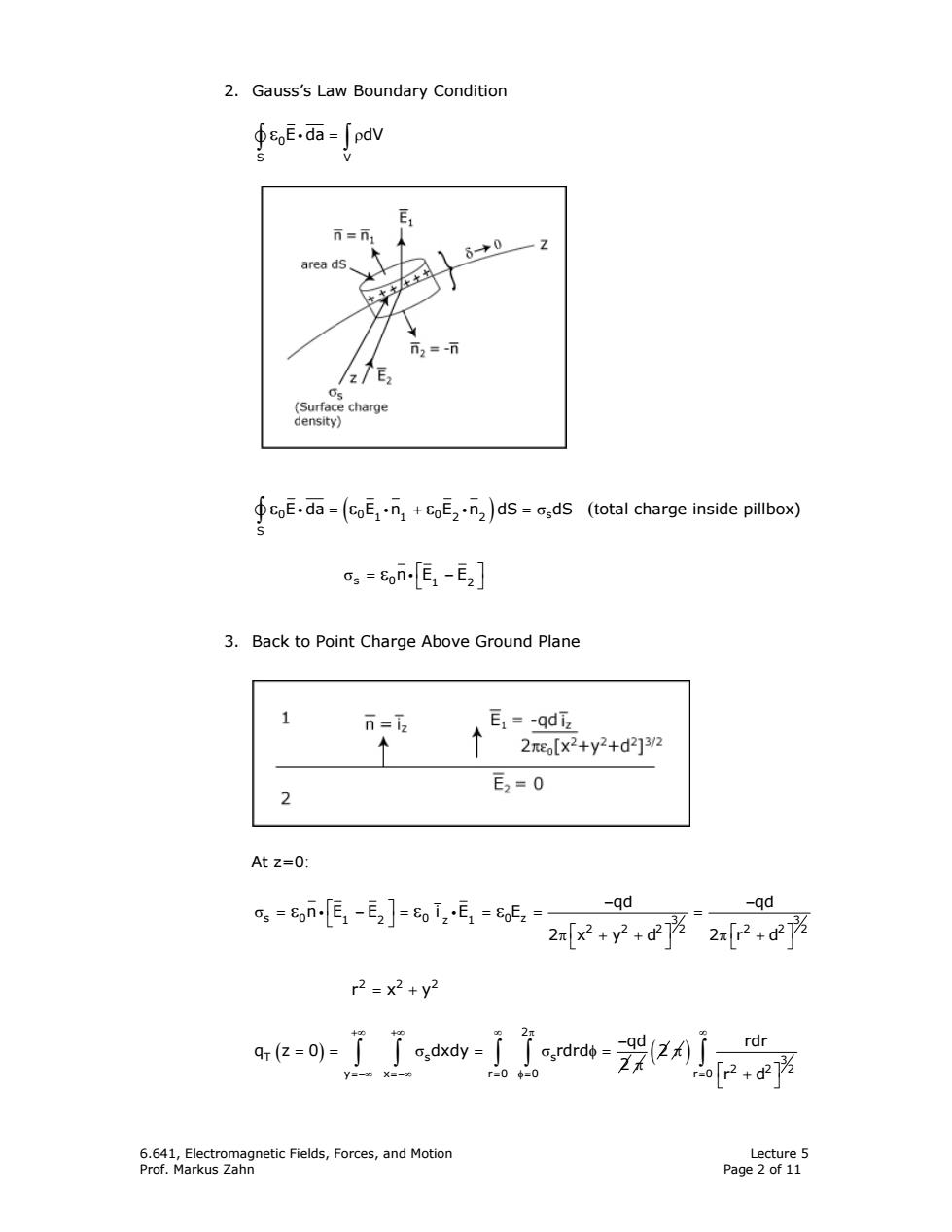
2.Gauss's Law Boundary Condition ∮E.da=jpav 万=1 area ds 土在土寸 i2=-万 E2 Os (Surface charge density) ()dsd(total charge inside plbox) o,=on…[月,-E2] 3.Back to Point Charge Above Ground Plane n=2 E =-qdiz 个 2e[x2+y2+d2]32 E2=0 2 At z=0: -6]6i.242 -qd -gd 2-[x2+y+d72[P+ r2=x2+y2 a-11ww-wzn 6.641,Electromagnetic Fields,Forces,and Motion Lecture 5 Prof.Markus Zahn Page 2 of 11
6.641, Electromagnetic Fields, Forces, and Motion Lecture 5 Prof. Markus Zahn Page 2 of 11 2. Gauss’s Law Boundary Condition 0 S V ε E da dV = ρ ∫ ∫ i v 0 00 s ( ) 11 22 S εεε E da E n E n dS dS = + =σ ∫ i ii v (total charge inside pillbox) s 0 1 2 σ= − nE E ⎡ ⎤ ⎣ ⎦ ε i 3. Back to Point Charge Above Ground Plane _ s 0 0 0z 1 2 z1 3 3 2 2 22 2 22 qd qd nE E i E E 2x y d 2r d − − σ= − = = = = ⎡ ⎤ ⎣ ⎦ π ++ π + ⎡ ⎤ ⎡⎤ ⎣ ⎦ ⎣⎦ ε εε i i = + 2 22 r xy ( ) 2 T ss y x r0 0 qd q z 0 dxdy rdrd 2 +∞ +∞ ∞ π = −∞ = −∞ = φ= − = = σ = σ φ= ∫ ∫ ∫∫ π ( ) 2 π 3 2 22 r 0 rdr r d ∞ = ⎡ ⎤ + ⎣ ⎦ ∫ At z=0:

u=r2+d2 du 2rdr rdr 37 「d=u为=-1 3/ [r2+ 2+ 92=o)=4 =-q -q2 f。-4co2d -q2 -16mEod2 II.Point Charge and Sphere 1.Grounded Sphere Conducting sphere at zero potential inducing chorgc b outside sphere (a) Inducing charge inside sphere g-8 g D D ) Figure 2-27 (a)The field due to a point charge q.a distance D outside a conducting sphere of radius R.can be found by placing a single image charge -gR/D at a distance b=RD from the center of the sphere.(b)The same relations hold true if the charge q is inside the sphere but now the image charge is outside the sphere,since D<R. Courtesy of Krieger Publishing.Used with permission. 6.641,Electromagnetic Fields,Forces,and Motion Lecture 5 Prof.Markus Zahn Page 3 of 11
6.641, Electromagnetic Fields, Forces, and Motion Lecture 5 Prof. Markus Zahn Page 3 of 11 2 2 u r d du 2rdr =+⇒ = 1 2 3 3 2 2 2 22 2 rdr du 1 u 2u r d r d − = =− =− ⎡ ⎤ + + ⎣ ⎦ ∫ ∫ T ( ) 2 2 0 qd qz0 q r d ∞ + = = =− + ( ) _ _ 2 2 q z 2 2 0 0 q q f i 4 2d 16 d − − = = πε πε II. Point Charge and Sphere 1. Grounded Sphere Courtesy of Krieger Publishing. Used with permission

s-[2+D2-2rDcos,s[b+r2-2rbcoso r=R=0÷g-(g-( q2s2=q2s2q2R2+D2-2RD cos0=q2b2+R2-2Rbcos0 q2(R2+D2)=q2(b2+R2) g心Z0os-70as雨一g-8 BR2)-8R2-2-6g0小R2-0 o-0-)-0 6、R2 D D2→q'=-qR/D force on sphere qq' -q"R/D -q2RD 4πe(D-b)2 4π80 R2 4(D2-R27 2.Isolated Sphere [Put additional Image Charge +q'=+qR/D at center] (zero charge) Φ(r=R)=,g'=q 4xEoR4xED 6.641,Electromagnetic Fields,Forces,and Motion Lecture 5 Prof.Markus Zahn Page 4 of 11
6.641, Electromagnetic Fields, Forces, and Motion Lecture 5 Prof. Markus Zahn Page 4 of 11 0 1 q q' 4 s s' ⎛ ⎞ Φ= + ⎜ ⎟ πε ⎝ ⎠ = + − θ = +− θ ⎡ ⎤⎡ ⎤ ⎣ ⎦⎣ ⎦ 1 1 2 2 2 22 2 s r D 2rD cos , s' b r 2rb cos ( ) 2 2 q q' q q' rR 0 s s' s s' − ⎛⎞ ⎛ ⎞ Φ= =⇒ = ⇒ = ⎜⎟ ⎜ ⎟ ⎝⎠ ⎝ ⎠ 2 2 22 2 2 2 2 2 2 q s ' q' s q' R D 2RD cos q b R 2Rb cos = ⇒ + − θ= + − θ ⎡ ⎤ ⎡ ⎤ ⎣ ⎦ ⎣ ⎦ ( ) ( ) 2 2 2 22 2 q' R D q b R += + 2 +q' 2 RD cos θ 2 = +q 2 Rb cos θ 2 2 q' b q D ⇒ = ( ) 2 b R 22 22 2 2 RD bR bb DR 0 D D ⎛ ⎞ + =+⇒− ++ = ⎜ ⎟ ⎝ ⎠ ( ) 2 R bDb 0 D ⎛ ⎞ − ⎜ ⎟ − = ⎝ ⎠ 2 R b D = 2 22 2 2 b R q' q q q' qR D D D = = ⇒ =− force on sphere ( ) ( ) 2 2 x 22 2 2 2 2 0 0 0 qq' q R D q RD f 4 Db R 4 DR 4 D D − − == = π − ⎛ ⎞ π − π − ⎜ ⎟ ⎝ ⎠ ε ε ε 2. Isolated Sphere [Put additional Image Charge +q' qR D = + at center] (zero charge) ( ) 0 0 q' q r R 4R4D Φ= = = π π ε ε
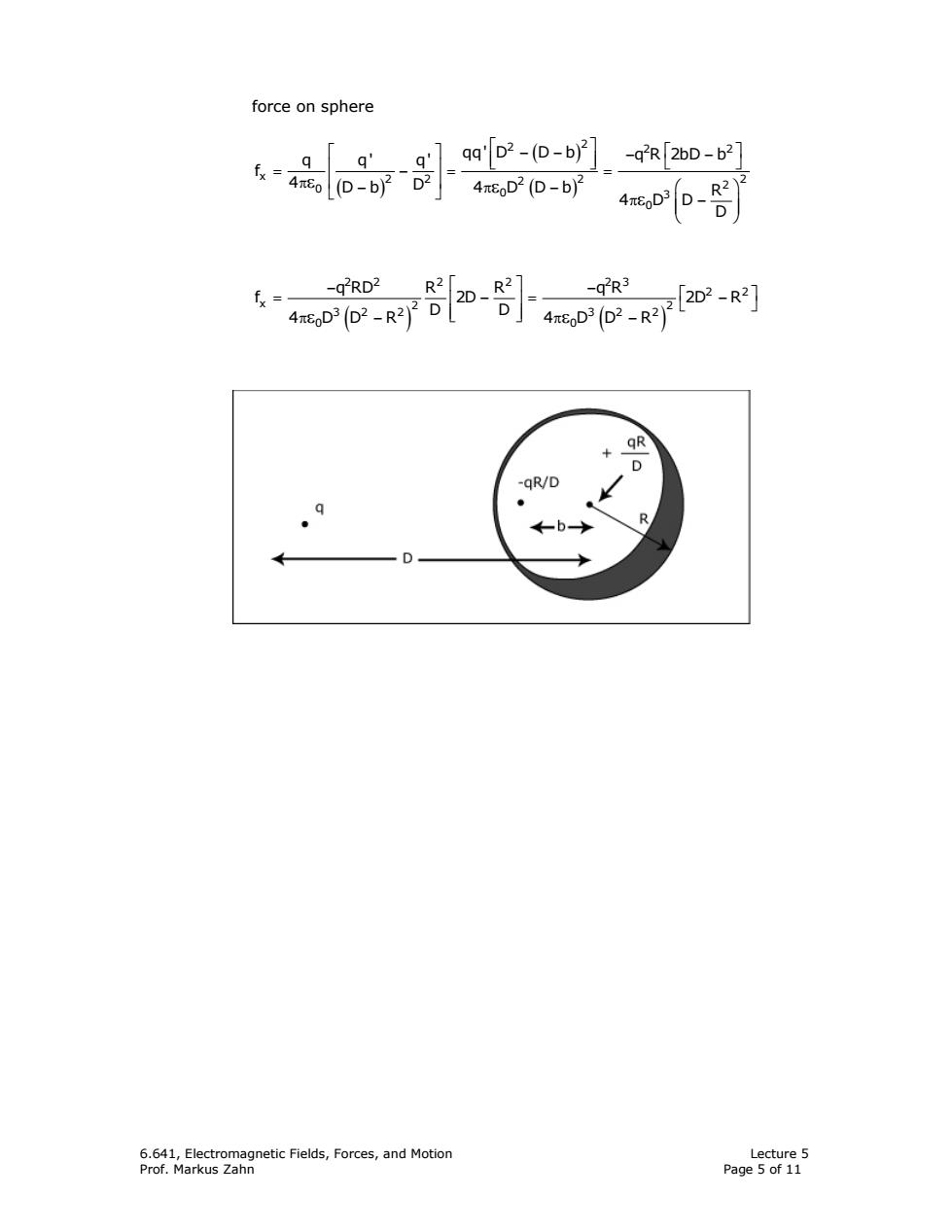
force on sphere q g' qq'D2-(D-b)-q'R[2bD-b2] fx= '7 4e0 1(D-b2 D2 4xoD2 (D-b)2 4p0-g】 -q'RD2 o R2[ -q2R3 4xEoD (D2-R2)2 D 48D2(D2-R2 [2D2-R2] qR D -qR/D ←b→ D 6.641,Electromagnetic Fields,Forces,and Motion Lecture 5 Prof.Markus Zahn Page 5 of 11
6.641, Electromagnetic Fields, Forces, and Motion Lecture 5 Prof. Markus Zahn Page 5 of 11 force on sphere ( ) ( ) ( ) 2 2 2 2 x 2 2 2 2 2 2 0 0 3 0 qq' D D b q R 2bD b q q' q' f 4 D b D 4 DDb R 4 DD D ⎡ ⎤ ⎡ ⎤ − − − − ⎡ ⎤ ⎢ ⎥ ⎣ ⎦ ⎣⎦ = − ⎢ ⎥ = = π ⎢ ⎥ − π− ⎛ ⎞ ⎣ ⎦ π − ⎜ ⎟ ⎝ ⎠ ε ε ε ( ) ( ) 22 2 2 2 3 2 2 x 2 2 32 2 32 2 0 0 q RD R R q R f 2D 2D R D D 4 DD R 4 DD R − − ⎡ ⎤ = − = − ⎡ ⎤ ⎢ ⎥ ⎣ ⎦ π − ε ε ⎢ ⎥ ⎣ ⎦ π −