
Abstract Vector Spaces Li Zhang Department of Mathematics Tongji University 4口,+心,4左4生+定QC
Abstract Vector Spaces Li Zhang Department of Mathematics Tongji University

Real Vector Space We will skip Chapter 1. Definition A vector space over R or(for short)a real vector space is a triple (V,+,where. 1 V is a set, 2 +is a binary operator that assigns to any pair v1,v2E V a new element vr+v2∈V, 3·is a binary operation that assigns to any pair c∈R and c∈Va new vector c.u∈V The operation satisfies 5 axioms. 4口++心++左+4生+定QC
Real Vector Space We will skip Chapter 1. Definition A vector space over R or (for short) a real vector space is a triple (V, +, ·) where, 1 V is a set, 2 + is a binary operator that assigns to any pair v1, v2 ∈ V a new element v1 + v2 ∈ V , 3 · is a binary operation that assigns to any pair c ∈ R and c ∈ V a new vector c·v ∈ V . The operation + satisfies 5 axioms

Axioms for Addition Al Commutativity u+v=v+u. A2 Associativity (u+v)+0=u+(v+w) A3 Existence of the zero vector There exists a unique element 0 of V such that v+0=v,for all v∈V A4 Existence of an additive inverse For each v E V,there exists a vector -v such that v+(-v)=0. We will abbreviate u+(-v)for u-v,so we have defined subtraction
Axioms for Addition + A1 Commutativity u + v = v + u. A2 Associativity (u + v) + w = u + (v + w). A3 Existence of the zero vector There exists a unique element 0 of V such that v + 0 = v, for all v ∈ V. A4 Existence of an additive inverse For each v ∈ V , there exists a vector −v such that v + (−v) = 0. We will abbreviate u + (−v) for u − v, so we have defined subtraction
Axioms for scalar multiplication. S1 Associativity c·(c2w)=(c1c2)v. S2 Distributivity (1st version) (C1+C2)v=cv+C2v. S3 Distributivity (2nd version) c(v1+2)=CU1+C2 S4 1U=U. 4口+心左4生主9QG
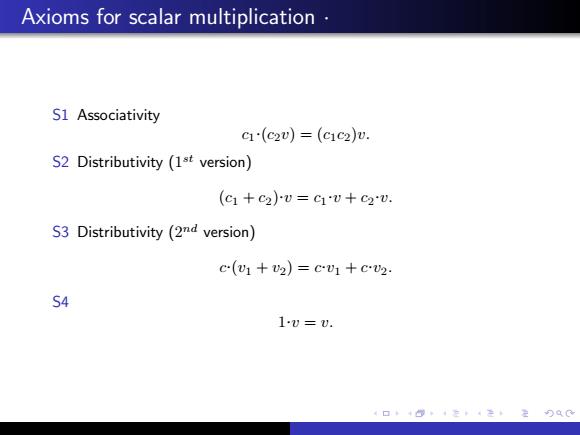
Axioms for scalar multiplication · S1 Associativity c1·(c2v) = (c1c2)v. S2 Distributivity (1 st version) (c1 + c2)·v = c1·v + c2·v. S3 Distributivity (2 nd version) c·(v1 + v2) = c·v1 + c·v2. S4 1·v = v

Vector Space Axioms We will call the axioms A1,A2,A3,A4 and S1,S2,S3,S4 the vector space axioms. We will prove shortly that 0v=0, and (-1)w=-w. 4口+++左+4生+定QC
Vector Space Axioms We will call the axioms A1, A2, A3, A4 and S1, S2, S3, S4 the vector space axioms. We will prove shortly that 0·v = 0, and (−1)v = −v