
Spanning Sets and Independent Sets Li Zhang Department of Mathematics Tongji University 悠 4口+心左4生主9QG
Spanning Sets and Independent Sets Li Zhang Department of Mathematics Tongji University

Span Definition Let v1.v2,....Un be the vectors in a vector space V.A vector uEV is said to be a linear combination of v1.v2.....vn if there exist scalars c1. C2,·,Cn such that u C1v1+c2v2+...+CnUn. The set of all linear combinations of v1,v2.....Un is said to the span of U1.U2,....Un written S(v1,v2,...,Un)or span (v1,v2,...Un) 4口+心左4生主9QG
Span Definition Let v1, v2, . . ., vn be the vectors in a vector space V . A vector u ∈ V is said to be a linear combination of v1, v2, . . ., vn if there exist scalars c1, c2, . . ., cn such that u = c1v1 + c2v2 + . . . + cnvn. The set of all linear combinations of v1, v2, . . ., vn is said to the span of v1, v2, . . ., vn written S (v1, v2, . . . , vn) or span (v1, v2, . . . , vn)
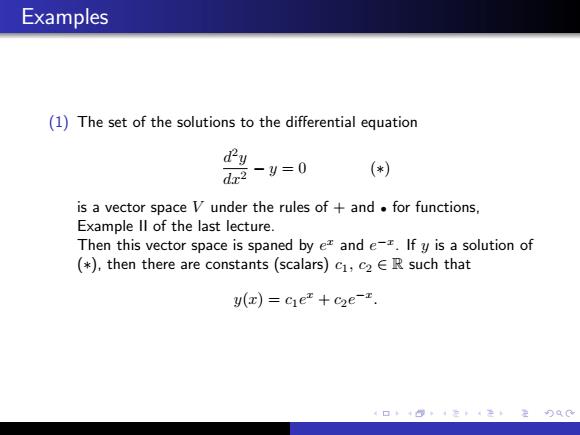
Examples (1)The set of the solutions to the differential equation Py dz2-=0 (*) is a vector space V under the rules of and.for functions, Example ll of the last lecture. Then this vector space is spaned by e2 and e-.If y is a solution of (*)then there are constants(scalars)c1,c2 ER such that y(x)=cle=+ce-=. 4口+++左+4生+定QC
Examples (1) The set of the solutions to the differential equation d 2y dx2 − y = 0 (∗) is a vector space V under the rules of + and • for functions, Example II of the last lecture. Then this vector space is spaned by e x and e −x . If y is a solution of (∗), then there are constants (scalars) c1, c2 ∈ R such that y(x) = c1e x + c2e −x

Examples (continued) (2)V=R3.e1=(1,0,0),e2=(0,1,0),e3=(0,0,1).Then S(e1,e2)=the zy-plane. 4口+心左4生+主9QG
Examples (continued) (2) V = R 3 , e1 = (1, 0, 0), e2 = (0, 1, 0), e3 = (0, 0, 1). Then S(e1, e2) = the xy-plane

Subspaces Suppose X is a set with a binary operationo so given any pair 1. x2∈X we have1ox2∈X. Let Y be a subset of X.We say Y is closed under o if for any pair y1,y2∈Y we have1o2∈Y. Definition Let(V,+,.)be a vector space and U C V be a subset.Then U is said to be a subvector space or subspace of V if (i)U is closed under + (ii)U is closed under.. 4口++心++左+4生+定QC
Subspaces Suppose X is a set with a binary operation ◦ so given any pair x1, x2 ∈ X we have x1◦x2 ∈ X. Let Y be a subset of X. We say Y is closed under ◦ if for any pair y1, y2 ∈ Y we have y1◦y2 ∈ Y . Definition Let (V, +, •) be a vector space and U ⊂ V be a subset. Then U is said to be a subvector space or subspace of V if (i) U is closed under +, (ii) U is closed under •