
Introduction Examples oA 1 x 1 matrix is nonsingular if it is nonzero. o An involutory matrix is its own inverse A2=1. o If A-(&8)uhee=d-c+0 then -由) 色电这女子 Matrix Theory Inverse -7/35
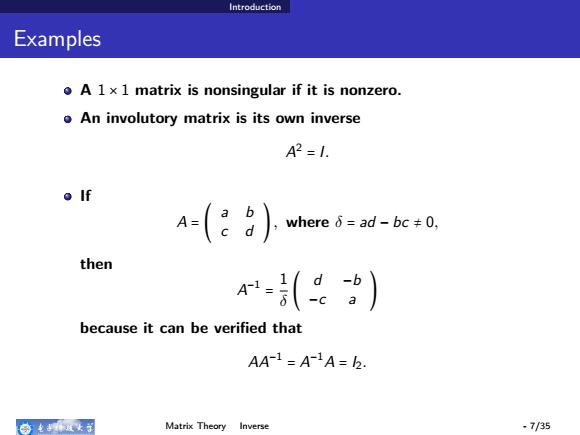
Introduction Examples A 1 × 1 matrix is nonsingular if it is nonzero. An involutory matrix is its own inverse A 2 = I. If A = ( a b c d ) , where δ = ad − bc ≠ 0, then A −1 = 1 δ ( d −b −c a ) because it can be verified that AA −1 = A −1A = I2. Matrix Theory Inverse - 7/35
Introduction Examples ●A1×1 matrix is nonsingular if it is nonzero. o An involutory matrix is its own inverse A2=1. o If a(:8) wheread-c+ then -引) because it can be verified that AA-1=A-1A=2. 务老环这女子 Matrix Theory Inverse -7/35
Introduction Examples A 1 × 1 matrix is nonsingular if it is nonzero. An involutory matrix is its own inverse A 2 = I. If A = ( a b c d ) , where δ = ad − bc ≠ 0, then A −1 = 1 δ ( d −b −c a ) because it can be verified that AA−1 = A −1A = I2. Matrix Theory Inverse - 7/35

Introduction Fact Although not all matrices are invertible,when an inverse exists,it is unique. 务老环这女子 Matrix Theory Inverse -8/35
Introduction Fact Although not all matrices are invertible, when an inverse exists, it is unique. ⇒ The inverse is unique. Proof. A ∈ C n×n AB = BA = In, where B ∈ C n×n AC = CA = In, where C ∈ C n×n Then, B = BIn = B(AC) = (BA)C = InC = C. Matrix Theory Inverse - 8/35

Introduction Fact Although not all matrices are invertible,when an inverse exists,it is unique. The inverse is unique. 奇电有这女了 Matrix Theory Inverse -8/35
Introduction Fact Although not all matrices are invertible, when an inverse exists, it is unique. ⇒ The inverse is unique. Proof. A ∈ C n×n AB = BA = In, where B ∈ C n×n AC = CA = In, where C ∈ C n×n Then, B = BIn = B(AC) = (BA)C = InC = C. Matrix Theory Inverse - 8/35

Introduction Fact Although not all matrices are invertible,when an inverse exists,it is unique. The inverse is unique. Proof. ●AE Cnxn 色电这女子 Matrix Theory Inverse -8/35
Introduction Fact Although not all matrices are invertible, when an inverse exists, it is unique. ⇒ The inverse is unique. Proof. A ∈ C n×n AB = BA = In, where B ∈ C n×n AC = CA = In, where C ∈ C n×n Then, B = BIn = B(AC) = (BA)C = InC = C. Matrix Theory Inverse - 8/35