电子科技大学：《图像处理及应用 Image Processing and Application》课程教学资源（课件讲稿）Chapter 04 Filtering in the Frequency Domain

电子科发女学光电科学与工程学院 SCHOOL OF OPTOELECTRONIC SCIENCE AND ENGINEERING OF UESTC 3.Impulses and Their Sifting Property A unit impulse of continuous variable 0=gua0i=1 Sifting property f()6()d=f(o) t=( f(t)8(t-1)di=f(o)1=1

( ) , 0 , 0, 0 t t t   = =    ◼ Sifting property 3. Impulses and Their Sifting Property f t t dt ( ) ( ) f (0)  − =  f t t t dt ( ) ( 0 ) f t( 0 )  − − =  ◼ A unit impulse of continuous variable s t t dt . . 1  ( )  − =  t = 0 0 t t =

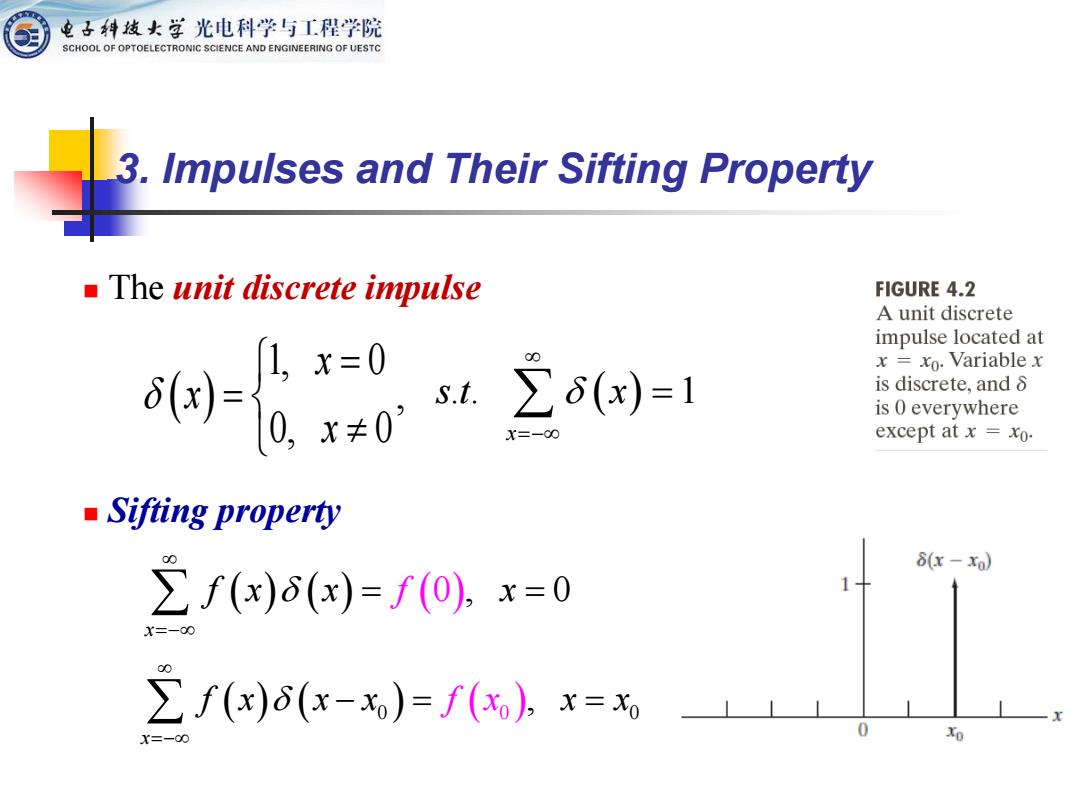
电子科发女学光电科学与工程学院 SCHOOL OF OPTOELECTRONIC SCIENCE AND ENGINEERING OF UESTC 3.Impulses and Their Sifting Property The unit discrete impulse FIGURE 4.2 A unit discrete impulse located at x=xo.Variable x is discrete,andδ is 0 everywhere except at x xo. -Sifting property 8(x-xo) ∑f(x)6(x)=f(0),x=0 ∑f(x)6(x-)=f(x),x=x-

( ) 1, 0 , 0, 0 x x x   = =    ◼ Sifting property 3. Impulses and Their Sifting Property ( ) ( ) (0), 0 x f x x x  f  =−  = = ( ) ( 0 0 ) ( 0 ), x f x x  x x x f x  =−  − = = ◼ The unit discrete impulse . . 1 ( ) x s t x   =−  =

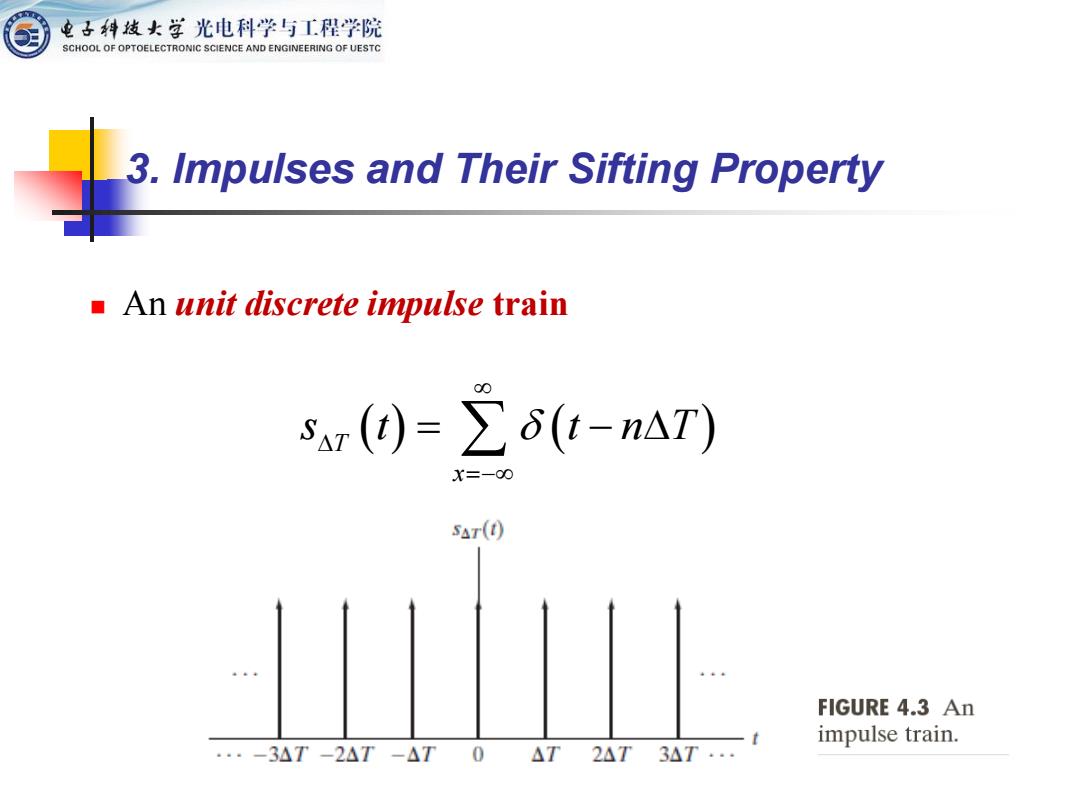
电子科发女学光电科学与工程学院 SCHOOL OF OPTOELECTRONIC SCIENCE AND ENGINEERING OF UESTC 3.Impulses and Their Sifting Property An unit discrete impulse train sa(d)=∑6(t-nAT) X=-00 5Ar(0 FIGURE 4.3 An ·-3AT-2△T-△T0 △T 2AT 3AT .. impulse train

3. Impulses and Their Sifting Property ◼ An unit discrete impulse train ( ) ( ) T x s t t n T    =− = −  

电子科发女学光电科学与工程学院 SCHOOL OF OPTOELECTRONIC SCIENCE AND ENGINEERING OF UESTC 4.Fourier Transform of Functions of One Continuous Variable Fourier transform (FT)of a continuous variable ft) (=F()=()ed .The inverse Fourier transform (IFT)of F(u) F-()-F(

( ) ( ) ( ) j t 2 f t F f t e dt   + − −  = =      ( ) ( ) ( ) 1 2j t F f t F e d     + − −  = =      4. Fourier Transform of Functions of One Continuous Variable ◼ Fourier transform (FT) of a continuous variable f(t) ◼ The inverse Fourier transform (IFT) of F(u)

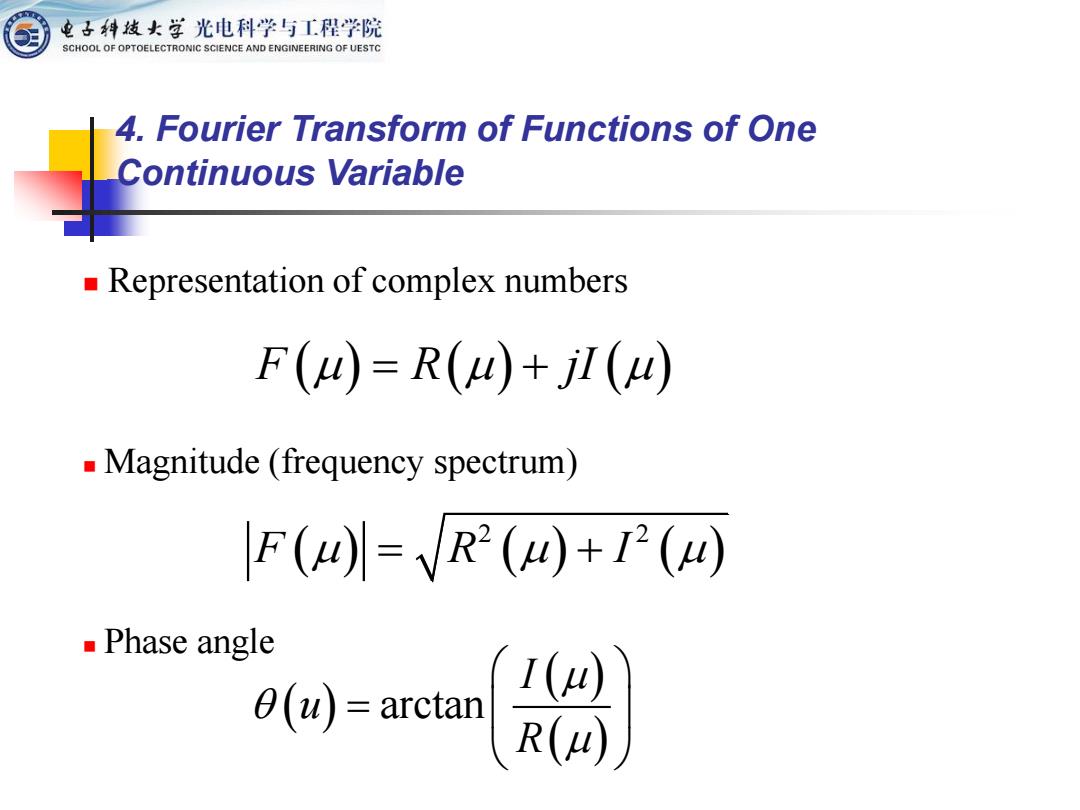
电子科发女学光电科学与工程学院 SCHOOL OF OPTOELECTRONIC SCIENCE AND ENGINEERING OF UESTC 4.Fourier Transform of Functions of One Continuous Variable Representation of complex numbers F(4)=R(4)+jI(u) Magnitude(frequency spectrum) F(=VR()+IP(4) Phase angle I(四 R()

F R jI (   ) = + ( ) ( ) ( ) ( ) ( ) 2 2 F R I    = + ( ) ( ) ( ) arctan I u R      =     4. Fourier Transform of Functions of One Continuous Variable ◼ Representation of complex numbers ◼ Magnitude (frequency spectrum) ◼ Phase angle