
Determinants o Matrices of lower order-Diagonal Method Main Diagonal←a11 a12 =a11a22-a12a21 Secondary Diag a21 a22 a11 a12a13 a11 a12 × D= a21 422 a23 21 a22 a31 a32 a33 a31 a32 D=a11a22a33+a12a23a31+13a21a32 -a11a23a32-a12a21a33-a13a22a31 Tongji University】 2/18
Determinants Matrices of lower order—Diagonal Method . a11. a12. a21. a22. . . . = a11a22 . Main Diagonal −a12a21 . Secondary Diag D = . a11. a12. a13. a11. a12. a21. a22. a23. a21. a22. a31. a32. a33. a31. a32. . . . − − − + + + D = a11a22a33 + a12a23a31 + a13a21a32 −a11a23a32 − a12a21a33 − a13a22a31 (Tongji University) Linear Algebra 2 / 18
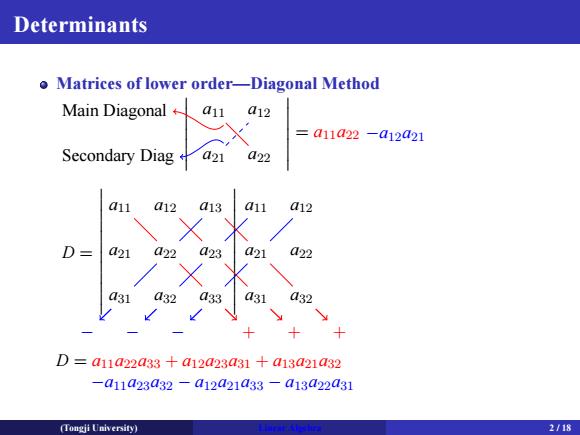
Determinants o Matrices of lower order-Diagonal Method Main Diagonal←a11 a12 =a11a22-a12a21 Secondary Diag a21 a22 a11 a12a13 a11 a12 × D= a21 422 a23 21 a22 a31 a32 a33 a31 a32 D=a11a22a33+a12a23a31+13a21a32 -a11a23a32-a12a21a33-a13a22a31 Tongji University】 2/18
Determinants Matrices of lower order—Diagonal Method . a11. a12. a21. a22. . . . = a11a22 . Main Diagonal −a12a21 . Secondary Diag D = . a11. a12. a13. a11. a12. a21. a22. a23. a21. a22. a31. a32. a33. a31. a32. . . . − − − + + + D = a11a22a33 + a12a23a31 + a13a21a32 −a11a23a32 − a12a21a33 − a13a22a31 (Tongji University) Linear Algebra 2 / 18
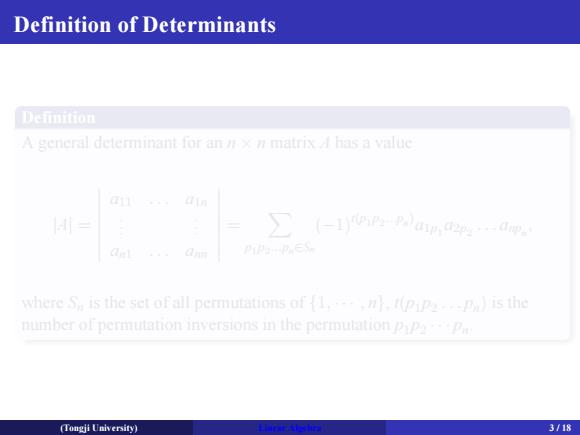
Definition of Determinants Defiition A general determinant for an nn matrix I has a value (1)pPP ai d2pdp dat...anm where Sn is the set ofall permutations of n t(ppp)is the number of permutation inversions in the permutation pipp (Tongji University) 3/18
Definition of Determinants . Definition . . A general determinant for an n × n matrix A has a value |A| = a11 . . . a1n . . . . . . an1 . . . ann = ∑ p1 p2 ...pn∈Sn (−1)t(p1 p2 ...pn ) a1p1 a2p2 . . . anpn , where Sn is the set of all permutations of {1, · · · , n}, t(p1 p2 . . . pn ) is the number of permutation inversions in the permutation p1 p2 · · · pn . (Tongji University) Linear Algebra 3 / 18
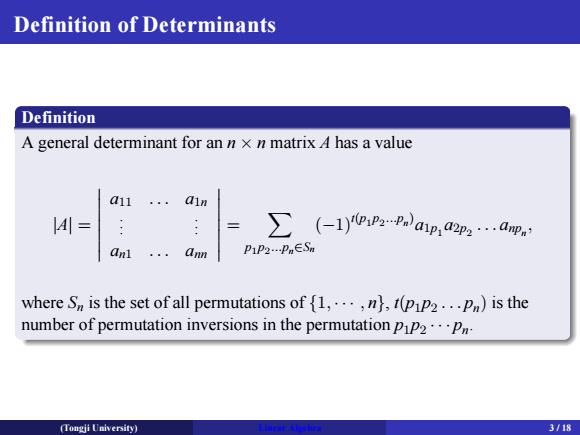
Definition of Determinants Definition A general determinant for an n x n matrix A has a value a11 ain 4= ∑(-l)PPPaip,2p2.ap.y anl...ann P1P2Pm∈Sn where Sn is the set of all permutations of {1,..,n},1(pip2...p)is the number of permutation inversions in the permutation pip2...Pn Tongji University】 3118
Definition of Determinants . Definition . . A general determinant for an n × n matrix A has a value |A| = a11 . . . a1n . . . . . . an1 . . . ann = ∑ p1 p2 ...pn∈Sn (−1)t(p1 p2 ...pn ) a1p1 a2p2 . . . anpn , where Sn is the set of all permutations of {1, · · · , n}, t(p1 p2 . . . pn ) is the number of permutation inversions in the permutation p1 p2 · · · pn . (Tongji University) Linear Algebra 3 / 18