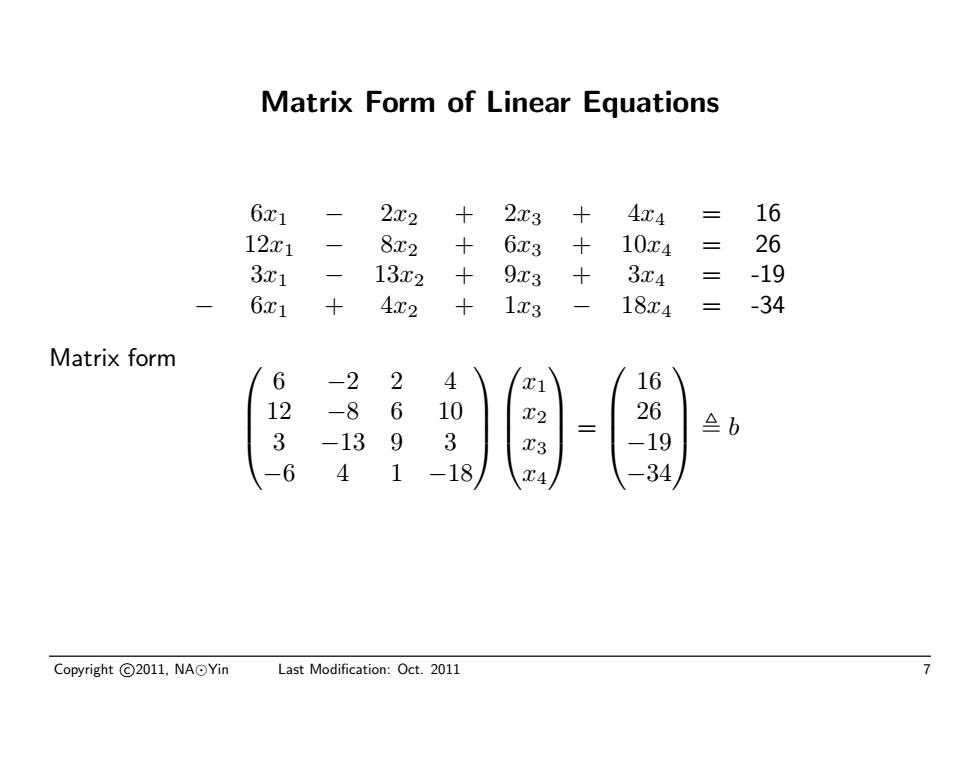
Matrix Form of Linear Equations 6x1 2x2 2x3 + 4x4 = 16 12x1 8x2 6x3 10x4 = 26 3x1 13c2 十 9x3 + 3x4 -19 6x1 + 4c2 + 1x3 18x4 = -34 Matrix form 6 -2 2 ¥ X1 16 12 -8 6 10 T2 26 b 3 -13 9 3 T3 -19 -6 4 1 -18 -34 Copyright©2011,NA⊙Yin Last Modification:Oct.2011 7
Matrix Form of Linear Equations 6x1 − 2x2 + 2x3 + 4x4 = 16 12x1 − 8x2 + 6x3 + 10x4 = 26 3x1 − 13x2 + 9x3 + 3x4 = -19 − 6x1 + 4x2 + 1x3 − 18x4 = -34 Matrix form 6 −2 2 4 12 −8 6 10 3 −13 9 3 −6 4 1 −18 x1 x2 x3 x4 = 16 26 −19 −34 , b Copyright c 2011, NA Yin Last Modification: Oct. 2011 7

Compact Form of Linear Equations 6x1 2x2 2x3 4x4 = 16 12x1 8x2 6x3 10x4 26 3x1 13x2 9x3 3x4 -19 6x1 + 4x2 +1x3 一 18x4 = -34 Compact form 6 -2 2 4 16 12 -8 6 10 26 3 -139 3 -19 -6 4 1 -18 -34 Proceeding with the forward elimination,.. Copyright©2011,NA⊙Yin Last Modification:Oct.2011 8
Compact Form of Linear Equations 6x1 − 2x2 + 2x3 + 4x4 = 16 12x1 − 8x2 + 6x3 + 10x4 = 26 3x1 − 13x2 + 9x3 + 3x4 = -19 − 6x1 + 4x2 + 1x3 − 18x4 = -34 Compact form 6 −2 2 4 16 12 −8 6 10 26 3 −13 9 3 −19 −6 4 1 −18 −34 Proceeding with the forward elimination, ... Copyright c 2011, NA Yin Last Modification: Oct. 2011 8

Forward Elimination-Example 6 -2 2 4 16 6 -2 4 16 -8 6 10 26 0 -4 2 -6 3 -13 9 3 -19 → 0 -12 1 -27 6 4 -18 -34 0 2 3 -14 -18 6 -2 2 4 16 6 -22 4 16 0 -4 2 2 -6 0 -4 2 2 6 → 0 0 2 -5 9 0 0 2 5 0 0 -13 -21 0 0 0 3 Matrix is upper triangular. Copyright©2011,NA⊙Yin Last Modification:Oct.2011 9
Forward Elimination–Example 6 −2 2 4 16 12 −8 6 10 26 3 −13 9 3 −19 −6 4 1 −18 −34 → 6 −2 2 4 16 0 −4 2 2 −6 0 −12 8 1 −27 0 2 3 −14 −18 → 6 −2 2 4 16 0 −4 2 2 −6 0 0 2 −5 −9 0 0 4 −13 −21 → 6 −2 2 4 16 0 −4 2 2 −6 0 0 2 −5 −9 0 0 0 −3 −3 Matrix is upper triangular. Copyright c 2011, NA Yin Last Modification: Oct. 2011 9
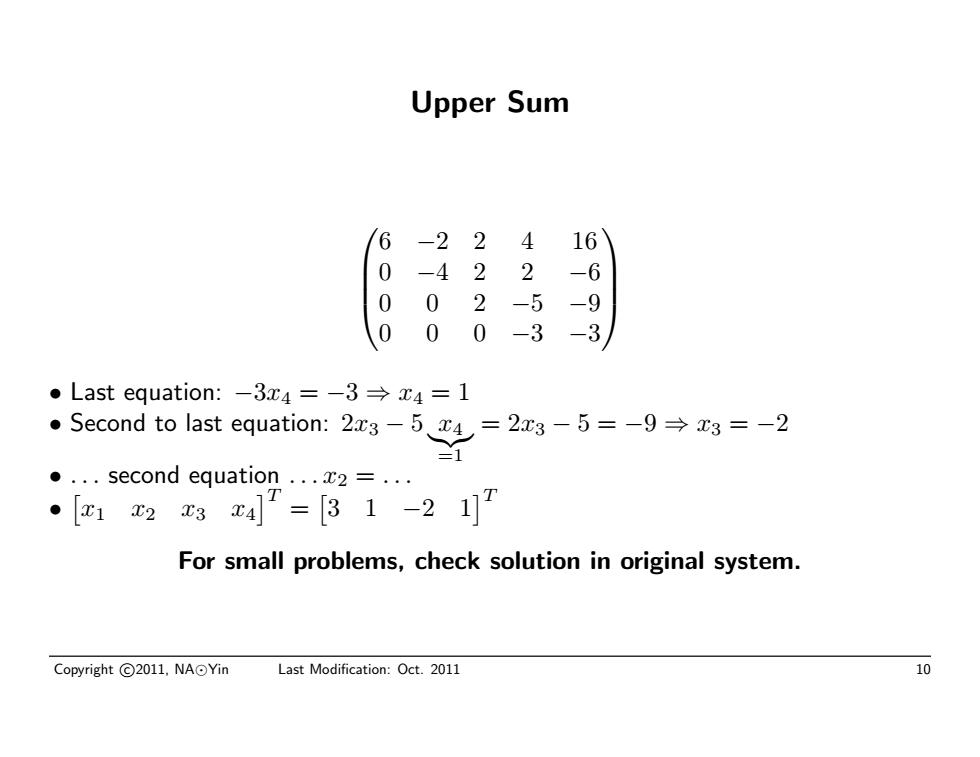
Upper Sum 6 -2 2 4 16 0 -4 2 2 -6 0 0 2 -5 -9 0 0 0 -3 -3 ●Last equation:-3x4=-3→x4=1 .Second to last equation:23-5 4 =23-5=-9 3=-2 =1 ..second equation...2=... ·12x3x4=B1-21]T 7 For small problems,check solution in original system. Copyright©2011,NA⊙Yin Last Modification:Oct.2011 10
Upper Sum 6 −2 2 4 16 0 −4 2 2 −6 0 0 2 −5 −9 0 0 0 −3 −3 • Last equation: −3x4 = −3 ⇒ x4 = 1 • Second to last equation: 2x3 − 5 x4 |{z} =1 = 2x3 − 5 = −9 ⇒ x3 = −2 • . . . second equation . . . x2 = . . . • x1 x2 x3 x4 T = 3 1 −2 1T For small problems, check solution in original system. Copyright c 2011, NA Yin Last Modification: Oct. 2011 10����
Linear Systems ●Introduction Naive Gaussian Elimination Limitations and Operation Counts ●LU factorization ●QR factorization Copyright©2011,NA⊙Yin Last Modification:Oct.2011 11
Linear Systems • Introduction • Naive Gaussian Elimination ⇒ Limitations and Operation Counts • LU factorization • QR factorization Copyright c 2011, NA Yin Last Modification: Oct. 2011 11