
Eigenvalue and Eigenvector →Introduction Power method and the variants ·Shifting ·Atekin Acceleration ●Summary Copyright©2011,NA⊙Yin Last Modification:Oct.2011 2
Eigenvalue and Eigenvector ⇒ Introduction • Power method and the variants • Shifting • Atekin Acceleration • Summary Copyright c 2011, NA Yin Last Modification: Oct. 2011 2

Introduction ●Given A∈Cmxm,find入∈R and x≠0∈Rm,so that Ax=入x →(A-I)x=0x≠0 →A-λI川=0 One approach for eigenvalue and eigenvector. Copyright©2011,NA⊙Yin Last Modification:Oct.2011 3
Introduction • Given A ∈ C n×n, find λ ∈ R and x 6= 0 ∈ R n, so that Ax = λx → (A − λI)x = 0 x 6= 0 → |A − λI| = 0 One approach for eigenvalue and eigenvector. Copyright c 2011, NA Yin Last Modification: Oct. 2011 3
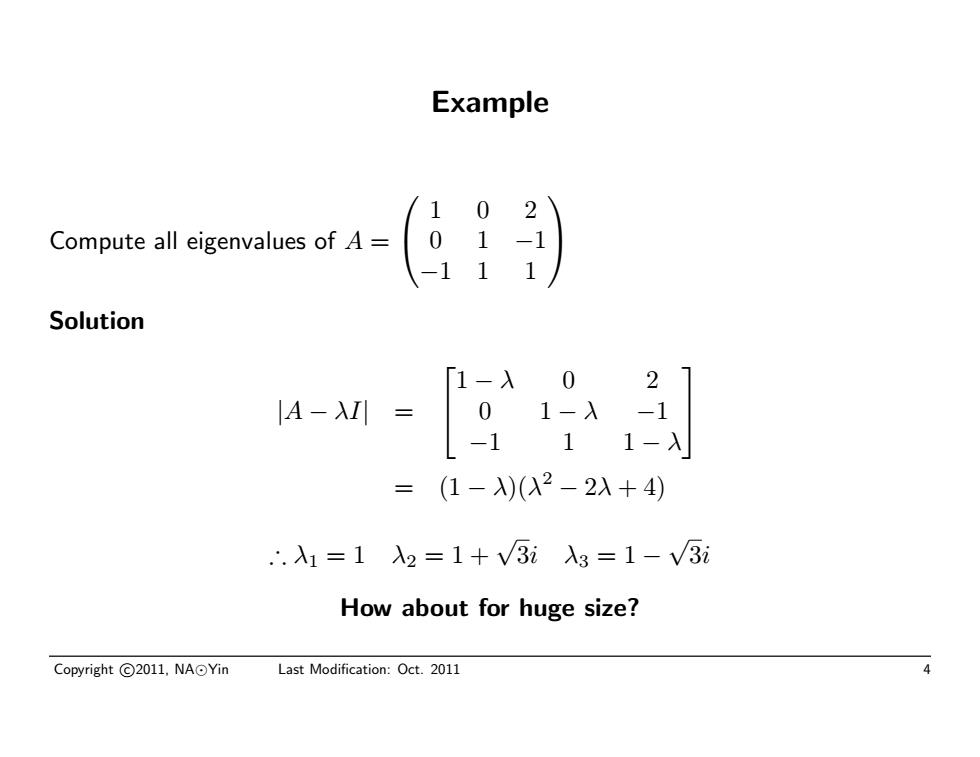
Example Compute all eigenvalues of A 1 Solution 11-λ =(1-)(2-2入+4) .λ1=1λ2=1+V3iλ3=1-V3i How about for huge size? Copyright©2011,NA⊙Yin Last Modification:Oct.2011 4
Example Compute all eigenvalues of A = 1 0 2 0 1 −1 −1 1 1 Solution |A − λI| = 1 − λ 0 2 0 1 − λ −1 −1 1 1 − λ = (1 − λ)(λ 2 − 2λ + 4) ∴ λ1 = 1 λ2 = 1 + √ 3i λ3 = 1 − √ 3i How about for huge size? Copyright c 2011, NA Yin Last Modification: Oct. 2011 4
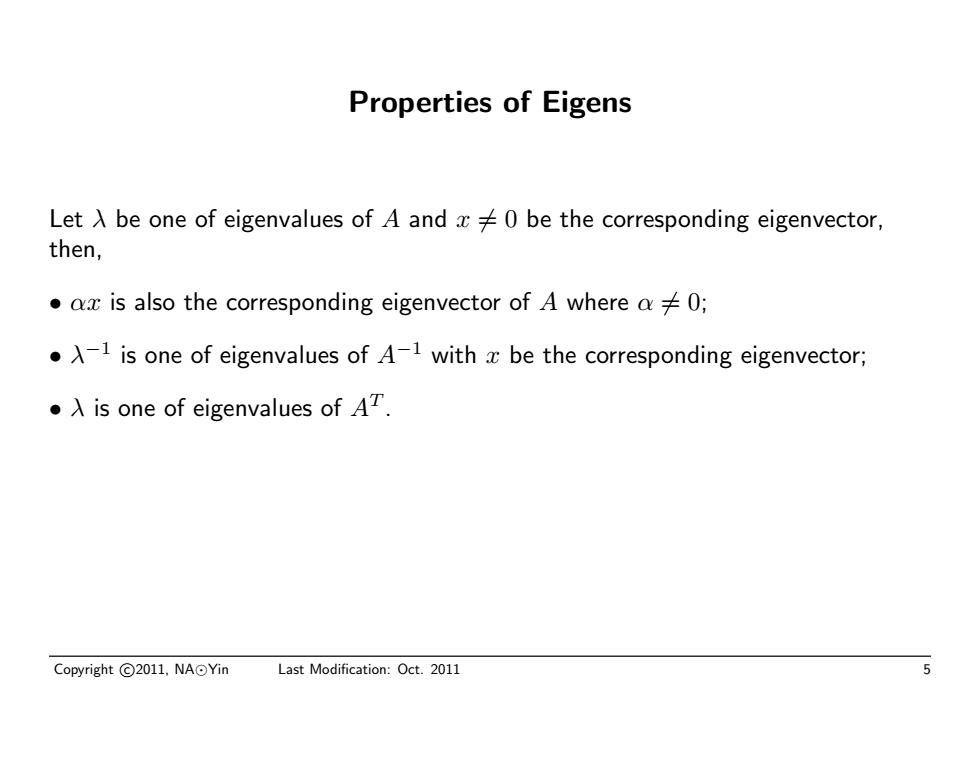
Properties of Eigens Let A be one of eigenvalues of A and x0 be the corresponding eigenvector, then, ax is also the corresponding eigenvector of A where a0; .A-1 is one of eigenvalues of A-1 with x be the corresponding eigenvector; ·入is one of eigenvalues of AT. Copyright©2011,NA⊙Yin Last Modification:Oct.2011 5
Properties of Eigens Let λ be one of eigenvalues of A and x 6= 0 be the corresponding eigenvector, then, • αx is also the corresponding eigenvector of A where α 6= 0; • λ −1 is one of eigenvalues of A−1 with x be the corresponding eigenvector; • λ is one of eigenvalues of AT . Copyright c 2011, NA Yin Last Modification: Oct. 2011 5

Properties of Eigens Let Ai,(i=1,2,...,n)be the eigenvalues of A and zi0 be the corresponding eigenvectors,then, ●∑21Xi=1ai where is the trace of A,denoted by tr(A); ·I1λi=|A where|A is determinant of A. ·A-a≤∑=1aal e.g. 01 1/20-1 Copyright©2011,NA⊙Yin Last Modification:Oct.2011 6
Properties of Eigens Let λi ,(i = 1, 2, . . . , n) be the eigenvalues of A and xi 6= 0 be the corresponding eigenvectors, then, • Pn i=1 λi = Pn i=1 aii where Pn i=1 aii is the trace of A, denoted by tr(A); • Qn i=1 λi = |A| where |A| is determinant of A. • |λ − aii| ≤ Pn j=1,j6=i |aij|. e.g. A = 1 0 −1 1 3 0 1/2 0 −1 Copyright c 2011, NA Yin Last Modification: Oct. 2011 6