同济大学：《结构力学》课程教学资源（教案讲稿，打印版）16 Dynamic analysis of structures

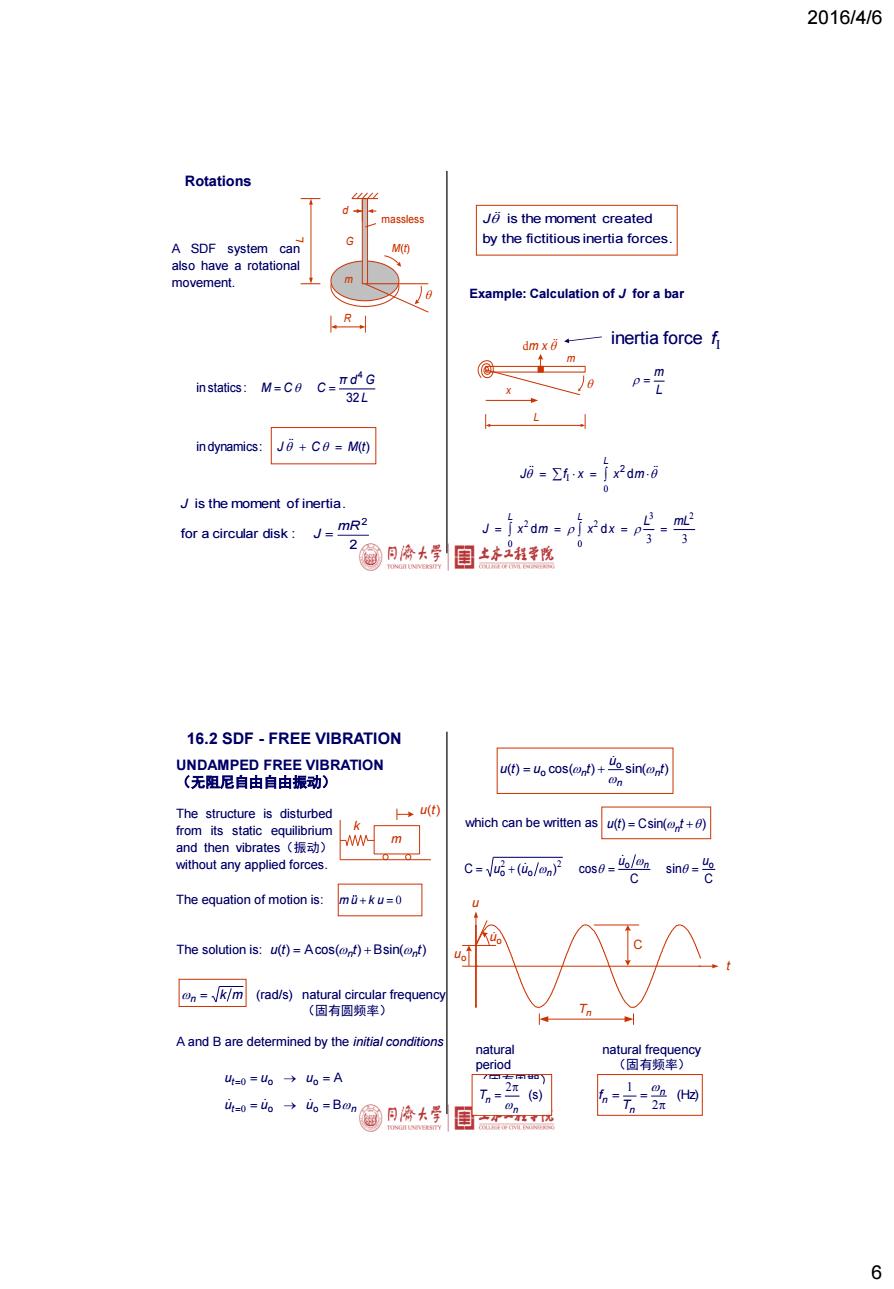
2016/4/6 Rotations J6 is the moment created by the fictitious inertia forces A SDF system can M(t) also have a rotational movement. Example:Calculation of J for a bar LR dmx6 inertia force f in statics: M=C0 C=md'G 32L in dynamics: J6+C0=M(t) Ji-∑所x=jx2dm-8 J is the moment of inertia. J=mR2 3_ml2 for a circular disk: 2 J-jxam-pixdx- 3 同桥大学 国土本相幸院 0 16.2 SDF-FREE VIBRATION UNDAMPED FREE VIBRATION u(t)=u cos(+osin() (无阻尼自由自由振动) n The structure is disturbed +u(t) from its static equilibrium which can be written as u(t)=Csin(t+) m and then vibrates(振动) without any applied forces C=√6+(/on2 cos8=6色2 sin-号 The equation of motion is: m0+ku=0 The solution is:u(t)=Acos(@t)+Bsin(@t) on=k/m (rad/s)natural circular frequency (固有圆频率) A and B are determined by the initial conditions natural natural frequency period (固有频率) t=0=u0→U0=A 1 4o=i。→=B@n盒同将大学 (s) n（H n 2 6

2016/4/6 6 Rotations indynamics: J   C   M(t)  2 for a circular disk : is the moment of inertia. 2 mR J J        L J f x x m 0  I    d 2 3 3 2 0 0 3 2 2 mL L J x m x x L L   d    d    L m   I inertia force f  massless m L R dG M(t)  m x  dm x L A SDF system can also have a rotational movement. L π d G M C C 32 in statics: 4    by the fictitious inertia forces. J  is the moment created Example: Calculation of J for a bar 16.2 SDF - FREE VIBRATION UNDAMPED FREE VIBRATION （无阻尼自由自由振动） u(t) Acos( t) Bsin( t)  n  n n  k m t n t u u u u u u B A o o o o            0 0 u(t)  Csin( t ) n (Hz) T f n n n    2 1  (rad/s) A and B are determined by the initial conditions which can be written as natural circular frequency （固有圆频率） The structure is disturbed from its static equilibrium and then vibrates（振动） without any applied forces. The equation of motion is: m u   k u  0 The solution is: ( ) cos( ) sin( ) o o t u u t u t n n n       k m u(t) C sin C C ( ) cos o o o o u u u u n   n         2 2 natural period （固有周期） natural frequency （固有频率） uo uo  Tn u t C T (s) n n    2

2016/4/6 Energy in undamped free vibration At each instant of time,the total energy E is made of two parts,the kinetic energy Ek and the strain energy Es. E()-ku) Ex(t)=mu(t) E。=max E.=0 Ek=0 Ek=max E(0=Ex)+E,() -mCnCOs(t++kCsint+ Remark:the conservation of energy can be used to derive the differential equation. -mC2cos(@nt+0)+kC2sin(nt+0) E0)-m+k -KC (k=m2)） conservation of energy dE =0 dt E(is constant,which implies conservation of energy. dE=m00+ku=0→m0+k知=0 d 同桥大学 国土本2程学院 16.3 Damped vibration(阻尼振动)of one degree systems A and B are determined by the initial conditions Friction in the structure is u(t) idealized by a linear viscous u(t)=-ore[Acos(@,t)+Bsin(t)] damper(线性粘滞阻尼器 )which develops a force +enL-Aa。sin(o)+Ba。cos(o, proportional to the velocity 6=-ca) 4=0=u0→6=A 4=0=i。→。=-nA+Bam。 The equation of motion is: mi+cǜ+ku=0 U(t)=e-iet u。coso,+。+sino.】 if c<c=2km (criticaldamping)the solutionis u(t)=e [Acos()+Bsin(t)] The solution can also be written as u(t)=Ce sin(t+0) sine=o damping ratio damped pulsation c Cr 2√km 0。=a,V-a C= 6+ cos9=4。+in46 Cap 同大学 士本工程学院 7

2016/4/6 7 Energy in undamped free vibration At each instant of time, the total energy E is made of two parts, the kinetic energy Ek and the strain energy Es. 2 2 1 E (t) m u(t) k   2 2 1 E (t) k u(t) s      C ( ) C cos ( ) C sin ( ) C cos( ) Csin( ) ( ) ( ) ( ) k k m m t k t m t k t E t E t E t n n n n n n n k s 2 2 2 2 2 2 2 2 2 2 1 2 1 2 1 2 1 2 1                        E(t) is constant, which implies conservation of energy. Remark : the conservation of energy can be used to derive the differential equation. 2 2 2 1 2 1 E(t)  m u  k u   0 t E d d conservation of energy  muu  kuu  0  mu  ku  0 t E    d d u t = max = 0 k E s E = 0 = k E s E max = 0 = k E s E max mu   c u   k u  0 if c c 2 km (criticaldamping) the solutionis  r  u(t) Acos( t) Bsin( t) D D t n      e km c c c r 2    2   1 D ωn    A sin( ) B cos( ) ( ) Acos( ) Bsin( ) t t u t t t D D D D D D t t n n n                  e  e t n D t u u u u u u ξω A Bω A 0 o o 0 o o                        ( ) cos( ) sin( ) o o o t u u u t u t D D D n t n        e ( ) C sin( )     u t t D t n e D u nu ω ξω θ C cos o  o   Friction in the structure is idealized by a linear viscous damper(线性粘滞阻尼器 )which develops a force proportional to the velocity f c u(t) D    A and B are determined by the initial conditions. The solution can also be written as The equation of motion is: damping ratio damped pulsation 2 2            D u u u n ω o ξω o C o  C sin uo θ  k m u(t) c 16.3 Damped vibration（阻尼振动）of one degree systems

2016/4/6 Ce-fai! p periods between two maximal points un and un+p tntp=in+pTo Un Ce-ontn sin(n+) Unsp Cewn(n+pTo)sin(o(n+pTo)+0) 2元 T。= =efenTo 2π Decay of motion nun=5anpT。=5n Un+p Onv1-52 A free vibration test can be used to determine experimentally the natural Un frequency(固有频率)and the damping 5<0.1→-g21 2xp of a structure. Un-p 同桥大学 目土本红相手悦 m /y(t) -my(t) 9 5<l(c<2mo)underdamped(欠阻尼) kuy(t) ci(t) Let @p=@v1-g2 +c少+k1y=0 y(t)=e-5m(c sin@pt+c2 cos@pt) )+250少+o2y=0 y(0)=yo,(0)=o 5=c/2m0 C1=(yo+0ya)/0D,C2=% y(t)=Ae y(t)=Ae-5 sin(@pt+op) 22+20入+o2=0 Proper equation A= V+(+) root入=-50±ioV1-52 Op tanPp =yoop/(o +soyo) 5=1(c=2mo) Critical damping y(t)=(C1+c2t)e(临界阻尼) 5>1(c>2mo) overdamped(过阻尼) C,=2m0 c.2mo =5 8

2016/4/6 8 Decay of motion pTD t t np  n  D D D D D pT n (t pT ) n t n p n n n n n n ( (t pT ) ) ( t ) u u ξω ξω ξω e e ω θ e ω θ          C sin C sin 2 n n n n p n 1 pT p u u D            2 ln n p n u u p         ln 2 1  0.1 1  1  2 n un p u and p points  periods between two maximal A free vibration test can be used to determine experimentally the natural frequency（固有频率）and the damping of a structure. D TD  2  t e C n n t n p t + un un+p u t m  y  cy   k11y  0 m y(t) m  y (t) ( ) 11 k y t cy (t)   c / 2m 2 0 2  y   y   y  t y t Ae ( )  2 0 2 2      Proper equation root 2    i 1  1(c  2m) Let 2  D  1 0 0 y(0)  y , y (0)  y  1 0 0 2 0 c (y y )/ ,c y     D  ( ) sin( ) D D t y t Ae  t      ( ) ( sin cos ) 1 2 y t e c t c t D D t       2 0 2 0 ( ) D y y A y      tan /( ) 0 0 0 y y y  D   D    1(c  2m) t y t c c t e  ( )  (  ) 1 2 cr  2m     m c c c r 2  1(c  2m) underdamped(欠阻尼) overdamped（过阻尼） Critical damping (临界阻尼)

2016/4/6 Example:Give the mass an initial displacement of 2cm by applying a force of 16.4kN.The amplitude reduces to 1 cm after 4 cycles within 2 seconds.Determine the parameters of the system. Solution: 2cm 1.Damping ratio(阻尼比)5= -In =0.0276 16.4kN 2π×41 2.Stiffness coefficient(刚度系数) 飞，=164x10 =8.2×105(N/m) 0.02 3.Undamped period(无阻尼周期) T。=2/4=0.5(s) T=ToV1-52=0.4998(s) 4.Weight 0=27-=12.5711s) 5.Damping coefficient(阻尼系数) T m=k1/o2=5190kg) c=2mo5=3601(N.s/m) W=-mg=50.86(kN)@向海大学 目土水工程学院 16.4 Undamped forced vibration of one-degree systems SDF-HARMONIC EXCITATIONS Example 2 y A harmonic load →U(t) is applied to the W m P。sin(et) m structure. y=u+ug k12 Without damping,the equation of motion is my=-ku 才7977777 mi+k u=po sin(ot) Ug(t)=Ugo sin(ot) Example 1 If u is to be studied (e.g.earthquake) The system consisting m(u+Ug)=-ku of the mass m and the excentric mass-m is =rsin(ot mi+ku =mugo @2 sin(at) considered for writing Newton's equation. 多k12k12 If y is to be studied(e.g.floor isolation) 7777 (m-而)ū+而(v+ū)=-ku my =-k(y-ug) mu+ku mro-sin(ot) my+k y =k uoo sin(ot) 同海大学 国士本工程学悦 9

2016/4/6 9 Example: Give the mass an initial displacement of 2cm by applying a force of 16.4kN. The amplitude reduces to 1 cm after 4 cycles within 2 seconds. Determine the parameters of the system. 2cm 16.4kN Solution: 1.Damping ratio（阻尼比） 0.0276 1 2 ln 2 4 1      2.Stiffness coefficient（刚度系数） 8.2 10 ( / ) 0.02 16.4 10 5 3 k1 1   N m   3.Undamped period（无阻尼周期）  2/ 4  0.5(s) TD 1 0.4998(s) 2 T  TD   4.Weight 12.57(1/s) 2   T   / 5190(kg) 2 m  k11   W  mg  50.86(kN) 5.Damping coefficient（阻尼系数） c  2m  3601(Ns/m) SDF - HARMONIC EXCITATIONS sin( ) o m u   k u  p t Example 1 sin( ) ( ) ( ) m u k u m r t m m u m v u k u   2            m u k u m u sin( t) m u u k u 2 go g          (   ) A harmonic load is applied to the structure. Without damping, the equation of motion is The system consisting of the mass m and the excentric mass m is considered for writing Newton’s equation. v = r sin(t) m m k / 2 k / 2 u k m u(t) sin( ) o p t u (t) u sin( t) g = go  k / 2 k / 2 u m Example 2 y y  u  ug m y   k u If u is to be studied (e.g. earthquake) m y k y k u sin( t) m y k y u go g          ( ) If y is to be studied (e.g. floor isolation) 16.4 Undamped forced vibration of one-degree systems

2016/4/6 WITHOUT DAMPING u(t) C and 6 are determined by the initial conditions Po sin(ot) u(t)is a summation of two sinus and is not mu+k u=po sin(oxt) defined for = In reality,the damping implies that u(t) The solution u(t)of the differential disappears after some time.Then the equation is the sum of two parts up(f)and solution steady state response )is U(⑨ u(t)=Un(t)+Up(t) u(t)=Up(t)=-Po/k (/sin(ot) homogeneous solution u(t)=Csin(t+6) conclusions particular solution up(t)=Asin(ot) After some time,the structure vibrates with Up(t)=-Ao2sin(ot) the same frequency as the applied force The amplitudes of the vibration are infinite →-mAo2+kA=P%→A= Po Polk I-(ofon)2 when =cn- k-ma u,()is the transient response(瞬态响应) u(t)=Csin(cnt+0)+ 6/k sin(@t) -(@ln)f u,(0 is the steady state response(稳态响应) 大学 闺土水2程学悦 NITH VISCOUS DAMPING(粘性阻尼) after calculations(see the book),it is obtained u(t) po sin(ot) m Po/k -M sin(ot-) V-(olonY]+25(oloaT 25(@l@d mu+cu+k u=po sin(ot) tan= 0<p<180 1-(@lon月 The homogeneous solution u(f)(transient response)disappears after some time. Remarks Un(t)=Cessin(+0) The total response is u(f)=un(f)+up(t).But after some time un(t)disappears and u(t)= The particular solution u(f)(steady state p(f)(steady state response) response)is of the form: After some time,the structure vibrates with the same frequency as the applied force. Up(t)=Asin(ot-) 土本工程学院 10

2016/4/6 10 WITHOUT DAMPING sin( ) o m u   k u  p t u (t)  Csin( t ) h ωn homogeneous solution particular solution u (t) Asin( t) p   2 2 1 ( ) / A A o o o n 2 p k k m p m kA p ω  ω ω          u (t) A sin( t) p   2    C and  are determined by the initial conditions u(t) is a summation of two sinus and is not defined for  = n. In reality, the damping implies that uh(t) disappears after some time. Then the solution ( steady state response ) is sin( ) ( ) ( ) ( ) o t p k u t u t n p    2 1   conclusions • After some time, the structure vibrates with the same frequency as the applied force. • The amplitudes of the vibration are infinite when  = n. up (t) is the steady state response(稳态响应) uh (t) is the transient response(瞬态响应) u(t) u (t) u (t)  h  p The solution u(t) of the differential equation is the sum of two parts uh (t) and up (t). k m u(t) sin( ) o p t sin( ) ( ) ( ) Csin( ) t p k u t t n o n ω 2 1         WITH VISCOUS DAMPING(粘性阻尼) sin( ) o m u   c u   k u  p t ( ) C sin( )     u t t D t h n e The homogeneous solution uh (t) (transient response) disappears after some time. The particular solution up (t) (steady state response) is of the form: u (t)  Asin(t ) p after calculations (see the book), it is obtained     sin( ) ( ) ( ) ( ) o            t p k u t n n p 2 2 2 1 2  0 180 1 2 2            n n ( ) ( ) tan Remarks • The total response is u(t) = uh(t) + up(t). But after some time uh(t) disappears and u(t) = up(t) (steady state response). • After some time, the structure vibrates with the same frequency as the applied force. k m u(t) c sin( ) o p t