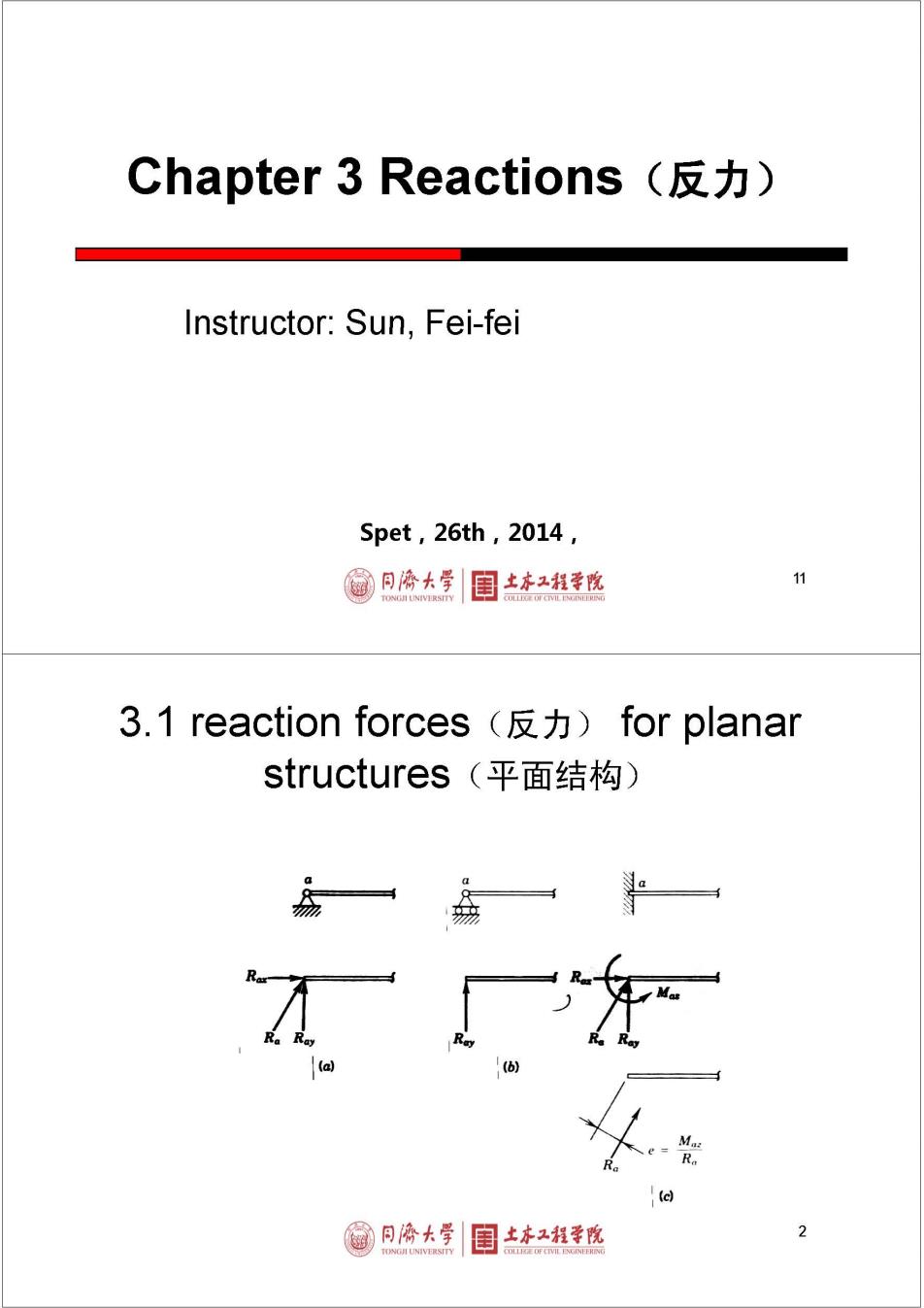
Chapter3 Reactions(反力) Instructor:Sun,Fei-fei Spet,26th,2014, 同大学 土亦工程学院 11 3.1 reaction forces(反力)for planar structures(平面结构) M (c) 同©大学 土本工相学脱 2
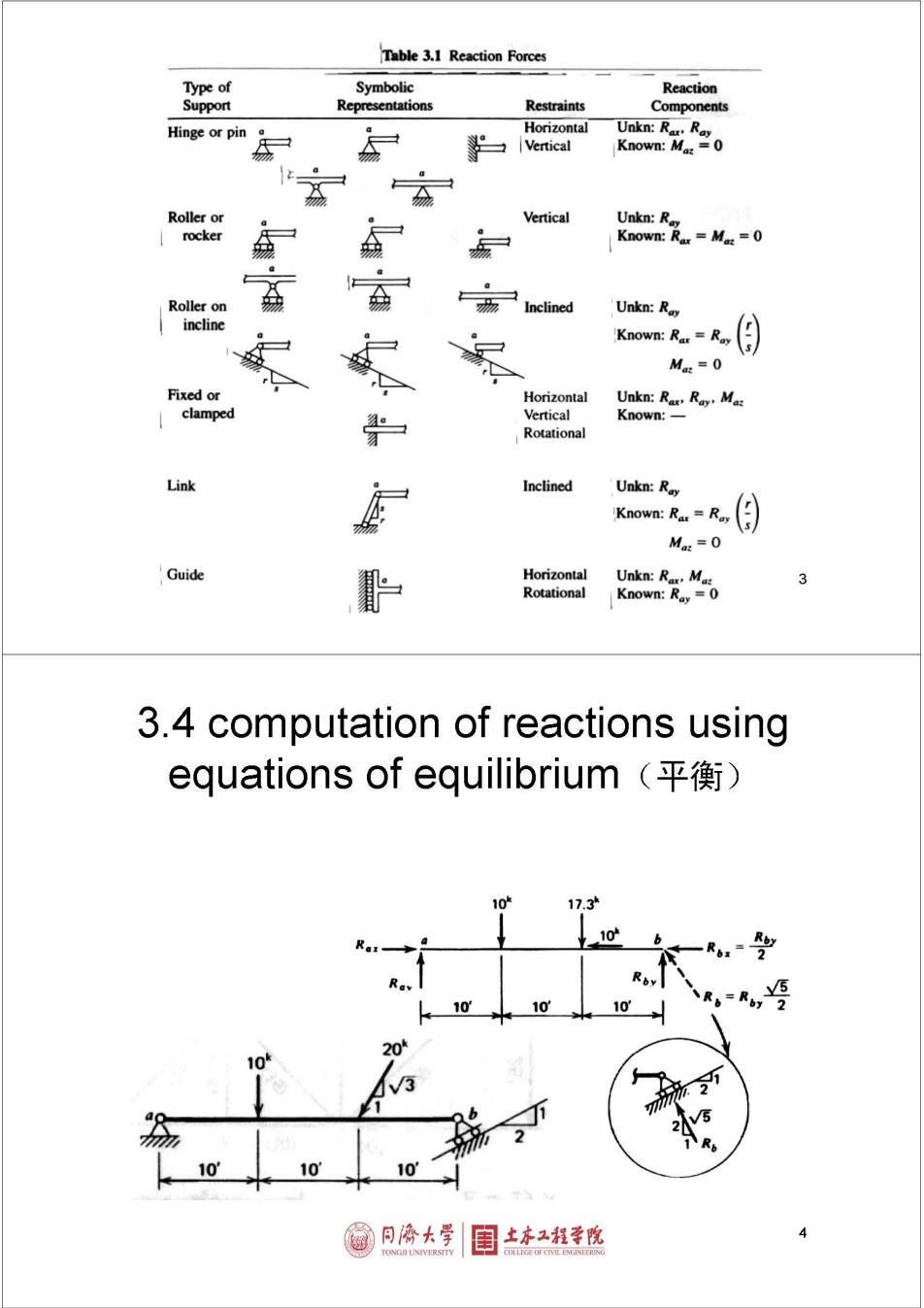
Table 3.1 Reaction Forces Type of Symbolic Reaction Support Representations Restraints Components Hinge or pin Horizontal y Unkn:Rar.Ray Vertical Known:Ma:=0 Roller or Vertical Unkn:Ray rocker Known:Rar Ma:=0 Roller on Inclined Unkn:Ray incline Known:Rar Ray Ma:=0 Fixed or Horizontal Unkn:Rar Ray:Mas clamped Vertical Known:- Rotational Link Inclined Unkn:Ray Known:Rar Ray Ma:=0 Guide 子 Horizontal Unkn:Rar.Ma: Rotational Known:Ray =0 3.4 computation of reactions using equations of equilibrium(平衡) 10 17.3 10 10 10 10 20 10 10 10 同©大学 土亦工程学院

3.5 condition equations for planar structures Indep. React. Numb.of Reg'd.React. Comps. Conditions Comps. Structure ra r=3+n Classification M.=0 4 1 3+1=4 r=r determinate, 6 stable M,=0。 编 12 13+2=5 ra=n determinate. stable 4 3+1=4 ra=r determinate, stable M,=0 15 11 3+1=4 ra>r indeterminate, stable 5 Indep. React. Numb.of Reg'd.React. Comps. Conditions Comps. Structure Ta r=3+n Classification TM=0 5 |2 3+2=5 ru=r. determinate, stable 4 2 3+2=5 r<r unstable M2=0 3 11 3+1=4 ra<r unstable 分 g M2=0:P=00 4 12 3+2=5 ra<r unstable 就 1 同份大子 工水二程子悦

Example Problem 3.4 150kN 120kN Hinge 14 3kN/m A C D 120m 20m Hinge 40m 20m 40m 20m 40m 120kN 90kN RD: 120 kN 3 kN/m 20m 20m 20m 20m 20m 20m 40m Example Problem 3.5 Entire structure 10 10 10 10 20 RB cs For the entire structure: For section BC: ∑M=0)+1020+40+60+80)-540) ∑M=0)+ 1020)+5(20)+Rc(40)-Ro40)=0 (condition equation) -Rg100)-Rc(20)=0 4Rg-4R。=30 Rcr 5Rcy =90 In summary,the equations of equilibrium are ∑P,=0tRw+Rg-4(10)=0 R。+5Rg=90 RA +Rcy=40 RAy +Rc=40 ∑P.=0R-Ro-5=0 Ru -Rcr =5 -4R6+4Rg=30 Ru-R。=5 同桥大学 土床工程学院
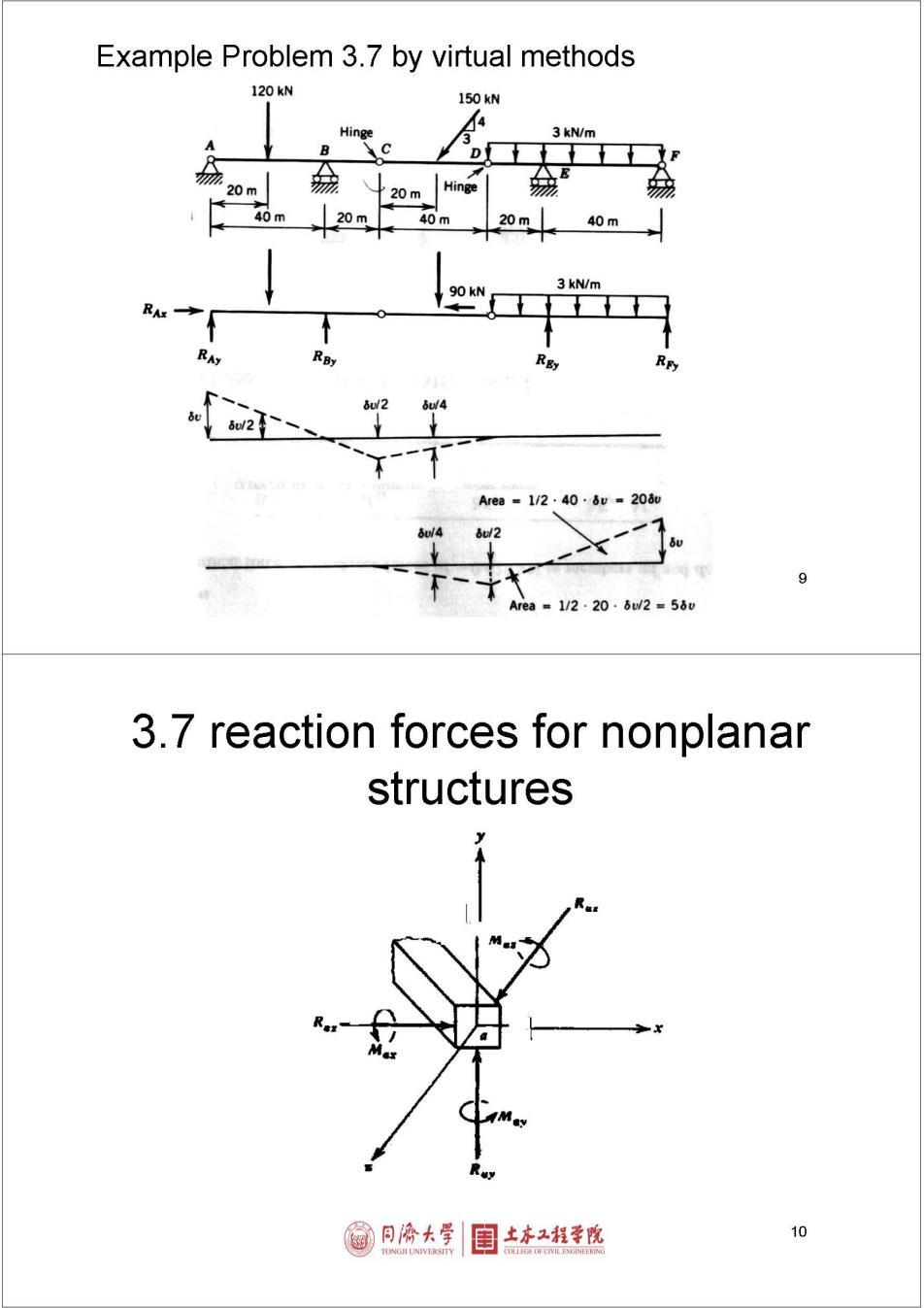
Example Problem 3.7 by virtual methods 120kN 150kN 4 Hinge 3 kN/m B 20m 20m Hinge 40m 20m 40m 20m 40m 90 kN 3 kN/m RF Area=1/2.40·6u=20加 9 Area=1/2·20.6uW2=56v 3.7 reaction forces for nonplanar structures 园何海大学 土本2程学院 10