
2016/4/6 Chapter 16 Dynamic analysis of structures @问济大学 目土本2程季悦 Why Dynamics? Statics vs.Dynamics F=ma @同冷大学 目士本红程学悦 1
2016/4/6 1 1 Chapter 16 Dynamic analysis of structures Why Dynamics? F ma Statics vs. Dynamics

2016/4/6 Contents 1 Introduction 2 Undamped free vibration(无阻尼自由振动)of one-degree systems 3 Undamped forced vibration(无阻尼强迫振动)of one-degree systems 4 Damped vibration(阻尼振动)of one degree systems 5 Free vibration(自由振动)of multi-.degree systems 6 Orthogonality(正交性)of normal modes 7 Forced vibration(受迫振动)of multi--degree systems 8 Free vibration of undamped(无阻尼)systems with distribution mass 9 Approximate procedures for determining circular frequency @问冷大学目土本2程季乾 16.1 Introduction ·Dynamic load(动荷载) Dynamic degree of freedom ·D'Alembert's principle(达朗贝尔原理 ·Dynamic equilibrium ·Equation of motion @同冷大学 目士本工相幸悦 2
2016/4/6 2 3 Undamped forced vibration(无阻尼强迫振动)of one-degree systems 1 Introduction 2 Undamped free vibration(无阻尼自由振动)of one-degree systems 4 Damped vibration(阻尼振动)of one degree systems 5 Free vibration(自由振动)of multi-degree systems 6 Orthogonality(正交性)of normal modes 7 Forced vibration(受迫振动)of multi-degree systems 8 Free vibration of undamped(无阻尼)systems with distribution mass 9 Approximate procedures for determining circular frequency Contents 16.1 Introduction • Dynamic load(动荷载) • Dynamic degree of freedom • D’Alembert’s principle(达朗贝尔原理 ) • Dynamic equilibrium • Equation of motion

2016/4/6 Dynamic load Periodic Unbalanced rotating machine (周期性) p(t) 2T。 3订。 Nonperiodic Bomb blast pressure-impulsive ( (非周期性) p(t) Earthquake-long duration 同桥大学 Dynamic degrees of freedom Number of dynamic DOF -the number of displacement components which must be considered in order to represent the effects of all significant inertial forces(惯性力)ofa structure m 1 DOF 2DOFs Infinite DOFs @同冷大学 3
2016/4/6 3 Dynamic load Periodic (周期性) Nonperiodic (非周期性) Unbalanced rotating machine Bomb blast pressure - impulsive(脉冲) Earthquake – long duration p t p(t) t o t po To To 2 To 3 p(t) t 1 DOF 2 DOFs m Infinite DOFs Dynamic degrees of freedom Number of dynamic DOF – the number of displacement components which must be considered in order to represent the effects of all significant inertial forces(惯性力)of a structure
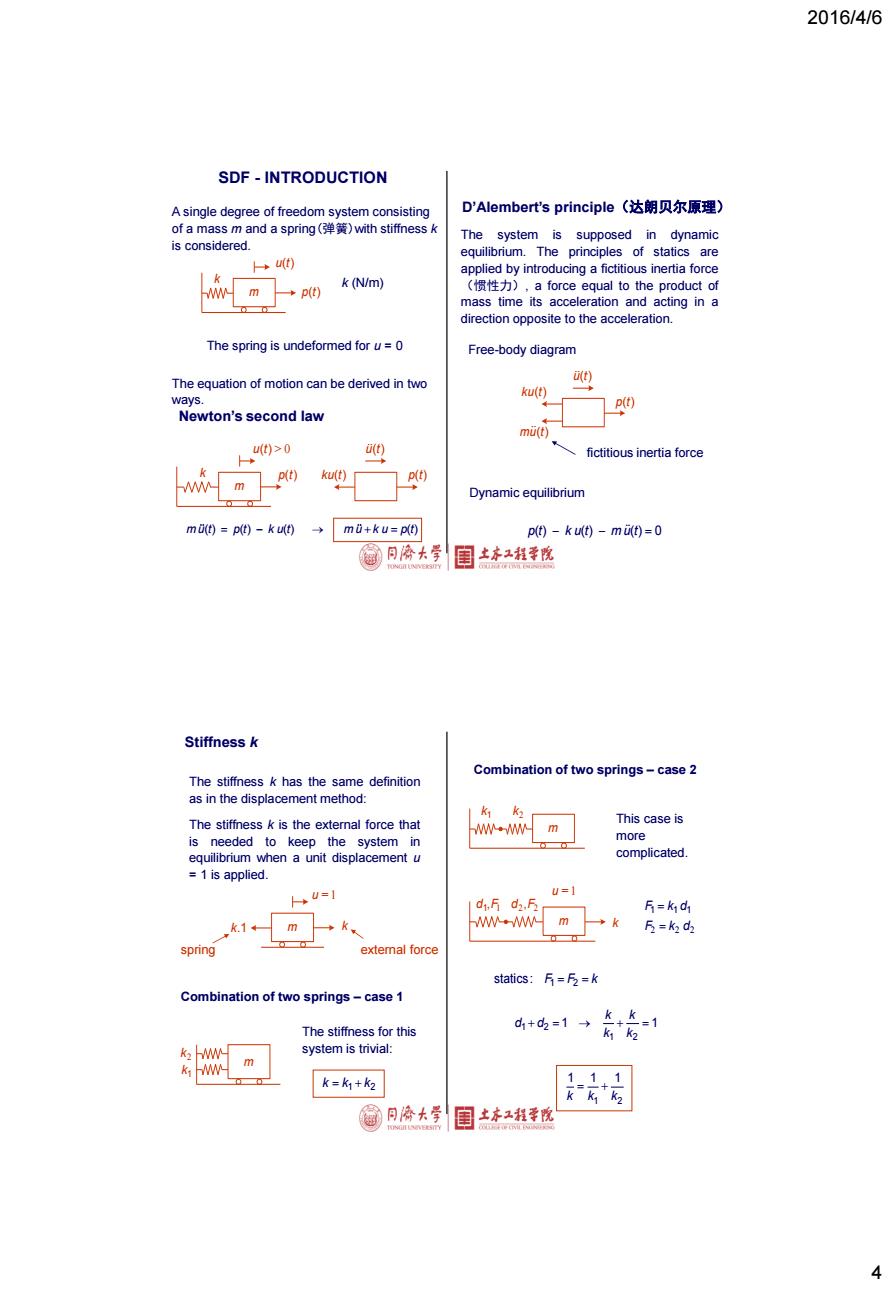
2016/4/6 SDF-INTRODUCTION A single degree of freedom system consisting D'Alembert's principle(达朗贝尔原理) of a mass m and a spring(弹簧)with stiffness k The system is supposed in dynamic is considered. equilibrium.The principles of statics are applied by introducing a fictitious inertia force k(N/m) m p(t) (惯性力),a force equal to the product of mass time its acceleration and acting in a direction opposite to the acceleration. The spring is undeformed for u=0 Free-body diagram The equation of motion can be derived in two i(t) ku(t) ways. Newton's second law mi(t) u(t)>0 i(t) fictitious inertia force o(t) ku(t) p(t) M Dynamic equilibrium m(t)=p(t)-ku(t) mu+ku=p(t) p(t)-ku(t)-mi(t)=0 目土永2程学院 Stiffness k Combination of two springs-case 2 The stiffness k has the same definition as in the displacement method: k The stiffness k is the extemnal force that This case is W is needed to keep the system in more equilibrium when a unit displacement u complicated. 1 is applied. U= U=1 di.F d2.F F=k d W m 5=k2d spring extemal force statics:F=F2=k Combination of two springs-case 1 The stiffness for this d1+d2=1→ kK=1 K2 FM system is trivial: 名m k=k+k2 11,1 k k3 K2 同橋大学 士本工相学院 4
2016/4/6 4 A single degree of freedom system consisting of a mass m and a spring(弹簧)with stiffness k is considered. SDF - INTRODUCTION The spring is undeformed for u = 0 k (N/m) Newton’s second law m u (t) p(t) k u(t) m u k u p(t) D’Alembert’s principle(达朗贝尔原理) p(t) k u(t) m u (t) 0 fictitious inertia force Dynamic equilibrium The equation of motion can be derived in two ways. The system is supposed in dynamic equilibrium. The principles of statics are applied by introducing a fictitious inertia force (惯性力), a force equal to the product of mass time its acceleration and acting in a direction opposite to the acceleration. Free-body diagram k m u(t) p(t) k m u(t) > 0 p(t) ku(t) p(t) u(t) p(t) ku(t) u(t) mu(t) Stiffness k The stiffness k has the same definition as in the displacement method: The stiffness k is the external force that is needed to keep the system in equilibrium when a unit displacement u = 1 is applied. Combination of two springs – case 1 The stiffness for this system is trivial: 1 2 k k k Combination of two springs – case 2 m k u = 1 k.1 1k m 2 k 1k m 2 k d F1 ,1 m u = 1 k d2 F2 , This case is more complicated. 2 2 d2 F k F k d 1 1 1 F F k 1 2 statics: 1 1 1 2 1 2 k k k k d d 1 2 1 1 1 k k k spring external force
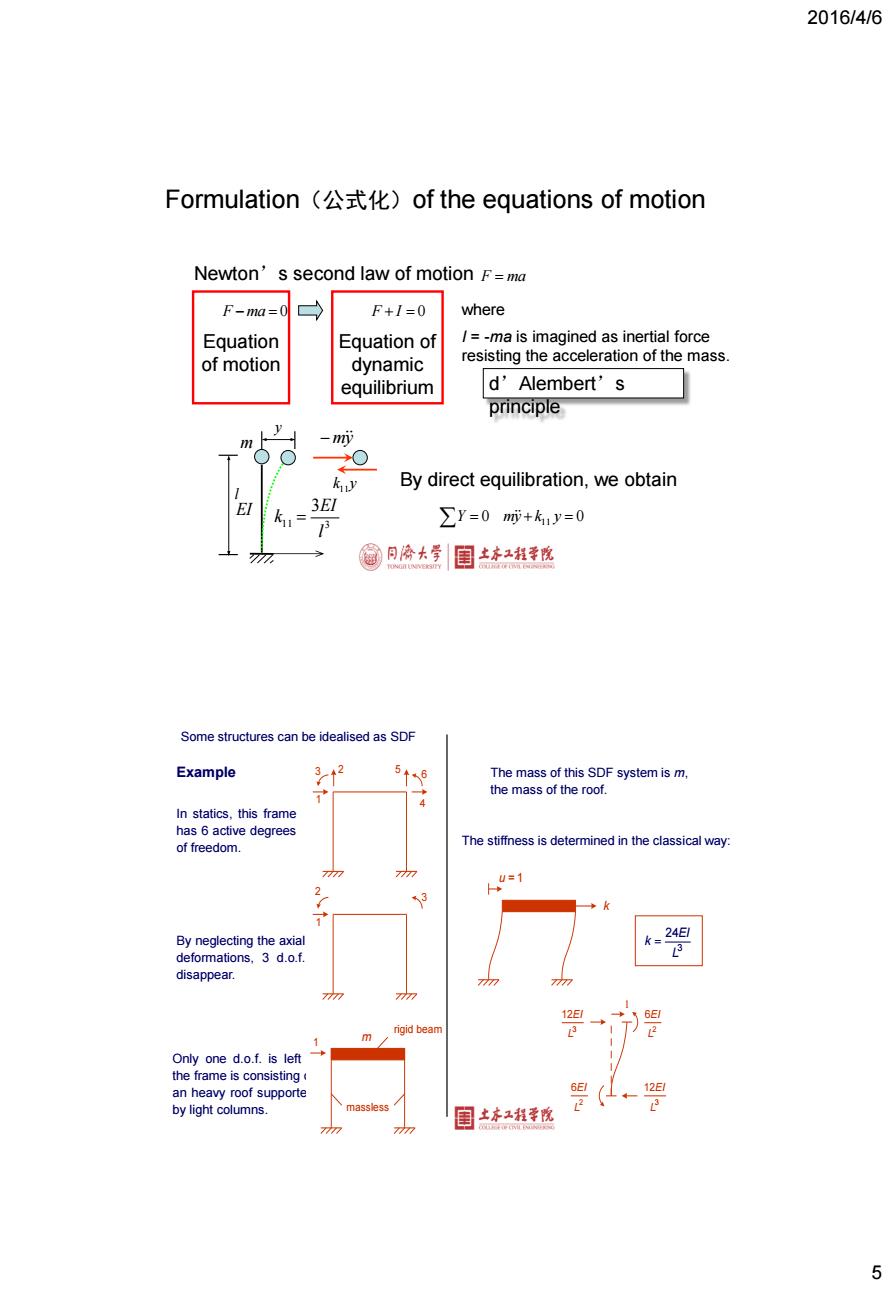
2016/4/6 Formulation(公式化)of the equations of motion Newton's second law of motion F=m F-ma=0 F+1=0 where Equation Equation of /=-ma is imagined as inertial force of motion dynamic resisting the acceleration of the mass. equilibrium d'Alembert's principle By direct equilibration,we obtain 3El 3 ∑Y=0m+k1y=0 同桥大学 士齐工程季晚 Some structures can be idealised as SDF Example 3 *2 The mass of this SDF system is m, the mass of the roof. In statics,this frame has 6 active degrees of freedom. The stiffness is determined in the classical way: 777 By neglecting the axial 24E k= deformations,3 d.o.f. 3 disappear 7770 7777 12E rigid beam Only one d.o.f.is left the frame is consisting an heavy roof supporte 6E 12E by light columns. massless 12 1土本工程学院 t导 5
2016/4/6 5 Equation of dynamic equilibrium Formulation(公式化)of the equations of motion Newton’s second law of motion F ma F ma 0 F I 0 I = -ma is imagined as inertial force resisting the acceleration of the mass. where d’Alembert’s principle Equation of motion m EI y l k y 11 m y 11 3 3 l EI k 0 0 Y m y k11 y By direct equilibration, we obtain Example 3 24 L EI k Only one d.o.f. is left if the frame is consisting of an heavy roof supported by light columns. 1 2 6 5 4 3 1 2 3 1 m rigid beam massless u = 1 k 1 3 12 L EI 2 6 L EI 3 12 L EI 2 6 L EI Some structures can be idealised as SDF In statics, this frame has 6 active degrees of freedom. By neglecting the axial deformations, 3 d.o.f. disappear. The mass of this SDF system is m, the mass of the roof. The stiffness is determined in the classical way: