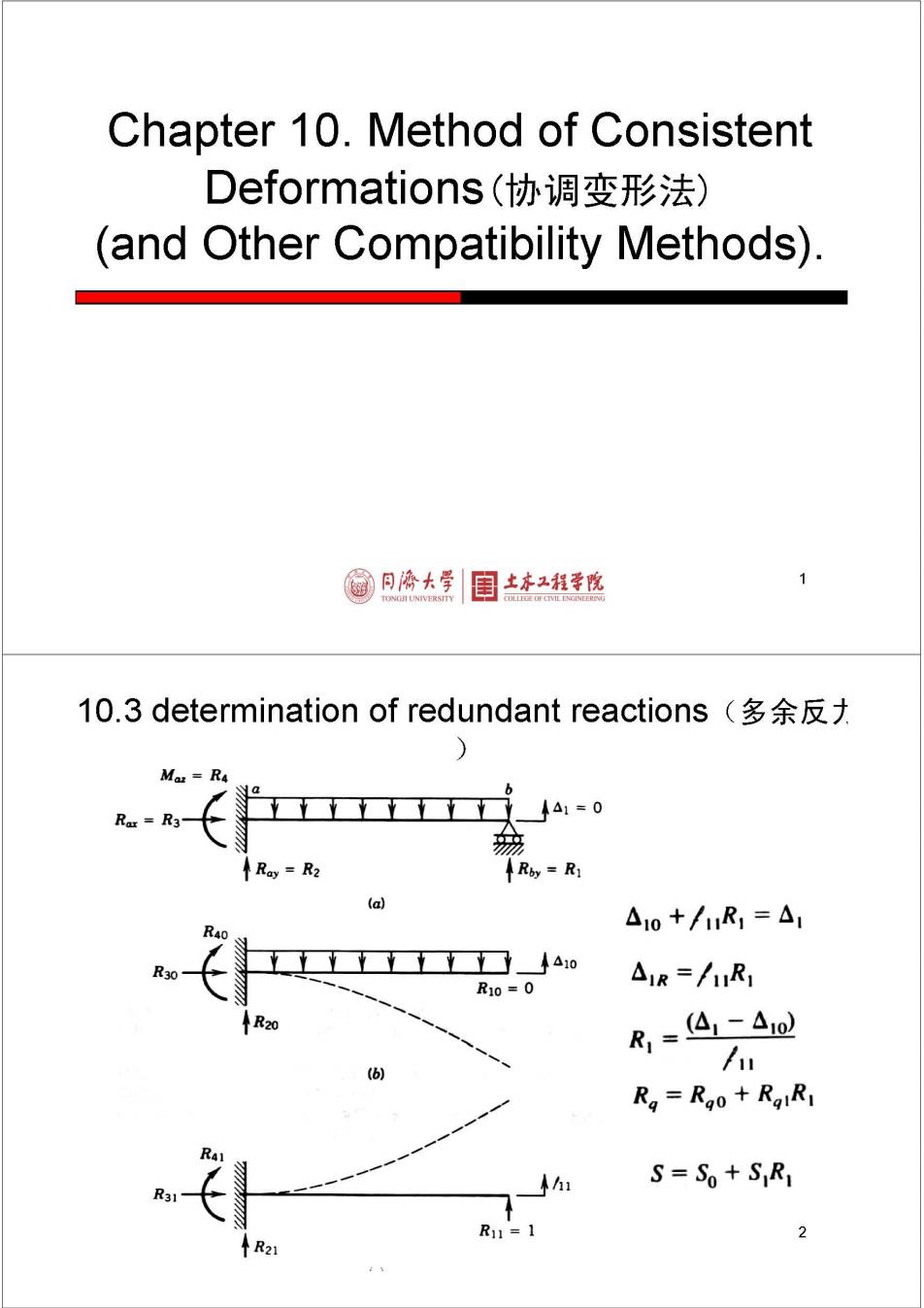
Chapter 10. Method of Consistent Deformations(协调变形法) (and Other Compatibility Methods). 月济大学 土工程学院 TONGJI UNIVERSITY 10.3 determination of redundant reactions( ) Ma=R4 Rax=R3 1=0 Ray=R2 Rby=Ri (a) R40 A10 +/11R1=A1 R30 410 R10=0 AIR=RI R20 R1=(A1-A10) (b) /u Rg=Ro + RaR R41 R31 hi S=S0+ SIR R11=1 2 R21
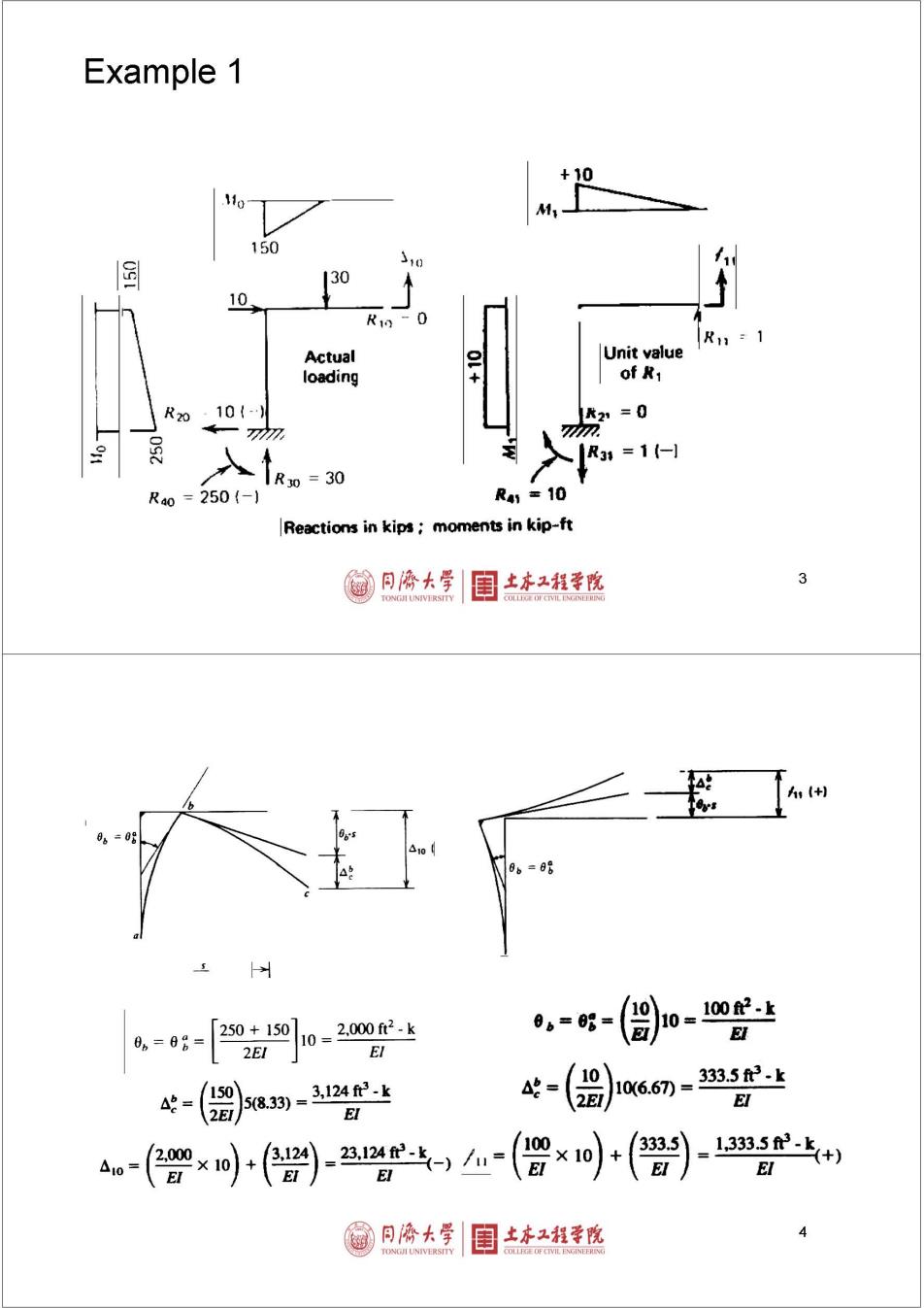
Example 1 +10 150 国 )10 30 10 R- 0 R:1 Actual Unit value loading of R1 R20·10(- 21=0 m 入R3=1- 1R0=30 R40=250(-J R41=10 Reactions in kips;moments in kip-ft 同©大学 土亦工程学院 06=88 06=08= 100.上 0。=08= 2,000f2.k El 4-(留324 A= 10 106.67= 33.53.k 4w-(智×+()-a2当-(智×0)+() 133350上+) El 同桥大学 土床工程学晚
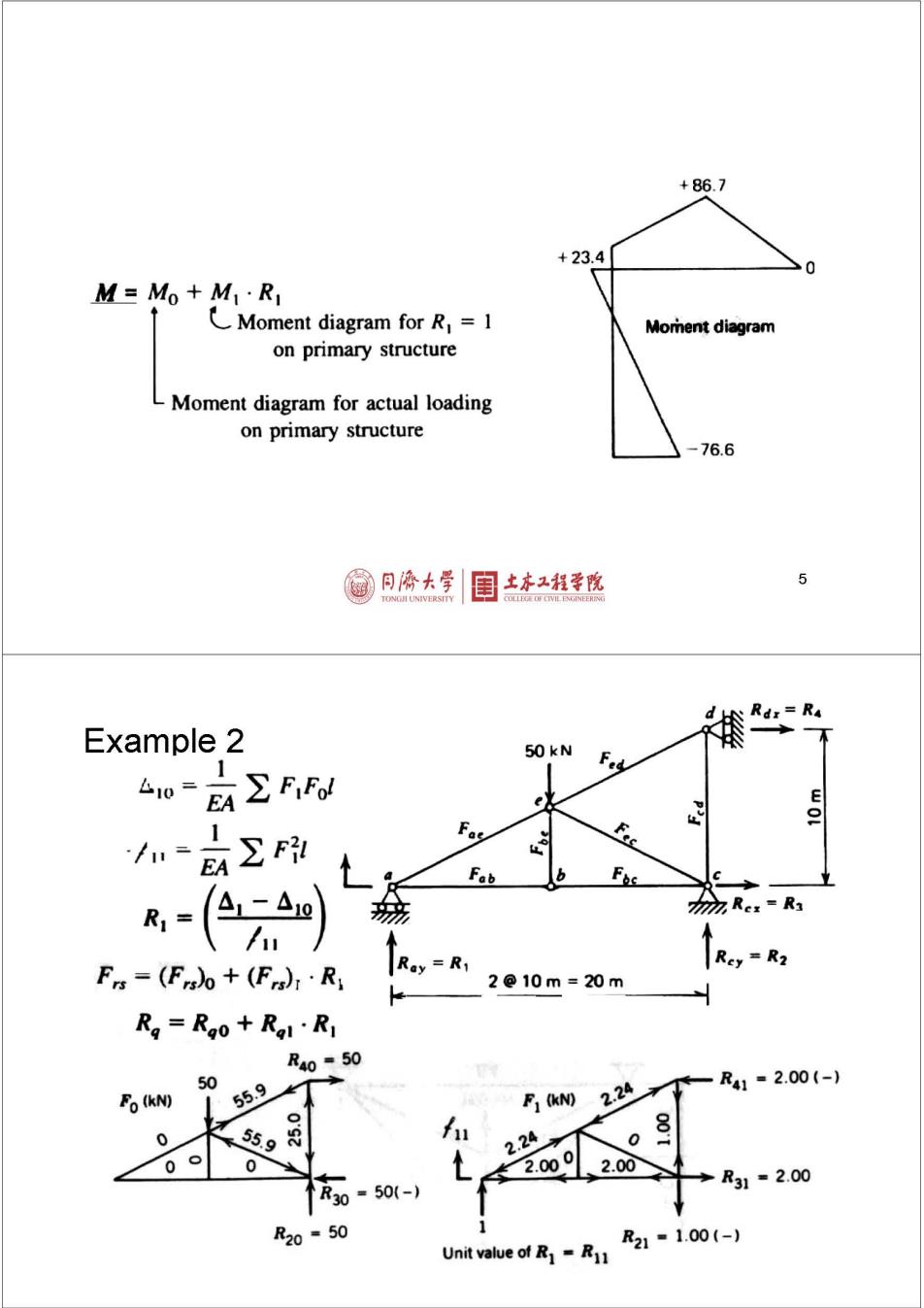
+86.7 +23.4 M=Mo+M1·R1 CMoment diagram for R=1 Moment diagram on primary structure Moment diagram for actual loading on primary structure -76.6 月大学 土亦工程学院 5 d Rd:=R4 Example 2 50kN 10 EA∑R Fab Rcx=R3 Rey=R2 Fs=(Fo+(F)·R, Ray =R1 2@10m=20m Rg=Rg0+Rg1·R1 R40=50 50 R41=2.00(-) Fo (kN) 55.9 月1kN2.24 8 0 2.24 0⊙ 0 2.000 2.00 R30=50(-) R31=2.00 R20=50 R21=1.00(-) Unit value of R=R1

10.5 support settlements(支座沉降) and elastic supports Chord connecting supports of primary structure 2 /11R1+/12R2=△1-△10 /2R1+/22R2=42-420 么-剑 +△20 同源大学 目±本赢nward settlements R1=k(-△) =- R,=V+1k) 同停大学 目 土亦工程学院

10.10 determination of redundant member forces for truss structures 了,bar forces (Fabl R1产 R21 (c) R31 △10+△1R=0 △1R=/1X S=So+SX1 同 9 Example 110= ΣR风 ∑ 53.3 。=0 Fo (kN) 75.4 378 3m.8 6.7 26.7 20.7 1R=80 R0=53.3 R0=287 707 a小1=X=1 (kN) 707 0 R1=0 R21=0 R31=0