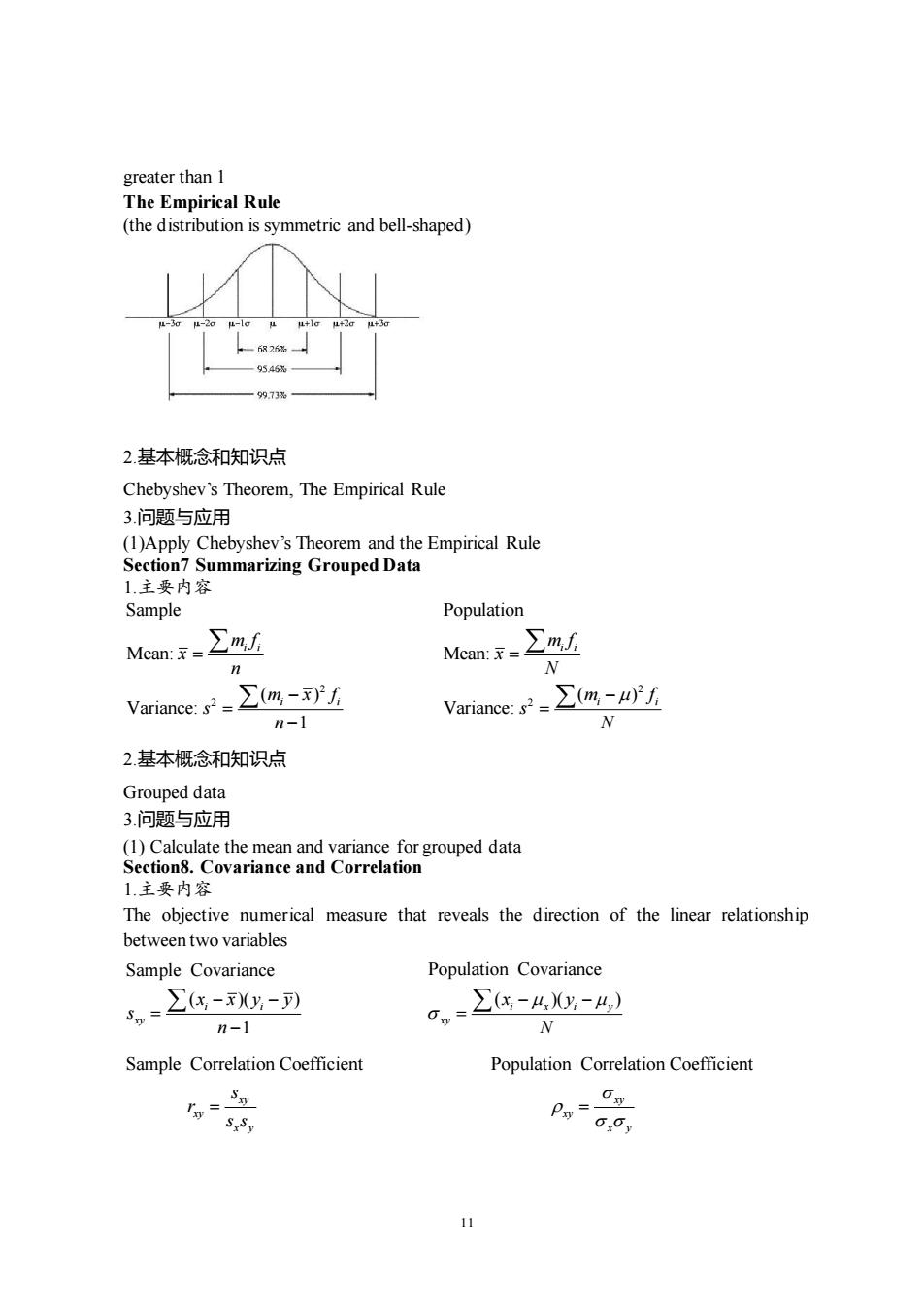
greater than I The Empirical Rule (the distribution is symmetric and bell-shaped) 7% 2基本概念和知识点 Chebyshev's Theorem,The Empirical Rule 3.问题与应用 (1)Apply Chebyshev's Theorem and the Empirical Rule Section7 Summarizing Grouped Data 1.主要内容 Sample Population Mean:r.∑以 Mean:F=∑mf N Variane:s-∑m-yi Varance:r=∑m-HPf n-1 N 2.基本概念和知识点 Grouped data 3.问题与应用 1.主要内容 The objective numerical measure that reveals the direction of the linear relationship between two variables Sample Covariance Population Covariance 与,=s-0,-刃 n- 0,=∑-40-4) Sample Correlation Coefficient Population Correlation Coefficient S Ps=Gy
11 greater than 1 The Empirical Rule (the distribution is symmetric and bell-shaped) 2.基本概念和知识点 Chebyshev’s Theorem, The Empirical Rule 3.问题与应用 (1)Apply Chebyshev’s Theorem and the Empirical Rule Section7 Summarizing Grouped Data 1.主要内容 2 2 Sample Mean: ( ) Variance: 1 i i i i m f x n m x f s n = − = − 2 2 Population Mean: ( ) Variance: i i i i m f x N m f s N = − = 2.基本概念和知识点 Grouped data 3.问题与应用 (1) Calculate the mean and variance for grouped data Section8. Covariance and Correlation 1.主要内容 The objective numerical measure that reveals the direction of the linear relationship between two variables Sample Covariance ( )( ) 1 i i xy x x y y s n − − = − Population Covariance ( )( ) i x i y xy x y N − − = Sample Correlation Coefficient xy xy x y s r s s = Population Correlation Coefficient xy xy x y =

2基本概念和知识点 Covariance.correlation coefficient 3.问题与应用 (1)Calculate and interpret the covariance and the correlation coefficient Case Analysis 课程思政: 1搜集与分析我国经济社会数据,描绘适当的统计图或统计表,结合数据域经济社会 相关政策,了解试试政治,研究中国问题,认识国情,激发爱国热情。 2.对数据进行哦统计分析时,充分运用所学方法,不歪曲数据特征,不误导读者,实 事求是而培养耐心细致的工作作风和严肃认真的工作态度。 (三)思考与实践 1.掌握书上本章概念(以绿色标出) 2.完成本章书后计算题 (四)教学方法与手段 1.教学方法:课堂讲授:课堂练习。 2.教学手段:多媒体教学。 Chapter 4 Introduction to Probability (一)目的与要求 1.Describe fundamental probability concepts. 2 Formulate and ex xplain subjective cal,and a prioi probabilities 3.Calculate and interpret the probability of the complement of an event,the probability tha at least one of two events will occur.and a joint probability. 4.Calculate and interpret a conditional probability. 5.Distinguish between independent and dependent events. 6.Calculate and interpret probabilities from a contingency table Apply the total pr rule and Bayes'theorem Useao to solve a particular counting problem. Section1.Fundamental Probability Concepts 1.主要内容 Events:a subset of the sample space Exhaustive events:all possible outcomes of a random experiment are included in the Mutually Exclusive events:do not share any common outcome of a random experiment Assigning probabilities (1)The probability of any event A is a value between 0 and 1,0s P(A)s 1. 12
12 2.基本概念和知识点 Covariance, correlation coefficient 3.问题与应用 (1) Calculate and interpret the covariance and the correlation coefficient Case Analysis 课程思政: 1.搜集与分析我国经济社会数据,描绘适当的统计图或统计表,结合数据域经济社会 相关政策,了解试试政治,研究中国问题,认识国情,激发爱国热情。 2.对数据进行哦统计分析时,充分运用所学方法,不歪曲数据特征,不误导读者,实 事求是而培养耐心细致的工作作风和严肃认真的工作态度。 (三)思考与实践 1.掌握书上本章概念(以绿色标出); 2.完成本章书后计算题。 (四)教学方法与手段 1.教学方法:课堂讲授;课堂练习。 2.教学手段:多媒体教学。 Chapter 4 Introduction to Probability (一)目的与要求 1. Describe fundamental probability concepts. 2. Formulate and explain subjective, empirical, and a priori probabilities. 3. Calculate and interpret the probability of the complement of an event, the probability that at least one of two events will occur, and a joint probability. 4. Calculate and interpret a conditional probability. 5. Distinguish between independent and dependent events. 6. Calculate and interpret probabilities from a contingency table. 7. Apply the total probability rule and Bayes’ theorem. 8. Use a counting rule to solve a particular counting problem. (二)教学内容 Section1. Fundamental Probability Concepts 1.主要内容 Events:a subset of the sample space Exhaustive events: all possible outcomes of a random experiment are included in the Mutually Exclusive events: do not share any common outcome of a random experiment Assigning probabilities (1)The probability of any event A is a value between 0 and 1, 0 ≤ P(A) ≤ 1

(2)The sum of the probabilities of any list of mutually exclusive and exhaustive events equals 1. ∑PX=1 Probabilities expressed as odds (1)Converting an odds ratio toa probability Given odds forevent Aoccurring of"a to b",the probability of A is+b Given oddsagainst event A occurring of"ato b,the probability ofA isb (2)Converting a probability to an oddsratio dene the prbby ofingPA)door P(A) The oddsforAoccurring equal 1-P() 1-PA) The oddsagainst Aoccurring equal P() 2.基本概念和知识点 Events,Exhaustive events,Mutually Exclusive events,assigning probabilities,Probabilities &odds 3.问题与应用 (1Describe fundamental probability ()Formulate and explain subjective,empirical,and a priori probabilities (3)Explain probabilities and odds Section2.Rules of Probability 1主要内容 The complement rule:P(4)=1-P() The addition rule:P(AUB)=P(A)+P(B)-P(ANB) P(AB)=P(A0B) P(BA)=P(A0B) Conditional probability: P(B) P(A) A an dependent P(A B)=P(A) P(BA)=P(B) The Multiplication Rule for Independent Events P(AOB)=P(A)P(B) 2.基本概念和知识点 13
13 (2)The sum of the probabilities of any list of mutually exclusive and exhaustive events equals 1. P X( ) 1= Probabilities expressed as odds (1)Converting an odds ratio to a probability Given odds for event A occurring of “a to b”, the probability of A is a a b + Given odds against event A occurring of “a to b”, the probability of A is b a b + (2)Converting a probability to an odds ratio If P(A) denotes the probability of an event A occurring, and P(A) does not equal zero or one, then: The odds for A occurring equal ( ) 1 ( ) P A − P A The odds against A occurring equal 1 ( ) ( ) P A P A − 2.基本概念和知识点 Events, Exhaustive events, Mutually Exclusive events, assigning probabilities, Probabilities & odds 3.问题与应用 (1) Describe fundamental probability concepts. (2) Formulate and explain subjective, empirical, and a priori probabilities (3) Explain probabilities and odds Section2. Rules of Probability 1.主要内容 The complement rule: P(A)=1-P(A C ) The addition rule: P(A∪B)=P(A)+P(B)-P(A∩B) Conditional probability: ( ) ( ) ( ) P A B P A B P B = , ( ) ( ) ( ) P A B P B A P A = Independent and dependent events A and B are independent ( ) ( ) ( ) ( ) P A B P A P B A P B = = The Multiplication Rule for Independent Events P A B P A P B ( ) ( ) ( ) = 2.基本概念和知识点

The the additio dependenttheucatn Rule for Independent Events rule, probability,independent and 3.问题与应用 (1)Calculate and interpret the probability of the complement of an event.the probability that at least one of two events will occur,and a joint probability. (2)Calculate and interpret a conditional probability (3)Distinguish between independent and dependent events Section3.Contingency Tables and Probabilities 1主要内容 nerally shows frequ ncies for two qualitative or categorical variables sive combination of x-y values. Empirical probabilities are easily calculated as the relative frequency of the occurrence of the event. 2.基本概念和知识点 Contingency table,relative frequency 3.问题与应用 1主要内容 The total probability ru P(A)=P(AOB)+P(AOB)P(A)=P(A B)P(B)+P(A B)P(B) Bayes'Theorem P(B4)= P(A0B) P(A B)P(B) P(A0B)+P(OB) P(B)=P(AB)P(B)+P(AB)P(B) 2基本概念和知识点 The total probability rule,Bayes'Theorem 3.问题与应用 (1)Apply the total probability rule and Bayes'theorem Section5.Counting Rules 1主要内空 )( The combination formula n n The permutation formula(Arrangement) 14
14 The complement rule, the addition rule, conditional probability, independent and dependent events, the Multiplication Rule for Independent Events 3.问题与应用 (1) Calculate and interpret the probability of the complement of an event, the probability that at least one of two events will occur, and a joint probability. (2) Calculate and interpret a conditional probability. (3) Distinguish between independent and dependent events. Section3. Contingency Tables and Probabilities 1.主要内容 A contingency table generally shows frequencies for two qualitative or categorical variables, x and y, where each cell represents a mutually exclusive combination of x-y values. Empirical probabilities are easily calculated as the relative frequency of the occurrence of the event. 2.基本概念和知识点 Contingency table, relative frequency 3.问题与应用 (1) Calculate and interpret probabilities from a contingency table. Section4. The Total Probability Rule and Bayes’ Theorem 1.主要内容 The total probability rule ( ) ( ) ( ) C P A P A B P A B = + , ( ) ( ) ( ) ( ) ( ) C C P A P A B P B P A B P B = + Bayes’ Theorem ( ) ( ) ( ) ( ) C P A B P B A P A B P A B = + , ( ) ( ) ( ) ( ) ( ) ( ) ( ) C C P A B P B P B A P A B P B P A B P B = + 2.基本概念和知识点 The total probability rule, Bayes’ Theorem 3.问题与应用 (1) Apply the total probability rule and Bayes’ theorem. Section5. Counting Rules 1.主要内容 The factorial formula n n n n n ! ( 1) ( 2) ( 3) 1 = − − − The combination formula ! ( )! ! n x n n C x n x x = = − The permutation formula (Arrangement)