Introduction Introduction In the context of linear system solution,the error in the solution constitutes a vector. r=b-A×X. If we do not want to pay attention to individual components of the error,perhaps because there are too many components, then we can combine all errors into a single number. This is akin to a grade point average which combines all grades into a single number. Mathematically,this "combining"is accomplished by norms. 命电有这女子 Matrix Theory Vector Norms -3/39
Introduction Introduction In the context of linear system solution, the error in the solution constitutes a vector. r = b − A × x. ▸ If we do not want to pay attention to individual components of the error, perhaps because there are too many components, then we can combine all errors into a single number. ▸ This is akin to a grade point average which combines all grades into a single number. ▸ Mathematically, this “combining” is accomplished by norms. Matrix Theory Vector Norms - 3/39
Introduction Introduction In the context of linear system solution,the error in the solution constitutes a vector. r =b-A xx. If we do not want to pay attention to individual components of the error,perhaps because there are too many components, then we can combine all errors into a single number. This is akin to a grade point average which combines all grades into a single number. Mathematically,this "combining"is accomplished by norms. Start with vector norms,measuring the length of a vector. 色老有头习 Matrix Theory Vector Norms -3/39
Introduction Introduction In the context of linear system solution, the error in the solution constitutes a vector. r = b − A × x. ▸ If we do not want to pay attention to individual components of the error, perhaps because there are too many components, then we can combine all errors into a single number. ▸ This is akin to a grade point average which combines all grades into a single number. ▸ Mathematically, this “combining” is accomplished by norms. Start with vector norms, measuring the length of a vector. Matrix Theory Vector Norms - 3/39
Definition Outline Introduction Definition Two Important Inequalities Exercises Normwise Errors Comprehensive Problems Hold Inequality again 奇老有这女子 Matrix Theory Vector Norms -4/39
Definition Outline Introduction Definition Two Important Inequalities Exercises Normwise Errors Comprehensive Problems H¨old Inequality again Matrix Theory Vector Norms - 4/39

Definition Definition A vector norm is a function from Cn to R with three properties: 命电有这女子 Matrix Theory Vector Norms -5/39
Definition Definition A vector norm ∣∣ ⋅ ∣∣ is a function from C n to R with three properties: Matrix Theory Vector Norms - 5/39
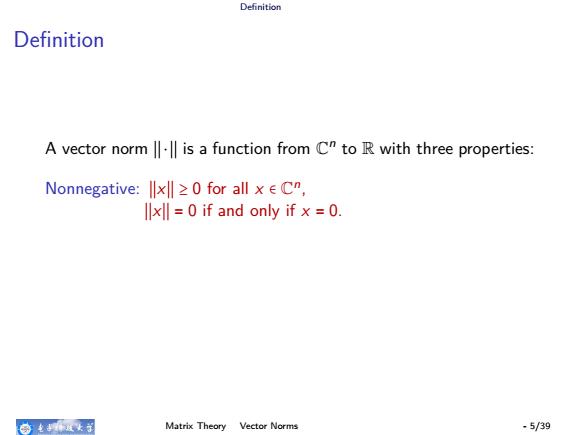
Definition Definition A vector norm is a function from Ch to R with three properties: Nonnegative:llxl≥0 for all x∈Cn, xI=0 if and only if x =0. 命电有这女子 Matrix Theory Vector Norms -5/39
Definition Definition A vector norm ∣∣ ⋅ ∣∣ is a function from C n to R with three properties: Nonnegative: ∣∣x∣∣ ≥ 0 for all x ∈ C n , ∣∣x∣∣ = 0 if and only if x = 0. Homogeneous: ∣∣αx∣∣ = ∣α∣∣∣x∣∣ for all α ∈ C, x ∈ C n . Triangle inequality: ∣∣x + y∣∣ ≤ ∣∣x∣∣ + ∣∣y∣∣ for all x, y ∈ C n . Matrix Theory Vector Norms - 5/39