
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters X~P(A) X~E() OX心R(,02 0XR(0.0) f)= (0+1)x”,0<x<1 0 else 4口t3,t3元,王000 Xiaohan Yang Chapter 10 Point Estimation
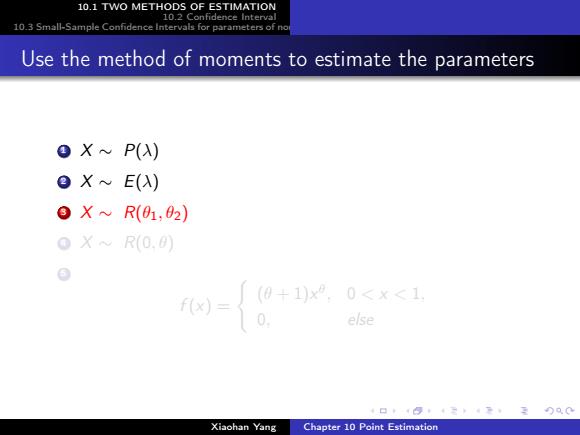
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interva 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters X~P(A) 9X~E() 0X~R(0,02) 0XR(0.0 (0+1Dx”0<x<1 0 else 4日·5,421手,3000 Xiaohan Yang Chapter 10 Point Estimation
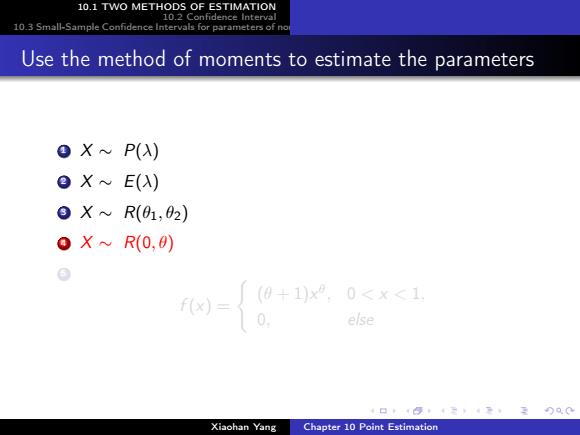
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters ④X~P(A) X~E(A) ⊙X~R(01,2) 0X~R(0,) f(x)= (0+1)x”,0<x<1 0 else 4口13,48)4元,3000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation