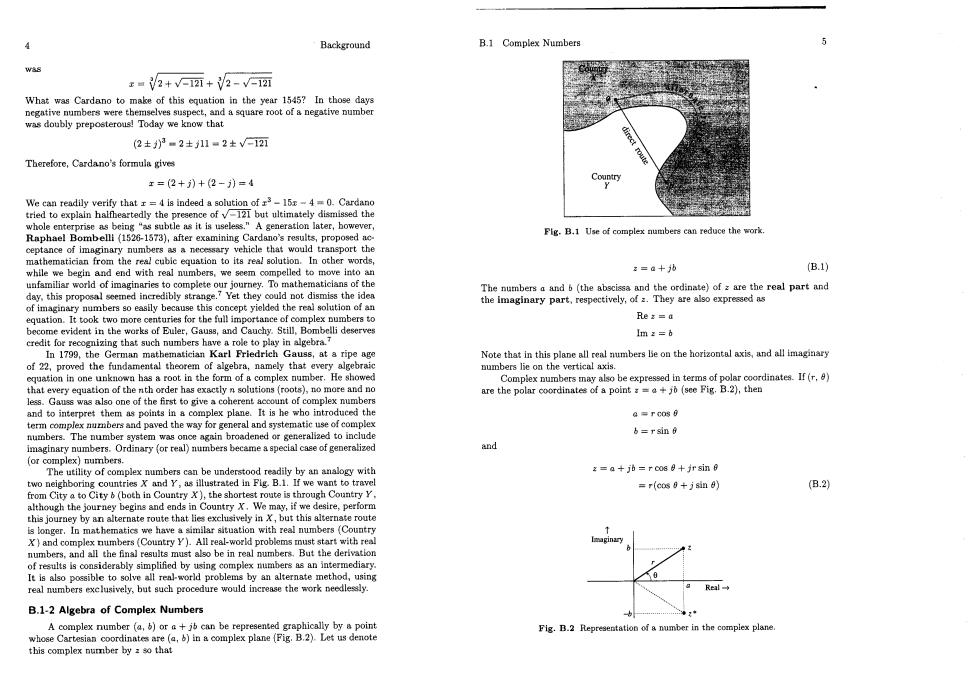
4 Background B.1 Complex Numbers x■2+12i+2-V-12 What was Cardano to make of this equation in the year 1545?In those days negative numbers were themselves suspect,and a square root of a negative number was doubly preposterous!Today we know that (2±)3■2±11=2±V-121 Therefore,Cardano's formula gives x=(2++2-)=4 Coupiry We ean readily verify thatr=4 is indeed a solution of -15x-4=0.Cardano tried to explain halfheartedly the presence of-121 but ultimately dismissed the whole enterprise as being "as subtle as it is useless." A generation later,bowever, Raphael Bombelli(1526-1573),after examining Cardano's resuts Fig.B.1 Use of complex numbers can reduce the work proposed ceptance of imaginary numbers asa vehicle tha spo mathematician from the real cubic equation to its real solu ords :=a+jb B.1) while we地ga end with real numbers,we scem co 6。 day,this The numbers a and b(the abscissa and the ordinate)of z are the real part and ly stra ge is the imaginary part,respectively,of :They are also expressed as he Re:=a lent in Still.Bomt elli des Im 2=6 h rale to 1799 ian Karl Friedrich Gauss.at a rip Note that in this planeal realnmbers eon the horizontaaxsnd almg of 22, the tal theore namely that every alg tical axis oot in the for of g complex number.He showed also be expressed in terms of polar coordinates.If(r) that ev of the ath order has exactly n solutions (roots),no more and no =+j(see Fig.B.2),then af the first to aiv a coherent account of complex numbers and to inte plex plane it is he who introducedi the ter com ved the way for eneral and systematic use of complex mbers.The number system was once again broadened or generalized to include b=rsin imaginary numbers.Ordinary for real)numbers became a special case of generalized and (or cor The utility of complex numbers can be understood readily by an anslogy with two neighboring countries X and Y,as illustrated in Fig.B.1.If we want to travel =r(cos 8+jsin 0) (B.2) from City a to City(both in Country X),the shortest route is through Country Y, although the journey begins and ends in Country X.We may,if we desire,perform this journey by an alternate route that lies exclsively in X,but this alternate route is longer,In mathematics we have a similar situation with real numbers (Country X)and complex numbers (Country Y).All real-world problems must start with rea numbers,and all the final results must also be in real numbers.But the derivatlon of results is considerably simplified by using complex numbers as an intermediary. It is also possible to solve all real-world problems by an alte rnate meth real numbers exclusively,but such procedure would increase theorkeedy. B.1-2 Algebra of Complex Numbers A complex number (a,5)or a+jb can be represented graphically by a point Fig.B.2 number in the cpex plane. whose Cartesian coordinates are (a,b)in a complex plane (Fig.B.2).Let us denote this complex number by so that
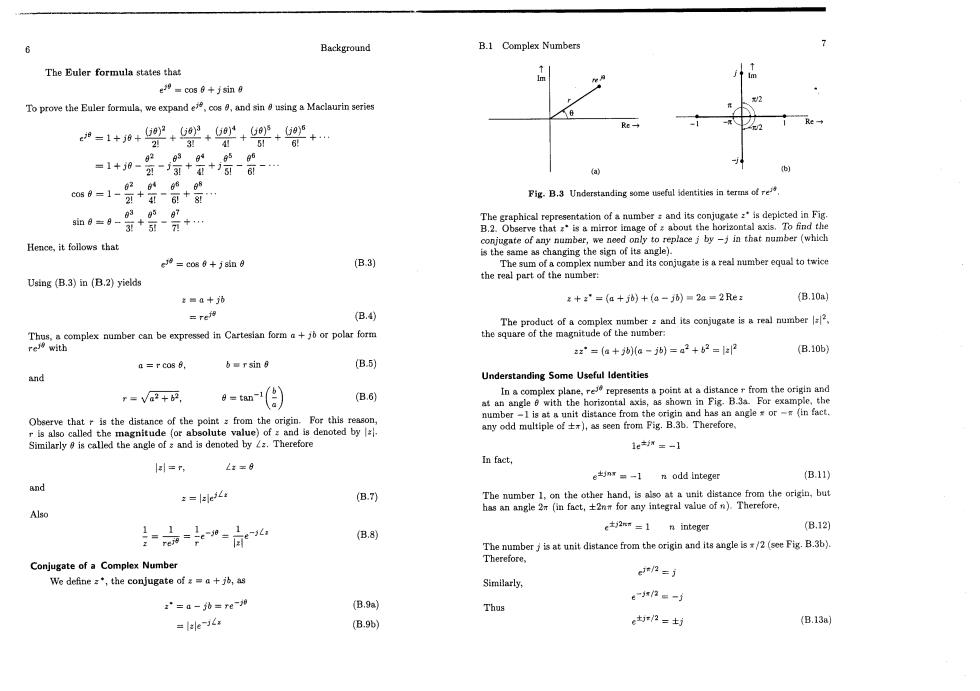
Background B.1 Complex Numbers The Euler formula states that cos 6+j sin 8 To prove the Euler formula,we expand and sin usingaMaclaurin series 9=1+0+++0+++. Re 5 =1+0-的的 2 31 6 Pig.B.3 useful identities in term 8=-+智-+. The graphical representation of a number s and its conjugate is depicted in Fig. B.2 Observe that ais a mirror image of about the horizontal axis.To find the Hence,it follows that ace8om的aej切-nthat b(dh 1=eos 6+j sin (B3) The sum of a complex number and its conjugate is a real number equal to twice the real part of the number: Using (B.3)in (B.2)yields :■a+市 ¥+”=(a+j)+(a-=2a=2Rez B,10 =refe (B4) The product of a complex number and its conjugate isa real number a complex number can be expressed in Cartesian form+or polar form the square of the magnitude of the number: z*=(a+)(a-j)=a2+2= (B.10b) a =rcos. bursin (B5) and Understanding Some Useful ldentities 。=m() aint at a distance r from the origin and r=√a2+2 (B.6 n in Fig.B.3a For example,the l is and has Observe that is the distance of the For this it dis ce from the origin as seen from Fig.B.3b.Therefore, an angle or(in fact. r is also calle时 1em=-1 In fact, =r, L:=8 e=-1 n odd integer (B.11 and :=leilr (B7) The number 1.on the other hand,isalsoata unit distance from the orign,bu Also has an angle 2(in fact,+2nm for any integral value of n).Therefore, 生=”=高 (8.12) (B8 e+2m=1 n intege时 The numberis at unit distance from the origin and its angle is/(se Fig.B.3b) Conjugate of a Complex Number Theretore, eltp2=j We define 'the conjugate of =a+jb,as Similarly, (B,9a) e2=-寸 *=a-fb=re Thus =lzle-J4: (B.96 e2=打 (B.13a)
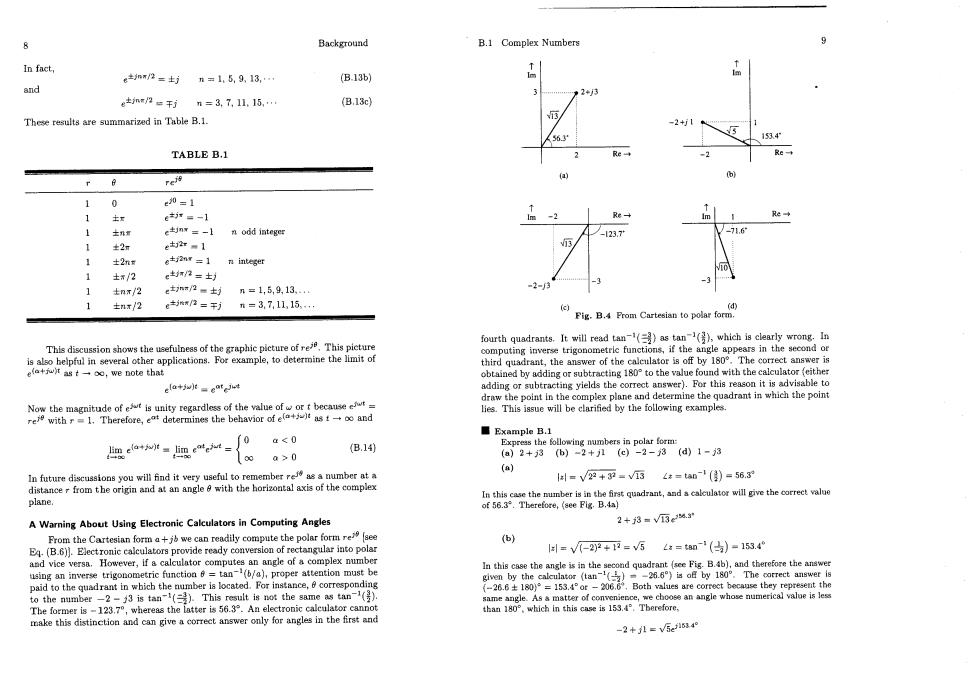
8 Background B.1 Complex Numbers In fact enm/2=jn=1.5,9,13,: (B.13b) and 3 en2=jn=3.7,11.15, (B,13c) These results are summarized in Table B.1. 63 TABLE B.1 1 0 e0=1 1 士x em=-1 Re- Re 1 士升智 e封n=-1 n odd integer -1237 1 ±2m e2r。1 1 土2n 2nr=1 n integer 1 土x/2 e封/2=封 -3 emm/2-士封n=1,5,9,13 2-3 士nT/2 1 土nx/2 ew/2=干jn=3,7,11,15,. Pig.B.Pom are fourth quadrants.It will read tan-1()as tan(),which is clearly This disc ssion shov computing inverse trigonome g the answer of the 80 t answer is g180 ith the calculator ela+jult cat lt ting yields the ason it is advisable to 线he magnitude of csoe is anity regardi恶Eee ines the behavior of e()as too and ■Example B.l mea*g=▣ee= 0 a<0 (B.14) es the following numbers in polar form oo a>0 (a)2+3(b)-2+1(c)-2-3(d)1-3 In future discussions you will find it very useful to rememberas a number at a (a) 1l=V22+32=v3tg=tan'()=56,3 distancer from the origin and at an angle with the horizontal axis of the complex plane. .Therefore,(see Fig.B.4a) A Warning About Using Electronic Calculators in Computing Angles 2+8=e4.3 From the Cartesian f 6 l=V0-2p+=v5a=1()-153.4 if a omputes an angle of a complex number 6=tan-(b/a) oper attention must be t in wbich the number is located.For instance,corresponding 26 3 is tan-1(=3).This result is not the same as tan1() Ass matter of conveniece,we choose an angle whose numerical value is less ie -123.7° eas the latter is 56.3.An electronic caleulator cannot than18o°.which in this case153.d°,Therefore,. ake this distinction and can only for angles in the 2+=V5e4
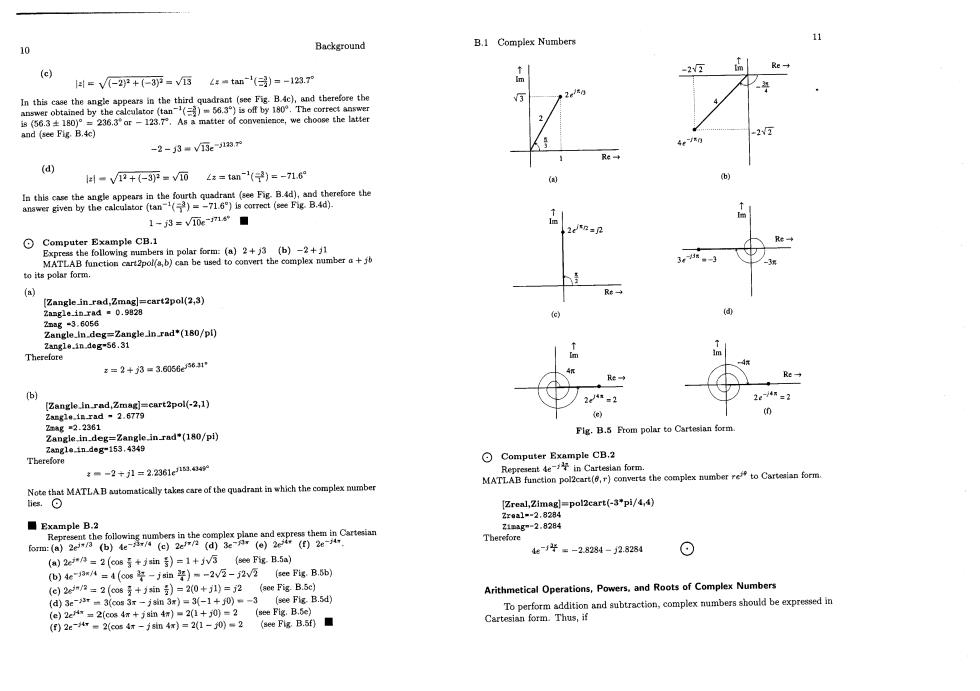
10 Background B.1 Complex Numbers (e) -2 Re- =V-2驴+(-3那=v13 t=tan()=-123.7 answer obtained by B.4c) 2 -2-3V3e1路,m (d) 川-V+(仁3∬=o4a=tan-(学)=-71.6° (b answer given by the calculator (tan(=- 1-j3=04-1m8”■ Re umbers in polar focm:(a)2+j3 (b)-+1 MATLAB function pol(a,b)can be used to oovert the complex number 3。-3 to its polar form. -cart2pol(2,3) 2ag-3.6056 Zangle_in deg=Zanglein_rad*(180/pl) 2ang18.1nd0g56.31 Therefore ¥=2+3=3.6056e361 RE→ (b) 2e=2 [Zanglein_rad,Zmag]=cart2pol(-2,1) 242 (e) =Zangle in-rad*(180/pi) Fig.B.5 From polar to Cartesian form. g11ndg153.4349 ¥=-2+1=2,2361e1a.44 convt the compex mumber re to Cartean fo NMATLAD omaicalyt care of the quadramt in which the coplex uber ocart(-3/4.) 282 ■Example B.2 g-2.8284 Therefore 4e-f4=-28284-2.3284 (a)2e=(c0sjsin=1+(see Fig.B.5a) ⊙ (b)4e-04=4(os¥-jsin)-2Va-2W2(eFgB.b (c)2en/2=2(co6号+1n》=20+11)=2. Arithmetical Operations,Powers.and Roots of Complex Numbers (d)3c-r=3(o3x-i如3x)=3-1+f0)=-3 B.5d) (e)22=2(cos 4x jsin 4)(+j)=2 (see Fig:B. ()2e-r=2(eos4r-1n4x}=2(1-0)=2 (see Fig B.5f)■ Cartesian form.Thus,i

Background B.1 Complex Numbers 13 4=3+4m5e59,1 and 兽-=滑-+品82持 then 22+3=V1336.3° Division:Polar Form 1+9=(3+j4)+(2+j3)=5+7 3531# given in polar form,we would need to convert them into Carte sian form fo uh2nd vision, 山方wpn this example that multiplication and division however,can be or pola o n the latte plish in polar form than in Cartesian form. =r1c ■Example B.4 and =raejda For =2e/4 and za8e/find (m)2z-z (b)(e)(d) then (a)Since subtraction cannot be performed directly in plafor,we cerand 2=(r1e3)(r2e)=rnre9+8 (B.15a to Cartesian form: and n=2e"=2(c0e吾+fn)=v2+② (B.15b) a8e3=8(c0sjsi)=4+jv3 Therefore Moreover, =(reie)"=reine (B.15c) 2-扫=2v2+V2)-(4+4v③ and sin(rein=rimn (B.15d) =(2v2-4)+2v2-4v3 =-1.17-j41 This shows that the operations of multiplication.division,powers,and roots can be carried out with remarkable ease when the numbers are in polar form. 3 ■Example B.3 言=-心 21■3+34=5231 寻==动-动 2=2+j沿=V155a (d) =3=(8c/是=8时(e/=2ar%■ Weshall ove this problem in both por and Cartesanform Multiplication:Cartesian Form Computer Example CB.3 212=3+j40(2+3)=(6-12)+(8+=-6+j17 2a2+ Multiplication:Polar Form 1=8+j42=2+j*3 g1221*3 a-(6ea)(Besr)=5ve1oo z1z2-6.000+17.00001 sl_over_x2=21/22 Division:Cartesian Form z1.070rz2-1.3488-0.07691 Therefore In order to eliminate the complex number in the denominator,we multlply both (3+4)2+j3)=-6+178d3+f4/2+3)=1.3486-0.0789 ⊙ the numerator and the denominator of the right-hand slde by 2-f3,the denominator's conjugate.This yields