
二、多元线性回归模型的基本假设
二、多元线性回归模型的基本假设
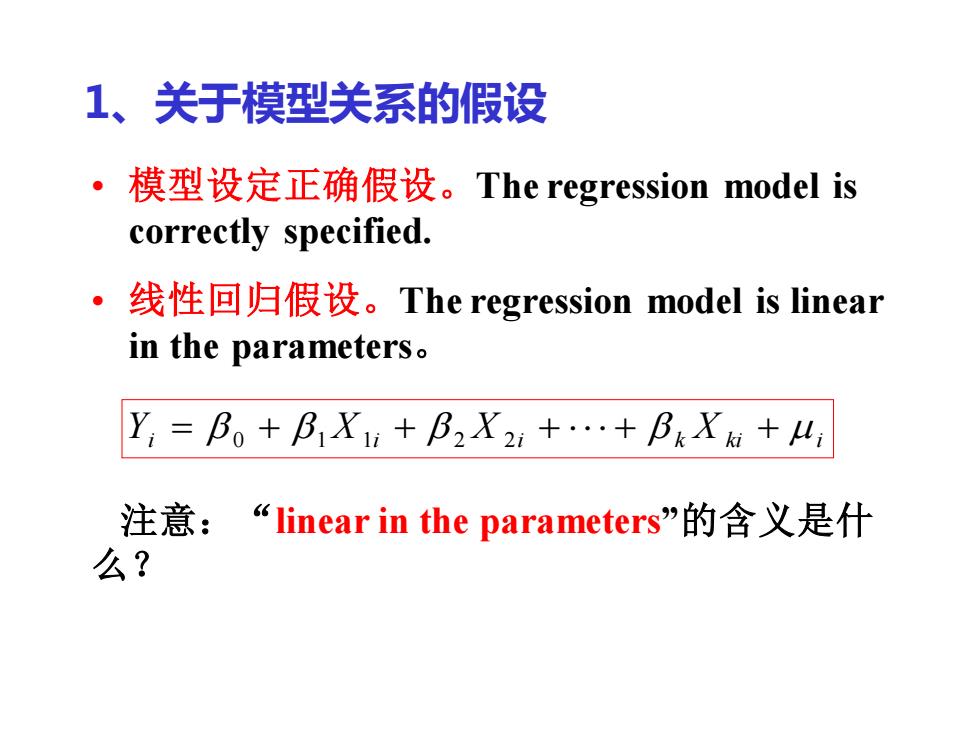
1、关于模型关系的假设 ·模型设定正确假设。The regression model is correctly specified. ·线性▣归假设。The regression model is linear in the parameters. Y,=B。+BXi+B2X2,+.+BkXa+4 注意:“linear in the parameters'”的含义是什 么?
1、关于模型关系的假设 • 模型设定正确假设。The regression model is correctly specified. • 线性回归假设。The regression model is linear in the parameters。 注意:“linear in the parameters”的含义是什 么? Yi X i X i + k X ki + i = + + + 0 1 1 2 2

2、关于解释变量的假设 ·确定性假设。X values are fixed in repeated sampling.More technically,X is assumed to be nonstochastic. 注意:“in repeated sampling'”的含义是什么? ·与随机项不相关假设。The covariances between Xi and ui are zero. c0V(X,4)=0,i=1,2,.,n E(X4)=0,i=1,2,.,n 由确定性假设可以推断
2、关于解释变量的假设 • 确定性假设。X values are fixed in repeated sampling. More technically, X is assumed to be nonstochastic. 注意:“in repeated sampling”的含义是什么? • 与随机项不相关假设。The covariances between Xi and μi are zero. 由确定性假设可以推断。 cov( , ) 0, 1,2, , ( ) 0, 1,2, , i i i i X i n E X i n = = = =

0) 观测值变化假设。X values in a given sample must not all be the same. 无完全共线性假设。There is no perfect multicollinearity among the explanatory variables. 时间序列数据作 适用于多元线性回归模型。 样本时间适用 ·样本方差假设。随着样本容量的无限增加,解 释变量X的样本方差趋于一有限常数。 ∑(x,-)2/n0 n→oo
• 观测值变化假设。X values in a given sample must not all be the same. • 无完全共线性假设。There is no perfect multicollinearity among the explanatory variables. 适用于多元线性回归模型。 • 样本方差假设。随着样本容量的无限增加,解 释变量X的样本方差趋于一有限常数。 (Xi − X) / n →Q, n → 2 时间序列数据作 样本时间适用

3、关于随机项的假设 。 0均值假设。The conditional mean value of:is zero. E(4X)=0,i=1,2,.,n 由模型设定正确假设推断。 ·同方差假设。The conditional variances of i are identical.(Homoscedasticity) amr(4X,)=o2,i=1,2,.,n 是否满足需要检验
3、关于随机项的假设 • 0均值假设。The conditional mean value of μi is zero. • 同方差假设。The conditional variances of μi are identical.(Homoscedasticity) 由模型设定正确假设推断。 ( ) 0, 1,2, , E X i n i i = = 2 ( ) , 1,2, , Var X i n i i = = 是否满足需要检验