
Asset Return Portfolio Return Suppose now that n different assets are available.We can form a master asset,or portfolio,of these n assets. Suppose that this is done by apportioning a total amount Xo among the n assets.We then select amounts Xoi,i=1,2,...,n,such that 1oi=Xo.where Xoi represents the amount invested in the ith asset. If we are allowed to sell an asset short,then some of the Xoi's can be negative;otherwise we restrict the Xoi's to be nonnegative. The amounts invested can be expressed as fractions of Xo,i.e., Xoi wiXo,i=1,2,...,n, ∑%=1, i=1 where wi is the weight or fraction of asset i in the portfolio. )Q0 Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 7/81
Asset Return Portfolio Return Suppose now that n different assets are available. We can form a master asset, or portfolio, of these n assets. Suppose that this is done by apportioning a total amount X0 among the n assets. We then select amounts X0i P , i = 1, 2, . . . , n, such that n i=1 X0i = X0, where X0i represents the amount invested in the ith asset. If we are allowed to sell an asset short, then some of the X0i ’s can be negative; otherwise we restrict the X0i ’s to be nonnegative. The amounts invested can be expressed as fractions of X0, i.e., X0i = wiX0, i = 1, 2, . . . , n, Xn i=1 wi = 1, where wi is the weight or fraction of asset i in the portfolio. Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 7 / 81
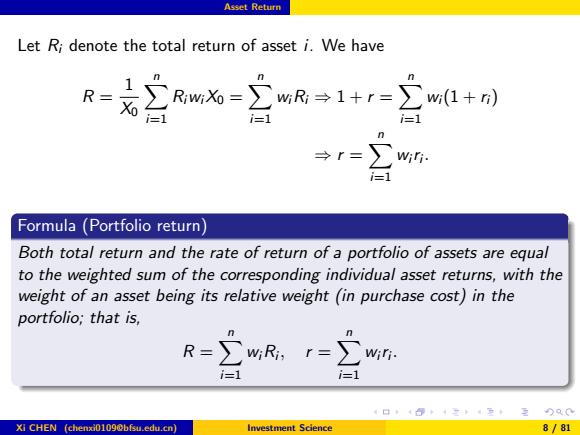
Asset Return Let R;denote the total return of asset i.We have R=无2Rw必=2R1+1-1+月 i=1 Formula(Portfolio return) Both total return and the rate of return of a portfolio of assets are equal to the weighted sum of the corresponding individual asset returns,with the weight of an asset being its relative weight(in purchase cost)in the portfolio;that is, R=∑R,=∑ =1 i=1 Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 8/81
Asset Return Let Ri denote the total return of asset i. We have R = 1 X0 Xn i=1 RiwiX0 = Xn i=1 wiRi ⇒ 1 + r = Xn i=1 wi(1 + ri) ⇒ r = Xn i=1 wiri . Formula (Portfolio return) Both total return and the rate of return of a portfolio of assets are equal to the weighted sum of the corresponding individual asset returns, with the weight of an asset being its relative weight (in purchase cost) in the portfolio; that is, R = Xn i=1 wiRi , r = Xn i=1 wiri . Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 8 / 81
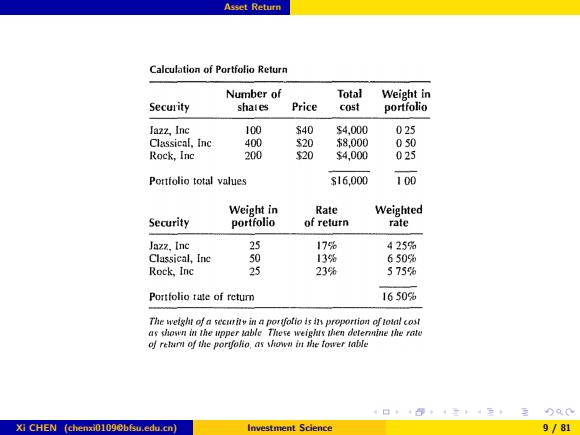
Asset Return Calculation of Portfolio Return Number of Total Weight in Security shales Price cost portfolio Jazz,Inc 100 340 s4,000 025 Classical,Inc 400 $20 $8,000 0s0 Rock,Inc 200 20 s4,000 025 Portfolio total values 316.000 100 Weight in Rate Weighted Security portfolio of return rate Jazz,【nc 25 17% 425% Classical,Inc 50 13% 650% Rock,Inc 25 23% 575% Portfolio tate of retum 1650% The weight of a securits in a poryfolio is in proportion of total cost as shown in the upper lable Theve weighus then deternine the rate of retarn of the porgfolio,as showu in the Tower table Xi CHEN (chenxi01090bfsu.edu.cn) Investment Science 9/81
Asset Return Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 9 / 81

Random Variables (self-learning) Outline 19 Asset Return ② Random Variables (self-learning) Random Returns (self-learning) Portfolio Mean and Variance The Feasible Set ⑥The Markowitz Model The Two-Fund Theorem Inclusion of a Risk-Free Asset The One-Fund Theorem 4口4+4三4至,至)只0 Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 10/81
Random Variables (self-learning) Outline 1 Asset Return 2 Random Variables (self-learning) 3 Random Returns (self-learning) 4 Portfolio Mean and Variance 5 The Feasible Set 6 The Markowitz Model 7 The Two-Fund Theorem 8 Inclusion of a Risk-Free Asset 9 The One-Fund Theorem Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 10 / 81

Random Returns (self-learning) Outline Asset Return Random Variables (self-learning) 3 Random Returns(self-learning) Portfolio Mean and Variance The Feasible Set ⑥The Markowitz Model The Two-Fund Theorem Inclusion of a Risk-Free Asset The One-Fund Theorem 4口4+4三4至,至)只0 Xi CHEN (chenxi01090bfsu.edu.cn) Investment Science 11/81
Random Returns (self-learning) Outline 1 Asset Return 2 Random Variables (self-learning) 3 Random Returns (self-learning) 4 Portfolio Mean and Variance 5 The Feasible Set 6 The Markowitz Model 7 The Two-Fund Theorem 8 Inclusion of a Risk-Free Asset 9 The One-Fund Theorem Xi CHEN (chenxi0109@bfsu.edu.cn) Investment Science 11 / 81