
4.Asymptotic Spectrum Theorems 1
1 4. Asymptotic Spectrum Theorems

Overview We will talk asymptotic spectral distributions such as: ·Semicircle law ·Circular law Marcenko-Pastur law (MP law) ·Quarter circle law 。Ring law 2
Overview 2 We will talk asymptotic spectral distributions such as: • Semicircle law • Circular law • Marcenko-Pastur law (MP law) • Quarter circle law • Ring law
4.1.Why go to infinity The limiting results for infinite dimension matrices are very simple and explicit Apply the limiting results into the analysis of large matrices in realistic applications This approximate method is often stunningly precise and even sometimes can be applied to approximate scenarios where the dimension of the matrix is very small. 3
3 4.1. Why go to infinity ? • The limiting results for infinite dimension matrices are very simple and explicit • Apply the limiting results into the analysis of large matrices in realistic applications • This approximate method is often stunningly precise and even sometimes can be applied to approximate scenarios where the dimension of the matrix is very small
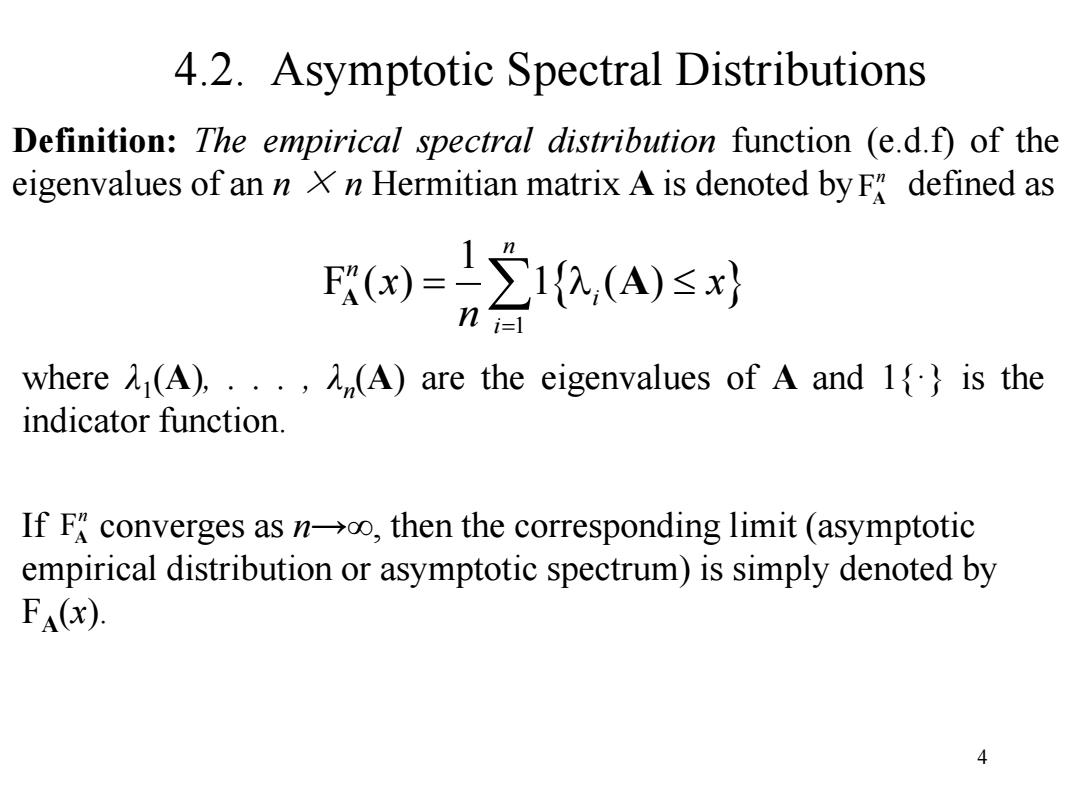
4.2.Asymptotic Spectral Distributions Definition:The empirical spectral distribution function (e.d.f)of the eigenvalues of an n xn Hermitian matrix A is denoted by F defined as 贸)=∑1(A)≤x刘 i=1 where,(A),···,n(A)are the eigenvalues of A and 1{}is the indicator function. If F converges as n->o,then the corresponding limit (asymptotic empirical distribution or asymptotic spectrum)is simply denoted by FA(x). 4
4 4.2. Asymptotic Spectral Distributions Definition: The empirical spectral distribution function (e.d.f) of the eigenvalues of an n × n Hermitian matrix A is denoted by defined as 1 1 F ( ) 1 ( ) n n i i x x n A A F n A where λ1 (A), . . . , λn (A) are the eigenvalues of A and 1{·} is the indicator function. If converges as n→∞, then the corresponding limit (asymptotic empirical distribution or asymptotic spectrum) is simply denoted by FA (x). F n A
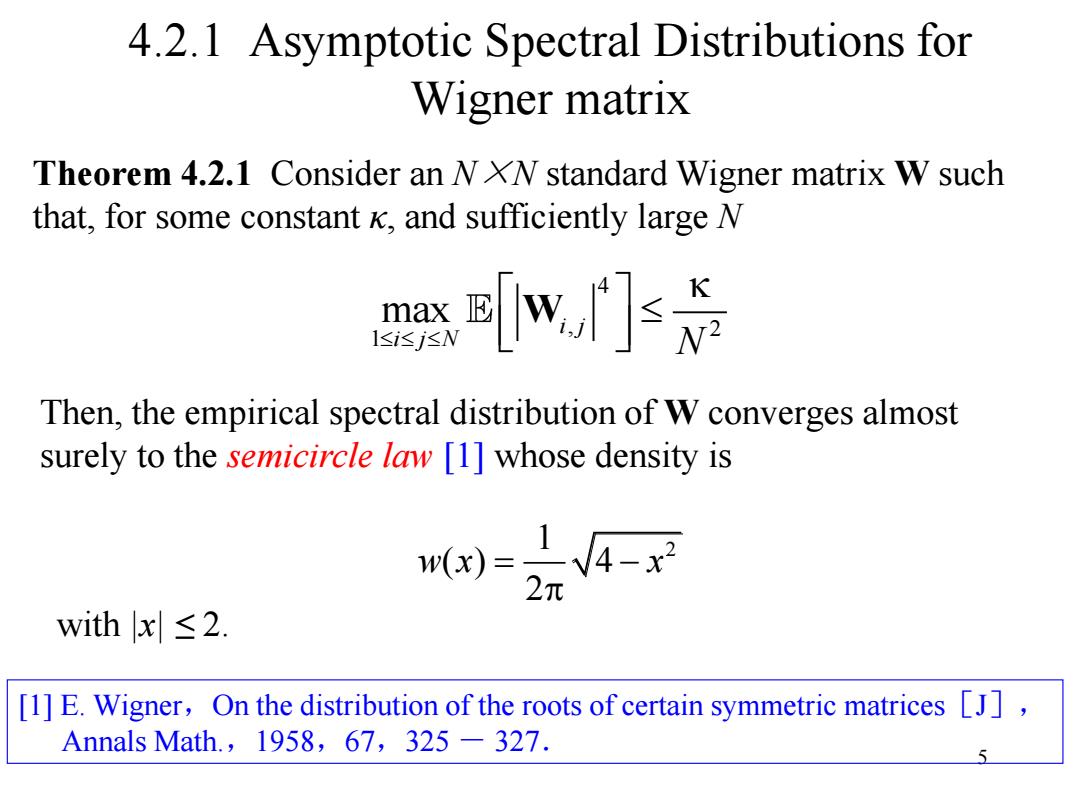
4.2.1 Asymptotic Spectral Distributions for Wigner matrix Theorem 4.2.1 Consider an NXN standard Wigner matrix W such that,for some constant K,and sufficiently large N msw门 Then,the empirical spectral distribution of W converges almost surely to the semicircle law [1]whose density is w()=V4- 2π with x≤2. [1]E.Wigner,On the distribution of the roots of certain symmetric matrices [J], Annals Math.,1958,67,325-327
5 4.2.1 Asymptotic Spectral Distributions for Wigner matrix Theorem 4.2.1 Consider an N×N standard Wigner matrix W such that, for some constant κ, and sufficiently large N 4 , 2 1 max i j i j N N W Then, the empirical spectral distribution of W converges almost surely to the semicircle law [1] whose density is 1 2 ( ) 4 2 w x x with |x| ≤ 2. [1] E. Wigner,On the distribution of the roots of certain symmetric matrices[J], Annals Math.,1958,67,325 - 327.