4.Asymptotic Spectrum Theorems 1
1 4. Asymptotic Spectrum Theorems

Overview An other case for spectrum sensing Asymptotic moment method Central limit theorem 2
2 Overview • An other case for spectrum sensing • Asymptotic moment method • Central limit theorem
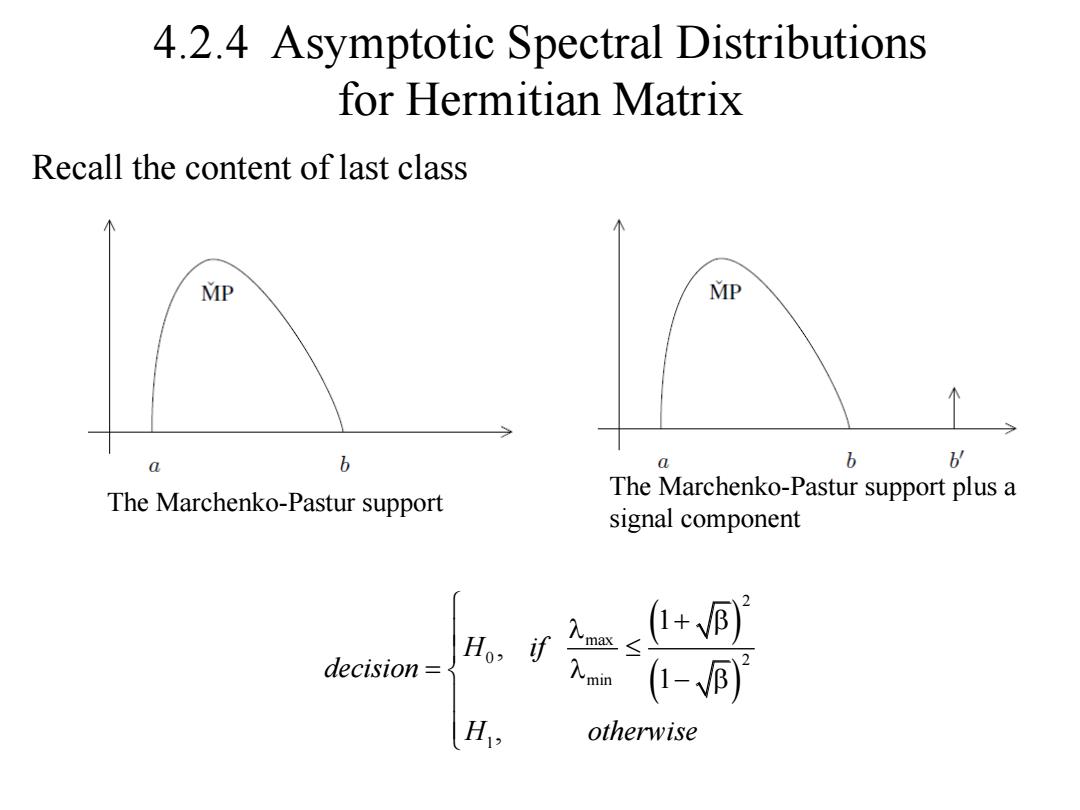
4.2.4 Asymptotic Spectral Distributions for Hermitian Matrix Recall the content of last class MP MP a b b b The Marchenko-Pastur support The Marchenko-Pastur support plus a signal component H otherwise
4.2.4 Asymptotic Spectral Distributions for Hermitian Matrix Recall the content of last class 2 max 0 2 min 1 1 , 1 , H if decision H otherwise The Marchenko-Pastur support The Marchenko-Pastur support plus a signal component
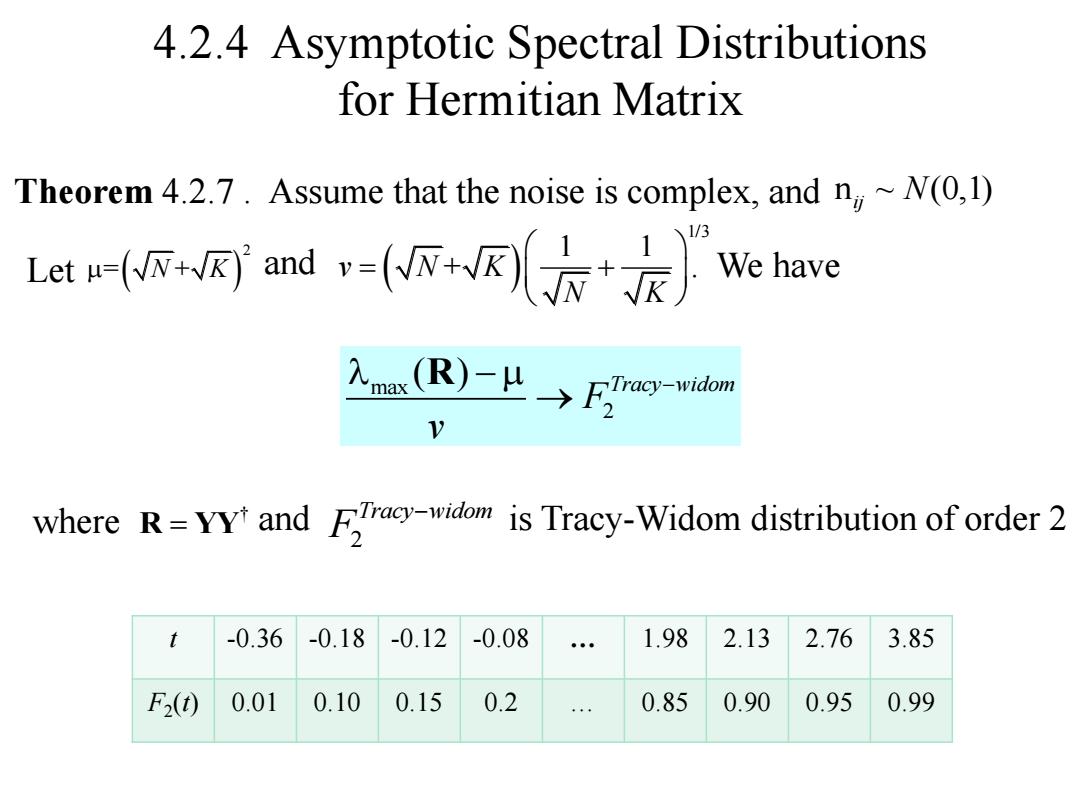
4.2.4 Asymptotic Spectral Distributions for Hermitian Matrix Theorem 4.2.7.Assume that the noise is complex,and n~N(0,1) /3 Let(网and-人r+W风+灰We have 入n(R)-L→Fa-witm where R=YY and Tiom is Tracy-Widom distribution of order 2 -0.36 -0.18 -0.12 -0.08 1.98 2.13 2.76 3.85 F2() 0.01 0.10 0.15 0.2 0.85 0.90 0.95 0.99
4.2.4 Asymptotic Spectral Distributions for Hermitian Matrix Theorem 4.2.7 . Assume that the noise is complex, and 2 = + N K and 1/3 1 1 v N K + N K . We have max 2 ( ) Tracy widom F v R where † R YY 2 Tracy widom F and is Tracy-Widom distribution of order 2 t -0.36 -0.18 -0.12 -0.08 … 1.98 2.13 2.76 3.85 F2 (t) 0.01 0.10 0.15 0.2 … 0.85 0.90 0.95 0.99 n ~ (0,1) ij N Let
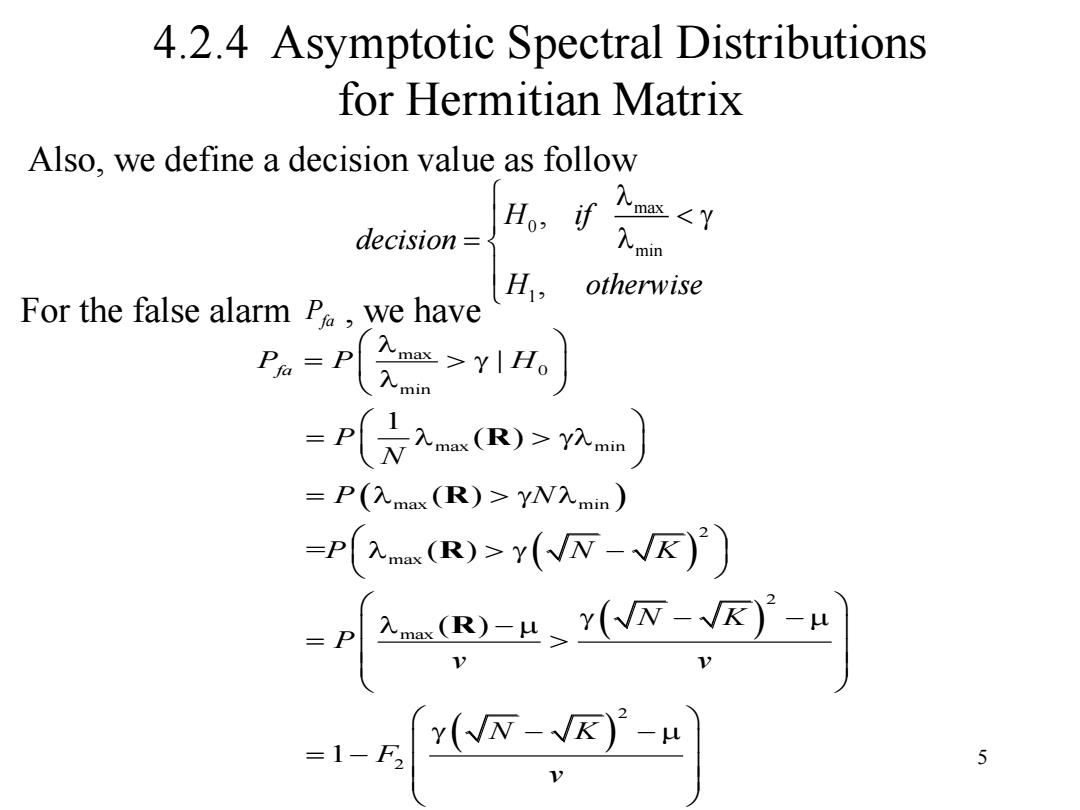
4.2.4 Asymptotic Spectral Distributions for Hermitian Matrix Also,we define a decision value as follow Ho2 入m∠Y decision 入nin H otherwise For the false alarm P,we have P。=P>Y1H =P(入na(R)>Yin =P(max(R)>YNAmin) =P(入mx(R)>y(WN-√K)) 2- 1-F Y(N-R)-m 5
5 4.2.4 Asymptotic Spectral Distributions for Hermitian Matrix max 0 min 1 , , H if decision H otherwise Also, we define a decision value as follow max 0 min max min max min 2 max 2 max 2 2 | 1 ( ) ( ) = ( ) ( ) 1 P P H fa P N P N P N K N K P v v N K F v R R R R For the false alarm , we have Pfa