
5. Free Probability 1
1 5. Free Probability
5. Free Probability It is in general a difficult problem to deduce the eigenvalue distribution of the sum or the product of two generic Hermitian matrices A,B as a function of the eigenvalue distributions of A and B.In fact,it is often impossible as the eigenvectors of A and B intervene in the expression of the eigenvalues of their sum or product. Free probability will be shown to provide a very efficient framework to study limiting distributions of some models like the sum or the product of two Hermitian matrices 2
2 5. Free Probability It is in general a difficult problem to deduce the eigenvalue distribution of the sum or the product of two generic Hermitian matrices A, B as a function of the eigenvalue distributions of A and B. In fact, it is often impossible as the eigenvectors of A and B intervene in the expression of the eigenvalues of their sum or product. Free probability will be shown to provide a very efficient framework to study limiting distributions of some models like the sum or the product of two Hermitian matrices

5. Free Probability Free probability is a mathematical theory that studies non-commutative random variables,such as matrices.The "freeness"or free independence property is the analogue of the classical notion of independence, The significance of free probability to random matrix theory lies in the fundamental observation that random matrices that have independent entries in the classical sense,also satisfy the "freeness property"in the free probability theory,in the asymptotic region. This fundamental observation is called as asymptotically free. Besides any random matrices, identity matrices also s satisfy asymptotically free 3
3 5. Free Probability Free probability is a mathematical theory that studies non-commutative random variables, such as matrices. The "freeness" or free independence property is the analogue of the classical notion of independence, The significance of free probability to random matrix theory lies in the fundamental observation that random matrices that have independent entries in the classical sense, also satisfy the "freeness property" in the free probability theory, in the asymptotic region. This fundamental observation is called as asymptotically free. Besides any random matrices, identity matrices also satisfy asymptotically free
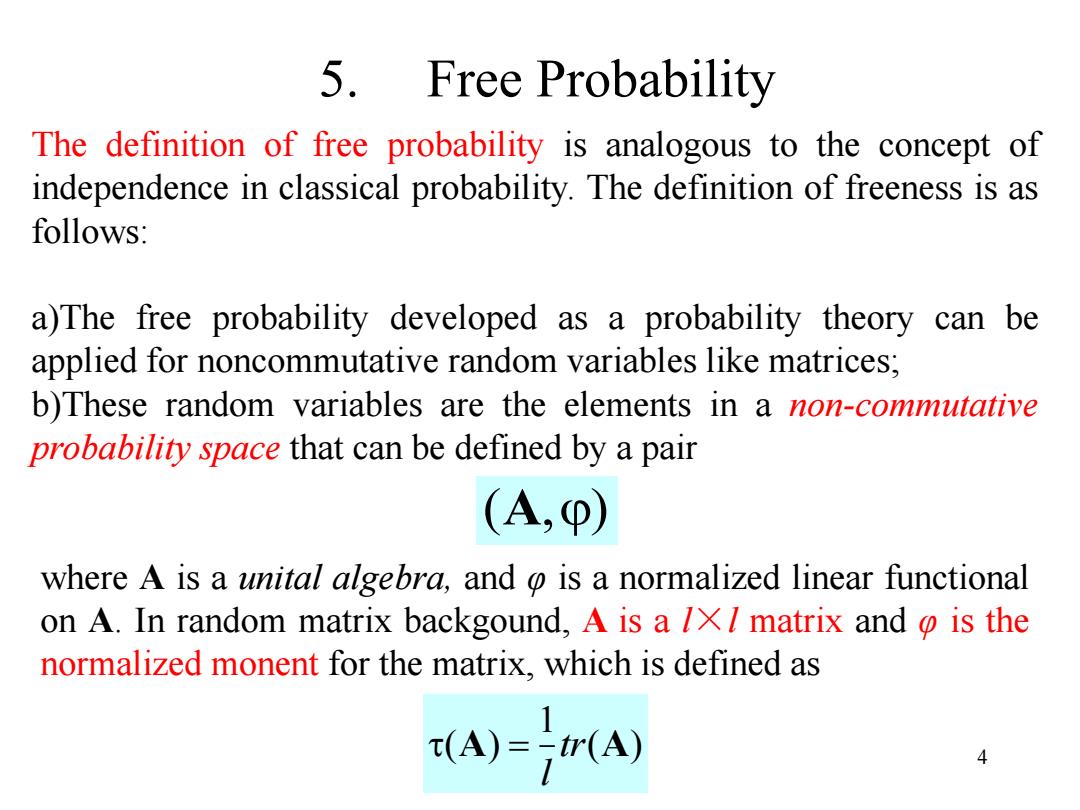
5. Free Probability The definition of free probability is analogous to the concept of independence in classical probability.The definition of freeness is as follows: a)The free probability developed as a probability theory can be applied for noncommutative random variables like matrices; b)These random variables are the elements in a non-commutative probability space that can be defined by a pair (A,φ) where A is a unital algebra,and o is a normalized linear functional on A.In random matrix backgound,A is a IXI matrix and o is the normalized monent for the matrix,which is defined as 4
4 5. Free Probability The definition of free probability is analogous to the concept of independence in classical probability. The definition of freeness is as follows: a)The free probability developed as a probability theory can be applied for noncommutative random variables like matrices; b)These random variables are the elements in a non-commutative probability space that can be defined by a pair ( , ) A where A is a unital algebra, and φ is a normalized linear functional on A. In random matrix backgound, A is a l×l matrix and φ is the normalized monent for the matrix, which is defined as 1 ( ) ( ) tr l A A
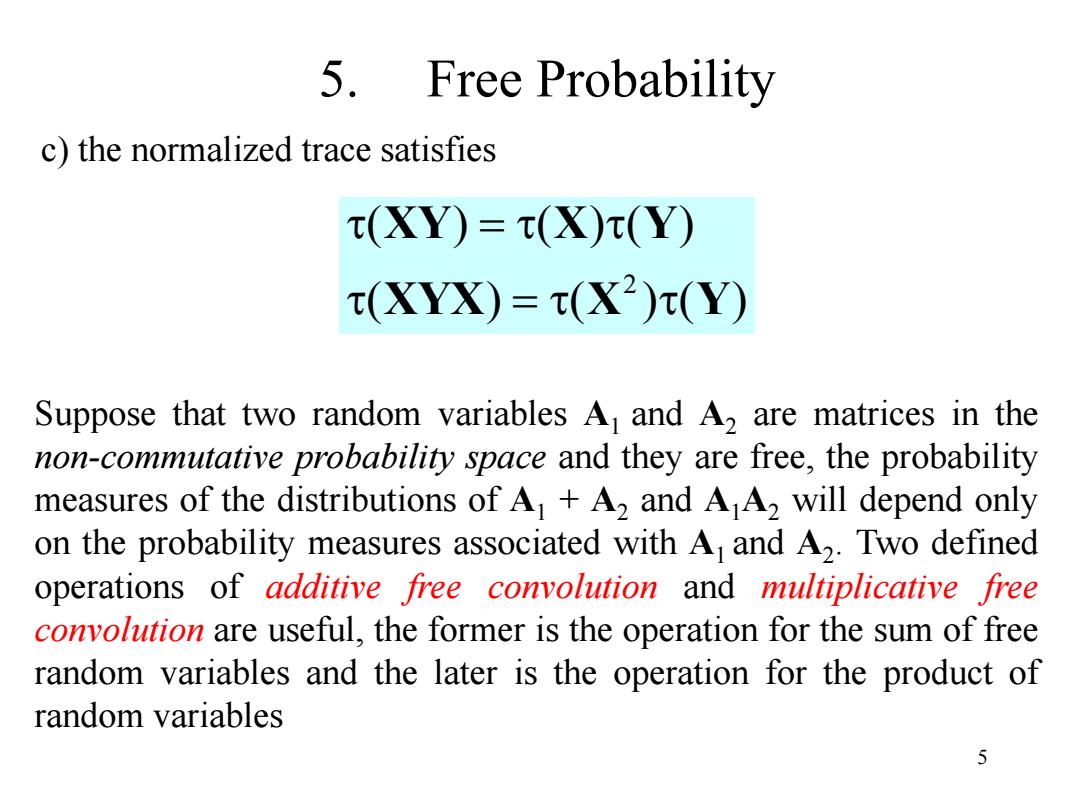
5. Free Probability c)the normalized trace satisfies (XY)=(X)(Y) (XYX)=(X(Y) Suppose that two random variables A and A,are matrices in the non-commutative probability space and they are free,the probability measures of the distributions of A+A2 and A A2 will depend only on the probability measures associated with A and A2.Two defined operations of additive free convolution and multiplicative free convolution are useful,the former is the operation for the sum of free random variables and the later is the operation for the product of random variables 5
5 5. Free Probability c) the normalized trace satisfies 2 ( ) ( ) ( ) ( ) ( ) ( ) XY X Y XYX X Y Suppose that two random variables A1 and A2 are matrices in the non-commutative probability space and they are free, the probability measures of the distributions of A1 + A2 and A1A2 will depend only on the probability measures associated with A1 and A2 . Two defined operations of additive free convolution and multiplicative free convolution are useful, the former is the operation for the sum of free random variables and the later is the operation for the product of random variables