
What is a Matrix? A matrix definition An array of numbers a11 a1n amn with m rows and n columns is an m x n matrix. Element aij is located in position (i,j). The element aij are scalars,namely,real or complex numbers. 命电有这女子 Matrix Theory Matrices -4/13
What is a Matrix? A matrix definition An array of numbers A = ⎛ ⎜ ⎝ a11 . . . a1n ⋮ ⋮ am1 . . . amn ⎞ ⎟ ⎠ with m rows and n columns is an m × n matrix. ▸ Element aij is located in position (i, j). ▸ The element aij are scalars, namely, real or complex numbers. ▸ The set of real numbers is R; the set of complex numbers is C. Remarks: 1. A matrix is a rectangular pattern of numbers –we usually enclose the numbers with brackets. 2. These elements can be any numbers we choose - positive, negative, zero, fractions, decimals, and so on. 3. To refer briefly to a specific matrix we might label it, usually with a capital letter. Matrix Theory Matrices - 4/13
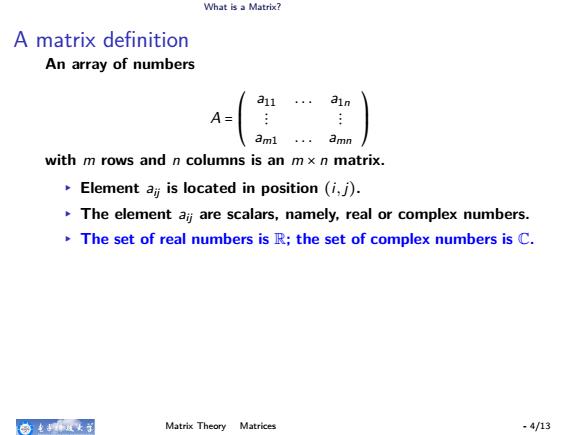
What is a Matrix? A matrix definition An array of numbers a11 A= amn with m rows and n columns is an m x n matrix. Element aij is located in position(i,j). The element aij are scalars,namely,real or complex numbers. .The set of real numbers is R;the set of complex numbers is C. 命电有这女 Matrix Theory Matrices -4/13
What is a Matrix? A matrix definition An array of numbers A = ⎛ ⎜ ⎝ a11 . . . a1n ⋮ ⋮ am1 . . . amn ⎞ ⎟ ⎠ with m rows and n columns is an m × n matrix. ▸ Element aij is located in position (i, j). ▸ The element aij are scalars, namely, real or complex numbers. ▸ The set of real numbers is R; the set of complex numbers is C. Remarks: 1. A matrix is a rectangular pattern of numbers –we usually enclose the numbers with brackets. 2. These elements can be any numbers we choose - positive, negative, zero, fractions, decimals, and so on. 3. To refer briefly to a specific matrix we might label it, usually with a capital letter. Matrix Theory Matrices - 4/13

What is a Matrix? A matrix definition An array of numbers a11 A- aml amn with m rows and n columns is an m x n matrix. Element aij is located in position(i,j). The element aij are scalars,namely,real or complex numbers. The set of real numbers is R;the set of complex numbers is C. Remarks: 奇电有这头 Matrix Theory Matrices -4/13
What is a Matrix? A matrix definition An array of numbers A = ⎛ ⎜ ⎝ a11 . . . a1n ⋮ ⋮ am1 . . . amn ⎞ ⎟ ⎠ with m rows and n columns is an m × n matrix. ▸ Element aij is located in position (i, j). ▸ The element aij are scalars, namely, real or complex numbers. ▸ The set of real numbers is R; the set of complex numbers is C. Remarks: 1. A matrix is a rectangular pattern of numbers –we usually enclose the numbers with brackets. 2. These elements can be any numbers we choose - positive, negative, zero, fractions, decimals, and so on. 3. To refer briefly to a specific matrix we might label it, usually with a capital letter. Matrix Theory Matrices - 4/13

What is a Matrix? A matrix definition An array of numbers a11 a1n A aml amn with m rows and n columns is an m x n matrix. Element aij is located in position(i,j). The element aij are scalars,namely,real or complex numbers. The set of real numbers is R;the set of complex numbers is C. Remarks: 1.A matrix is a rectangular pattern of numbers-we usually enclose the numbers with brackets. 命电有这女 Matrix Theory Matrices -4/13
What is a Matrix? A matrix definition An array of numbers A = ⎛ ⎜ ⎝ a11 . . . a1n ⋮ ⋮ am1 . . . amn ⎞ ⎟ ⎠ with m rows and n columns is an m × n matrix. ▸ Element aij is located in position (i, j). ▸ The element aij are scalars, namely, real or complex numbers. ▸ The set of real numbers is R; the set of complex numbers is C. Remarks: 1. A matrix is a rectangular pattern of numbers –we usually enclose the numbers with brackets. 2. These elements can be any numbers we choose - positive, negative, zero, fractions, decimals, and so on. 3. To refer briefly to a specific matrix we might label it, usually with a capital letter. Matrix Theory Matrices - 4/13
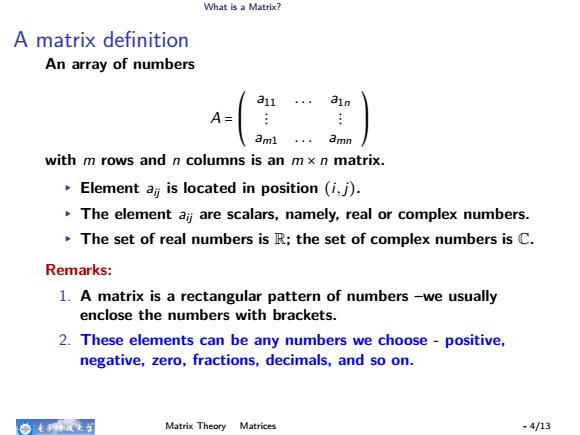
What is a Matrix? A matrix definition An array of numbers a11 。。 a1n aml amn with m rows and n columns is an m x n matrix. Element aij is located in position (i,j). The element aij are scalars,namely,real or complex numbers. The set of real numbers is R;the set of complex numbers is C. Remarks: 1.A matrix is a rectangular pattern of numbers-we usually enclose the numbers with brackets. 2.These elements can be any numbers we choose-positive, negative,zero,fractions,decimals,and so on. 奇电这头了 Matrix Theory Matrices -4/13
What is a Matrix? A matrix definition An array of numbers A = ⎛ ⎜ ⎝ a11 . . . a1n ⋮ ⋮ am1 . . . amn ⎞ ⎟ ⎠ with m rows and n columns is an m × n matrix. ▸ Element aij is located in position (i, j). ▸ The element aij are scalars, namely, real or complex numbers. ▸ The set of real numbers is R; the set of complex numbers is C. Remarks: 1. A matrix is a rectangular pattern of numbers –we usually enclose the numbers with brackets. 2. These elements can be any numbers we choose - positive, negative, zero, fractions, decimals, and so on. 3. To refer briefly to a specific matrix we might label it, usually with a capital letter. Matrix Theory Matrices - 4/13