
Introduction On the cosmic scale,gravitation dominates the universe.Nuclear and electromagnetic forces account for the detailed processes that allow stars to shine and astronomers to see them.But it is gravitation that shapes the universe, determining the geometry of space and time and thus the large-scale distribution of galaxies.Providing insight into gravitation-its effects,its nature and its causes -is therefore rightly seen as one of the most important goals of physics and astronomy Through more than a thousand years of human history the common explanation of gravitation was based on the Aristotelian belief that objects had a natural place in an Earth-centred universe that they would seek out if free to do so.For about two and a half centuries the Newtonian idea of gravity as a force held sway.Then,in the twentieth century,came Einstein's conception of gravity as a manifestation of spacetime curvature.It is this latter view that is the main concern of this book. The story of Einsteinian gravitation begins with a failure.Einstein's theory of special relativity,published in 1905 while he was working as a clerk in the Swiss Figure I Albert Einstein Patent Office in Bern,marked an enormous step forward in theoretical physics (1879-1955)depicted during the and soon brought him academic recognition and personal fame.However,it also time that he worked at the Patent showed that the Newtonian idea of a gravitational force was inconsistent with the Office in Bern.While there,he relativistic approach and that a new theory of gravitation was required.Ten years published a series of papers later,Einstein's general theory of relativity met that need,highlighting the relating to special relativity, important role of geometry in accounting for gravitational phenomena and leading quantum physics and statistical on to concepts such as black holes and gravitational waves.Within a year and a mechanics.He was awarded the half of its completion,the new theory was providing the basis for a novel approach Nobel Prize for Physics in 1921, to cosmology-the science of the universe-that would soon have to take account mainly for his work on the of the astronomy of galaxies and the physics of cosmic expansion.The change in photoelectric effect. thinking demanded by relativity was radical and profound.Its mastery is one of the great challenges and greatest delights of any serious study of physical science. This book begins with two chapters devoted to special relativity.These are followed by a mainly mathematical chapter that provides the background in geometry that is needed to appreciate Einstein's subsequent development of the theory.Chapter 4 examines the basic principles and assumptions of general relativity-Einstein's theory of gravity-while Chapters 5 and 6 apply the theory to an isolated spherical body and then extend that analysis to non-rotating and rotating black holes.Chapter 7 concerns the testing of general relativity,including the use of astronomical observations and gravitational waves.Finally,Chapter 8 examines modern relativistic cosmology,setting the scene for further and ongoing studies of observational cosmology. The text before you is the result of a collaborative effort involving a team of authors and editors working as part of the broader effort to produce the Open University course S383 The Relativistic Universe.Details of the team's membership and responsibilities are listed elsewhere but it is appropriate to acknowledge here the particular contributions of Jim Hague regarding Chapters 1 and 2,Derek Capper concerning Chapters 3,4 and 7,and Aiden Droogan in relation to Chapters 5,6 and 8.Robert Lambourne was responsible for planning and producing the final unified text which benefited greatly from the input of the S383 Course Team Chair,Andrew Norton,and the attention of production editor
Introduction On the cosmic scale, gravitation dominates the universe. Nuclear and electromagnetic forces account for the detailed processes that allow stars to shine and astronomers to see them. But it is gravitation that shapes the universe, determining the geometry of space and time and thus the large-scale distribution of galaxies. Providing insight into gravitation – its effects, its nature and its causes – is therefore rightly seen as one of the most important goals of physics and astronomy. Through more than a thousand years of human history the common explanation of gravitation was based on the Aristotelian belief that objects had a natural place in an Earth-centred universe that they would seek out if free to do so. For about two and a half centuries the Newtonian idea of gravity as a force held sway. Then, in the twentieth century, came Einstein’s conception of gravity as a manifestation of spacetime curvature. It is this latter view that is the main concern of this book. Figure 1 Albert Einstein (1879–1955) depicted during the time that he worked at the Patent Office in Bern. While there, he published a series of papers relating to special relativity, quantum physics and statistical mechanics. He was awarded the Nobel Prize for Physics in 1921, mainly for his work on the photoelectric effect. The story of Einsteinian gravitation begins with a failure. Einstein’s theory of special relativity, published in 1905 while he was working as a clerk in the Swiss Patent Office in Bern, marked an enormous step forward in theoretical physics and soon brought him academic recognition and personal fame. However, it also showed that the Newtonian idea of a gravitational force was inconsistent with the relativistic approach and that a new theory of gravitation was required. Ten years later, Einstein’s general theory of relativity met that need, highlighting the important role of geometry in accounting for gravitational phenomena and leading on to concepts such as black holes and gravitational waves. Within a year and a half of its completion, the new theory was providing the basis for a novel approach to cosmology – the science of the universe – that would soon have to take account of the astronomy of galaxies and the physics of cosmic expansion. The change in thinking demanded by relativity was radical and profound. Its mastery is one of the great challenges and greatest delights of any serious study of physical science. This book begins with two chapters devoted to special relativity. These are followed by a mainly mathematical chapter that provides the background in geometry that is needed to appreciate Einstein’s subsequent development of the theory. Chapter 4 examines the basic principles and assumptions of general relativity – Einstein’s theory of gravity – while Chapters 5 and 6 apply the theory to an isolated spherical body and then extend that analysis to non-rotating and rotating black holes. Chapter 7 concerns the testing of general relativity, including the use of astronomical observations and gravitational waves. Finally, Chapter 8 examines modern relativistic cosmology, setting the scene for further and ongoing studies of observational cosmology. The text before you is the result of a collaborative effort involving a team of authors and editors working as part of the broader effort to produce the Open University course S383 The Relativistic Universe. Details of the team’s membership and responsibilities are listed elsewhere but it is appropriate to acknowledge here the particular contributions of Jim Hague regarding Chapters 1 and 2, Derek Capper concerning Chapters 3, 4 and 7, and Aiden Droogan in relation to Chapters 5,6and 8. Robert Lambourne was responsible for planning and producing the final unified text which benefited greatly from the input of the S383 Course Team Chair, Andrew Norton, and the attention of production editor 9

Introduction Peter Twomey.The whole team drew heavily on the work and wisdom of an earlier Open University Course Team that was responsible for the production of the course S357 Space,Time and Cosmology. A major aim for this book is to allow upper-level undergraduate students to develop the skills and confidence needed to pursue the independent study of the many more comprehensive texts that are now available to students of relativity, gravitation and cosmology.To facilitate this the current text has largely adopted the notation used in the outstanding book by Hobson et al. General Relativity:An Introduction for Physicists,M.P.Hobson,G.Efstathiou and A.N.Lasenby,Cambridge University Press,2006. Other books that provide valuable further reading are(roughly in order of increasing mathematical demand): An Introduction to Modern Cosmology,A.Liddle,Wiley,1999. Relativity,Gravitation and Cosmology:A Basic Introduction,T-P.Cheng,Oxford University Press:2005. Introducing Einstein's Relativity,R.d'Inverno,Oxford University Press,1992. Relativity:Special,General and Cosmological,W.Rindler,Oxford University Press,2001. Cosmology,S.Weinberg,Cambridge University Press,2008. Two useful sources of reprints of original papers of historical significance are: The Principle of Relativity,A.Einstein et al.,Dover,New York,1952. Cosmological Constants,edited by J.Bernstein and G.Feinberg,Columbia University Press,1986. Those wishing to undertake background reading in astronomy,physics and mathematics to support their study of this book or of any of the others listed above might find the following particularly helpful: An Introduction to Galaxies and Cosmology,edited by M.H.Jones and R.J.A. Lambourne,Cambridge University Press,2003. The seven volumes in the series The Physical World,edited by R.J.A.Lambourne,A.J.Norton et al.,Institute of Physics Publishing,2000. (Go to www.physicalworld.org for further details.) The paired volumes Basic Mathematics for the Physical Sciences,edited by R.J.A.Lambourne and M.H.Tinker,Wiley,2000. Further Mathematics for the Physical Sciences,edited by M.H.Tinker and R.J.A.Lambourne,Wiley,2000. Numbered exercises appear throughout this book.Complete solutions to these exercises can be found at the back of the book.There are a number of lengthy worked examples;these are highlighted by a blue background.There are also several shorter in-text questions that are immediately followed by their answers. These may be treated as questions or examples.The questions are indented and indicated by a filled circle;their answers are also indented and shown by an open circle. 10
Introduction Peter Twomey. The whole team drew heavily on the work and wisdom of an earlier Open University Course Team that was responsible for the production of the course S357 Space, Time and Cosmology. A major aim for this book is to allow upper-level undergraduate students to develop the skills and confidence needed to pursue the independent study of the many more comprehensive texts that are now available to students of relativity, gravitation and cosmology. To facilitate this the current text has largely adopted the notation used in the outstanding book by Hobson et al. General Relativity : An Introduction for Physicists, M. P. Hobson, G. Efstathiou and A. N. Lasenby, Cambridge University Press, 2006. Other books that provide valuable further reading are (roughly in order of increasing mathematical demand): An Introduction to Modern Cosmology, A. Liddle, Wiley, 1999. Relativity, Gravitation and Cosmology : A Basic Introduction, T-P. Cheng, Oxford University Press: 2005. Introducing Einstein’s Relativity, R. d’Inverno, Oxford University Press, 1992. Relativity : Special, General and Cosmological, W. Rindler, Oxford University Press, 2001. Cosmology, S. Weinberg, Cambridge University Press, 2008. Two useful sources of reprints of original papers of historical significance are: The Principle of Relativity, A. Einstein et al., Dover, New York, 1952. Cosmological Constants, edited by J. Bernstein and G. Feinberg, Columbia University Press, 1986. Those wishing to undertake background reading in astronomy, physics and mathematics to support their study of this book or of any of the others listed above might find the following particularly helpful: An Introduction to Galaxies and Cosmology, edited by M. H. Jones and R. J. A. Lambourne, Cambridge University Press, 2003. The seven volumes in the series The Physical World, edited by R. J. A. Lambourne, A. J. Norton et al., Institute of Physics Publishing, 2000. (Go to www.physicalworld.org for further details.) The paired volumes Basic Mathematics for the Physical Sciences, edited by R. J. A. Lambourne and M. H. Tinker, Wiley, 2000. Further Mathematics for the Physical Sciences, edited by M. H. Tinker and R. J. A. Lambourne, Wiley, 2000. Numbered exercises appear throughout this book. Complete solutions to these exercises can be found at the back of the book. There are a number of lengthy worked examples; these are highlighted by a blue background. There are also several shorter in-text questions that are immediately followed by their answers. These may be treated as questions or examples. The questions are indented and indicated by a filled circle; their answers are also indented and shown by an open circle. 10

Chapter I Special relativity a and spacetime Introduction In two seminal papers in 1861 and 1864,and in his treatise of 1873,James Clerk Maxwell(Figure 1.1),Scottish physicist and genius,wrote down his revolutionary unified theory of electricity and magnetism,a theory that is now summarized in the equations that bear his name.One of the deep results of the theory introduced by Maxwell was the prediction that wave-like excitations of combined electric and magnetic fields would travel through a vacuum with the same speed as light. It was soon widely accepted that light itself was an electromagnetic disturbance propagating through space,thus unifying electricity and magnetism with optics. The fundamental work of Maxwell opened the way for an understanding of the universe at a much deeper level.Maxwell himself,in common with many scientists of the nineteenth century,believed in an all-pervading medium called the ether,through which electromagnetic disturbances travelled,just as ocean waves travelled through water.Maxwell's theory predicted that light travels with the same speed in all directions,so it was generally assumed that the theory predicted the results of measurements made using equipment that was at rest with Figure 1.I James Clerk Maxwell(1831-1879) respect to the ether.Since the Earth was expected to move through the ether as it orbited the Sun,measurements made in terrestrial laboratories were expected to developed a theory of show that light actually travelled with different speeds in different directions, electromagnetism that was allowing the speed of the Earth's movement through the ether to be determined. already compatible with special However,experiments,most notably by A.A.Michelson and E.W.Morley in relativity theory several decades 1887,failed to detect any variations in the measured speed of light.This led some before Einstein and others to suspect that measurements of the speed of light in a vacuum would always developed the theory.He is also yield the same result irrespective of the motion of the measuring equipment. famous for major contributions Explaining how this could be the case was a major challenge that prompted to statistical physics and the ingenious proposals from mathematicians and physicists such as Henri Poincare, invention of colour photography. George Fitzgerald and Hendrik Lorentz.However,it was the young Albert Einstein who first put forward a coherent and comprehensive solution in his 1905 paper 'On the electrodynamics of moving bodies',which introduced the special theory of relativity.With the benefit of hindsight,we now realize that Maxwell had unintentionally formulated the first major theory that was consistent with special relativity,a revolutionary new way of thinking about space and time. This chapter reviews the implications of special relativity theory for the understanding of space and time.The narrative covers the fundamentals of the theory,concentrating on some of the major differences between our intuition about space and time and the predictions of special relativity.By the end of this chapter,you should have a broad conceptual understanding of special relativity, and be able to derive its basic equations,the Lorentz transformations,from the postulates of special relativity.You will understand how to use events and intervals to describe properties of space and time far from gravitating bodies.You will also have been introduced to Minkowski spacetime,a four-dimensional fusion of space and time that provides the natural setting for discussions of special relativity. 11
Chapter 1 Special relativity and spacetime Introduction In two seminal papers in 1861 and 1864, and in his treatise of 1873, James Clerk Maxwell (Figure 1.1), Scottish physicist and genius, wrote down his revolutionary unified theory of electricity and magnetism, a theory that is now summarized in the equations that bear his name. One of the deep results of the theory introduced by Maxwell was the prediction that wave-like excitations of combined electric and magnetic fields would travel through a vacuum with the same speed as light. It was soon widely accepted that light itself was an electromagnetic disturbance propagating through space, thus unifying electricity and magnetism with optics. Figure 1.1 James Clerk Maxwell (1831–1879) developed a theory of electromagnetism that was already compatible with special relativity theory several decades before Einstein and others developed the theory. He is also famous for major contributions to statistical physics and the invention of colour photography. The fundamental work of Maxwell opened the way for an understanding of the universe at a much deeper level. Maxwell himself, in common with many scientists of the nineteenth century, believed in an all-pervading medium called the ether, through which electromagnetic disturbances travelled, just as ocean waves travelled through water. Maxwell’s theory predicted that light travels with the same speed in all directions, so it was generally assumed that the theory predicted the results of measurements made using equipment that was at rest with respect to the ether. Since the Earth was expected to move through the ether as it orbited the Sun, measurements made in terrestrial laboratories were expected to show that light actually travelled with different speeds in different directions, allowing the speed of the Earth’s movement through the ether to be determined. However, experiments, most notably by A. A. Michelson and E. W. Morley in 1887, failed to detect any variations in the measured speed of light. This led some to suspect that measurements of the speed of light in a vacuum would always yield the same result irrespective of the motion of the measuring equipment. Explaining how this could be the case was a major challenge that prompted ingenious proposals from mathematicians and physicists such as Henri Poincare,´ George Fitzgerald and Hendrik Lorentz. However, it was the young Albert Einstein who first put forward a coherent and comprehensive solution in his 1905 paper ‘On the electrodynamics of moving bodies’, which introduced the special theory of relativity. With the benefit of hindsight, we now realize that Maxwell had unintentionally formulated the first major theory that was consistent with special relativity, a revolutionary new way of thinking about space and time. This chapter reviews the implications of special relativity theory for the understanding of space and time. The narrative covers the fundamentals of the theory, concentrating on some of the major differences between our intuition about space and time and the predictions of special relativity. By the end of this chapter, you should have a broad conceptual understanding of special relativity, and be able to derive its basic equations, the Lorentz transformations, from the postulates of special relativity. You will understand how to use events and intervals to describe properties of space and time far from gravitating bodies. You will also have been introduced to Minkowski spacetime, a four-dimensional fusion of space and time that provides the natural setting for discussions of special relativity. 11

Chapter I Special relativity and spacetime 1.I Basic concepts of special relativity 1.1.I Events,frames of reference and observers When dealing with special relativity it is important to use language very precisely in order to avoid confusion and error.Fundamental to the precise description of physical phenomena is the concept of an event,the spacetime analogue of a point in space or an instant in time. Events An event is an instantaneous occurrence at a specific point in space. An exploding firecracker or a small light that flashes once are good approximations to events,since each happens at a definite time and at a definite position. To know when and where an event happened,we need to assign some coordinates to it:a time coordinate t and an ordered set of spatial coordinates such as the Cartesian coordinates (x,y,z),though we might equally well use spherical coordinates(r,0,)or any other suitable set.The important point is that we should be able to assign a unique set of clearly defined coordinates to any event. This leads us to our second important concept,a frame of reference. Frames of reference A frame of reference is a system for assigning coordinates to events.It consists of a system of synchronized clocks that allows a unique value of the time to be assigned to any event,and a system of spatial coordinates that allows a unique position to be assigned to any event. In much of what follows we shall make use of a Cartesian coordinate system with axes labelled r,y and z.The precise specification of such a system involves selecting an origin and specifying the orientation of the three orthogonal axes that meet at the origin.As far as the system of clocks is concerned,you can imagine that space is filled with identical synchronized clocks all ticking together(we shall need to say more about how this might be achieved later).When using a particular frame of reference,the time assigned to an event is the time shown on the clock at the site of the event when the event happens.It is particularly important to note that the time of an event is not the time at which the event is seen at some far off point-it is the time at the event itself that matters. Reference frames are often represented by the letter S.Figure 1.2 provides what we hope is a memorable illustration of the basic idea,in this case with just two spatial dimensions.This might be called the frame Sgnome. Among all the frames of reference that might be imagined,there is a class of frames that is particularly important in special relativity.This is the class of inertial frames.An inertial frame of reference is one in which a body that is not subject to any net force maintains a constant velocity.This is Newton's first law of motion,so we can say the following. 12
Chapter 1 Special relativity and spacetime 1.1 Basic concepts of special relativity 1.1.1 Events, frames of reference and observers When dealing with special relativity it is important to use language very precisely in order to avoid confusion and error. Fundamental to the precise description of physical phenomena is the concept of an event, the spacetime analogue of a point in space or an instant in time. Events An event is an instantaneous occurrence ataspecific point in space. An exploding firecracker or a small light that flashes once are good approximations to events, since each happens at a definite time and at a definite position. To know when and where an event happened, we need to assign some coordinates to it: a time coordinate t and an ordered set of spatial coordinates such as the Cartesian coordinates (x, y, z), though we might equally well use spherical coordinates (r, θ, φ) or any other suitable set. The important point is that we should be able to assign a unique set of clearly defined coordinates to any event. This leads us to our second important concept, a frame of reference. Frames of reference A frame of reference is a system for assigning coordinates to events. It consists of a system of synchronized clocks that allows a unique value of the time to be assigned to any event, and a system of spatial coordinates that allows a unique position to be assigned to any event. In much of what follows we shall make use of a Cartesian coordinate system with axes labelled x, y and z. The precise specification of such a system involves selecting an origin and specifying the orientation of the three orthogonal axes that meet at the origin. As far as the system of clocks is concerned, you can imagine that space is filled with identical synchronized clocks all ticking together (we shall need to say more about how this might be achieved later). When using a particular frame of reference, the time assigned to an event is the time shown on the clock at the site of the event when the event happens. It is particularly important to note that the time of an event is not the time at which the event is seen at some far off point — it is the time at the event itself that matters. Reference frames are often represented by the letter S. Figure 1.2 provides what we hope is a memorable illustration of the basic idea, in this case with just two spatial dimensions. This might be called the frame Sgnome. Among all the frames of reference that might be imagined, there is a class of frames that is particularly important in special relativity. This is the class of inertial frames. An inertial frame of reference is one in which a body that is not subject to any net force maintains a constant velocity. This is Newton’s first law of motion, so we can say the following. 12
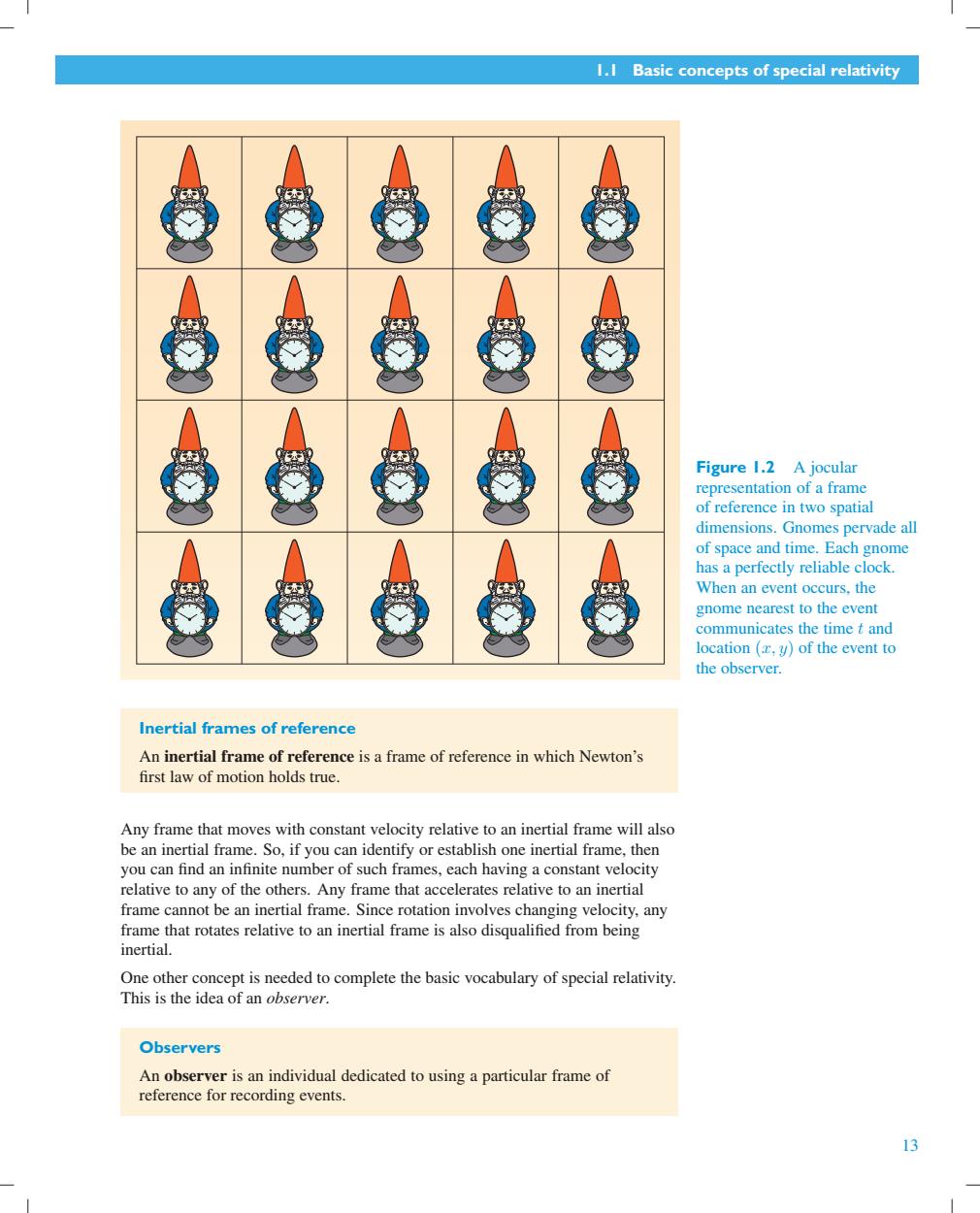
I.I Basic concepts of special relativity Figure 1.2 A jocular representation of a frame of reference in two spatial dimensions.Gnomes pervade all of space and time.Each gnome has a perfectly reliable clock. When an event occurs,the gnome nearest to the event communicates the time t and location (y)of the event to the observer. Inertial frames of reference An inertial frame of reference is a frame of reference in which Newton's first law of motion holds true. Any frame that moves with constant velocity relative to an inertial frame will also be an inertial frame.So,if you can identify or establish one inertial frame,then you can find an infinite number of such frames,each having a constant velocity relative to any of the others.Any frame that accelerates relative to an inertial frame cannot be an inertial frame.Since rotation involves changing velocity,any frame that rotates relative to an inertial frame is also disqualified from being inertial. One other concept is needed to complete the basic vocabulary of special relativity. This is the idea of an observer. Observers An observer is an individual dedicated to using a particular frame of reference for recording events. 13
1.1 Basic concepts of special relativity Figure 1.2 A jocular representation of a frame of reference in two spatial dimensions. Gnomes pervade all of space and time. Each gnome has a perfectly reliable clock. When an event occurs, the gnome nearest to the event communicates the time t and location (x, y) of the event to the observer. Inertial frames of reference An inertial frame of reference is a frame of reference in which Newton’s first law of motion holds true. Any frame that moves with constant velocity relative to an inertial frame will also be an inertial frame. So, if you can identify or establish one inertial frame, then you can find an infinite number of such frames, each having a constant velocity relative to any of the others. Any frame that accelerates relative to an inertial frame cannot be an inertial frame. Since rotation involves changing velocity, any frame that rotates relative to an inertial frame is also disqualified from being inertial. One other concept is needed to complete the basic vocabulary of special relativity. This is the idea of an observer. Observers An observer is an individual dedicated to using a particular frame of reference for recording events. 13