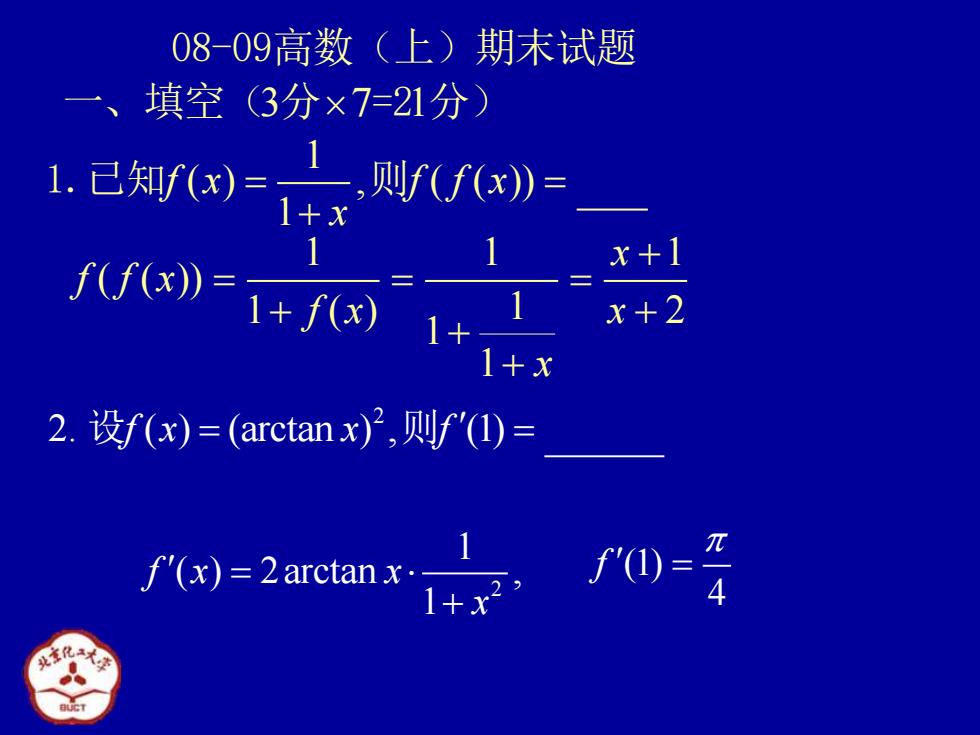
08-09高数(上)期末试题 一、填空(3分×7=21分) 1.已w)=十x则V) 1+x ff(x)=1+f) x+1 1 x+2 1+x 2.设f(x)=(arctanx)2,则f'(①=
08-09高数(上)期末试题 1 ( ) , ( ( )) 1 f x f f x x = = + 1.已知 则 2 2. ( ) (arctan ) , (1) 设f x x f = = 则 一 、填空(3 7 1 分 =2 分) 1 1 1 ( ( )) 1 ( ) 2 1 1 1 x f f x f x x x + = = = + + + + 2 1 ( ) 2arctan , 1 f x x x = + (1) 4 f =

3.过点(2,0,-3)且与直线 x+y+2z-7=0 垂直的 3x+y+z=0 平面方程 所求平面方程的法向量n=1 12={-1,5,-2 311 所求平面方程为:-(x-2)+5y-0)-2(z+3)=0 即:x-5y+2z+4=0
2 - 7 0 2 0 3 3 0 x y z x y z + + = + + = 3.过点( ,,- )且与直线 垂直的 平面方程 1 1 2 { 1,5, 2} 3 1 1 i j k 所求平面方程的法向量n = = − − 即:x y z − + + = 5 2 4 0 所求平面方程为:-( 2) 5( 0) 2( 3) 0 x y z − + − − + =

4.曲面z=x2+y2与平面x+y+z=1的交线在xOy面上的 投影方程 z=x2+y2 消去z得x2+y2-1+x+y=0 X+y+2=1 拟影方为++0+分- 2=0 s)月 x→0 { -1-x lim e-1-x x(e-1) x->0 x2 ex-11 lim x-→0 2x 2
2 2 4 1 .曲面z x y x y z xOy = + + + = 与平面 的交线在 面上的 投影方程 0 1 1 5 lim 1 x x→ x e − = − . 2 2 2 2 1 0 1 z x y z x y x y x y z = + + − + + = + + = 消去 得 1 1 3 2 2 ( ) ( ) 2 2 2 0 x y z + + + = = 投影方程为 2 0 0 0 1 1 1 1 lim lim lim 1 ( 1) x x x x x x x e x e x → → → x e x e x − − − − − = = − − 0 1 1 lim 2 2 x x e → x − = =
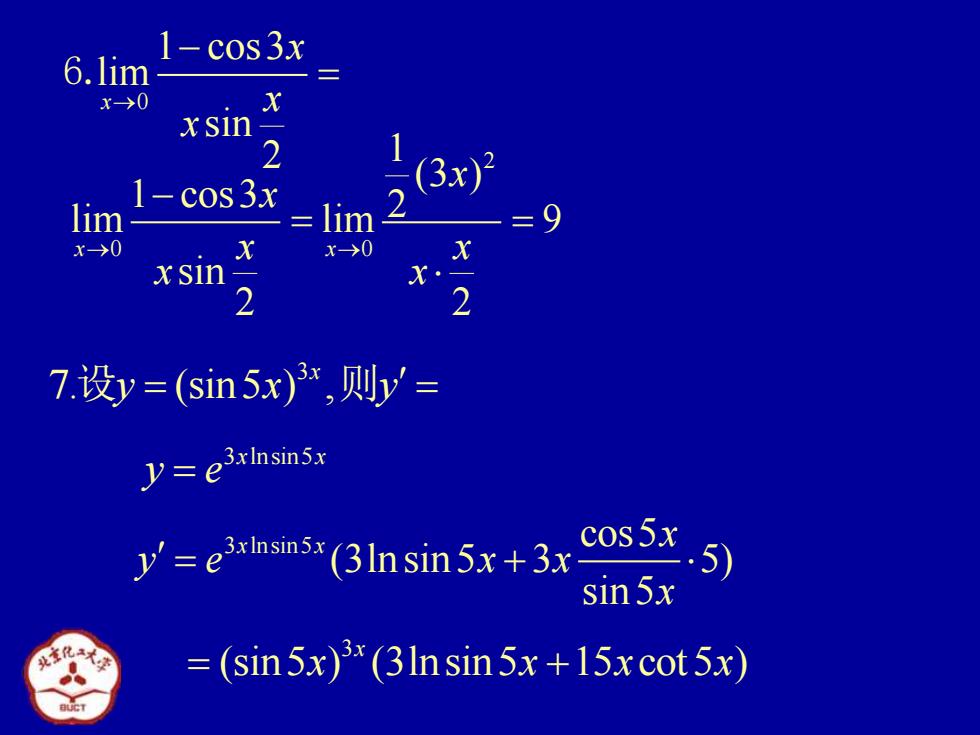
6.lim 1-cos3x x-→>0 xsin- 1-cos3x (3x)月 lim lim -→0 x→0 xsin 2 7设y=(sin5x),则y'= =e3xInsin5x =exnsins*(3In sin5x+3x cos5x 5 sin5x =(sin5x)*(3Insin5x+15xcot 5x)
0 1 cos3 lim sin 2 x x x x → − 6. = 3 7. (sin5 ) , x 设y x y = = 则 2 0 0 1 (3 ) 1 cos3 2 lim lim 9 sin 2 2 x x x x x x x x → → − = = 3 lnsin5 x x y e = 3 lnsin5 cos5 (3ln sin 5 3 5) sin 5 x x x y e x x x = + 3 (sin5 ) (3lnsin5 15 cot 5 ) x = + x x x x

二、解下列各题(6分×6=36分) 1.设y=cos”xsinn,求d 解:y=ncosx(-sinx)sinx+cos”xcosnxn ncos"x.cos(n+1)x dy =[ncos"x.cos(n+1)x]dx
二、解下列各题(6分6=36分) 1 cos sin , n .设y x nx dy = 求 1 cos ( sin ) sin cos cos n n y n x x nx x nx n − 解: = − + 1 cos cos( 1) n n x n x − = + 1 [ cos cos( 1) ] n dy n x n x dx − = +