Interpolatory Splines Note:splines split up range [a, *opposite of CTR-→CSR→GQ development ."Spline"implies no interpolation,not even any y-values ●If given points {(to,o),(t1,y1),(t22),…,(tn,yn)} "interpolatory spline"traverses these as well Splines=nice,analytical functions Copyright©2011NA⊙Yin 6
Interpolatory Splines • Note:splines split up range [a, b] ∗ opposite of CTR→CSR→GQ development • ”Spline” implies no interpolation,not even any y−values • If given points {(t0, y0),(t1, y1),(t2, y2), . . . ,(tn, yn)} ”interpolatory spline” traverses these as well Splines=nice,analytical functions Copyright c 2011 NA Yin 6
Approximation by Splines ●Motivation →Linear Splines ●Quadratic Splines ●Cubic Splines ●Summary Copyright 2011 NAOYin 7
Approximation by Splines • Motivation ⇒ Linear Splines • Quadratic Splines • Cubic Splines • Summary Copyright c 2011 NA Yin 7
Linear Splines Given domain a,b,a spline S(x) Is defined on entire domain .Provides continuity,i.e.,is Co[a,b] partition of "knots" ta=to,ti,t2,...,tn=b} such that S(x)=ax+b∈P([t,ti+1]),i=0,,n-1 Recall:no y-values or interpolation yet Copyright©2011NA⊙Yin 8
Linear Splines Given domain [a, b],a spline S(x) • Is defined on entire domain • Provides continuity,i.e.,is C 0 [a, b] • ∃ partition of ”knots” {a = t0, t1, t2, . . . , tn = b} such that Si(x) = aix + bi ∈ P1([ti , ti+1]), i = 0, . . . , n − 1 Recall:no y−values or interpolation yet Copyright c 2011 NA Yin 8
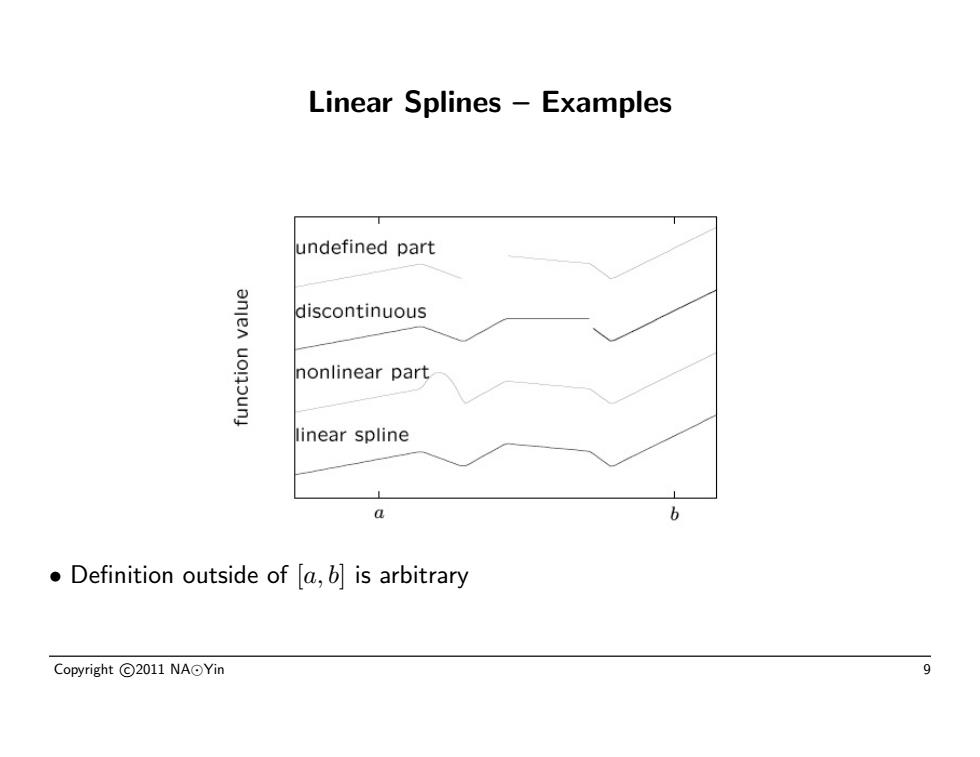
Linear Splines Examples undefined part discontinuous nonlinear part linear spline a Definition outside of a,b is arbitrary Copyright 2011 NAOYin g
Linear Splines – Examples • Definition outside of [a, b] is arbitrary Copyright c 2011 NA Yin 9
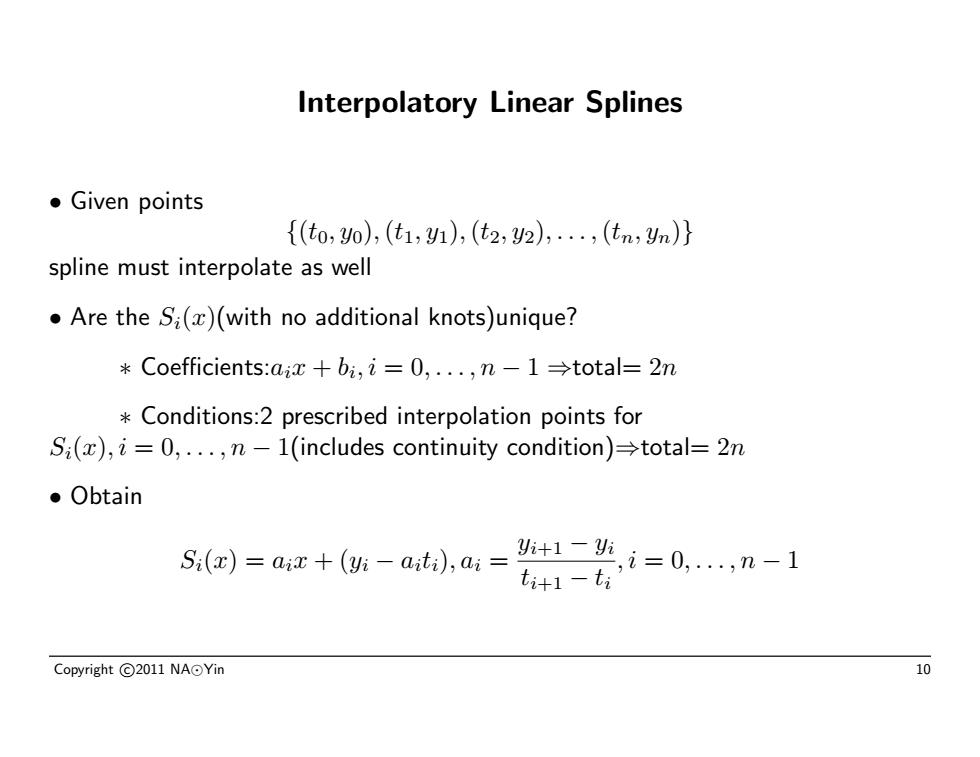
Interpolatory Linear Splines ●Given points {(to,y0),(t1,y1),(t2,y2,,(tn,yn)} spline must interpolate as well .Are the Si(z)(with no additional knots)unique? Coefficients:aix+bi,i=0,...,n-1 =total=2n Conditions:2 prescribed interpolation points for Si(),i=0,...,n-1(includes continuity condition)=total=2n ●Obtain S()=ax+(5-at,a=班+1-,i=0,.,n-1 titi-ti Copyright©2011NA⊙Yin 10
Interpolatory Linear Splines • Given points {(t0, y0),(t1, y1),(t2, y2), . . . ,(tn, yn)} spline must interpolate as well • Are the Si(x)(with no additional knots)unique? ∗ Coefficients:aix + bi , i = 0, . . . , n − 1 ⇒total= 2n ∗ Conditions:2 prescribed interpolation points for Si(x), i = 0, . . . , n − 1(includes continuity condition)⇒total= 2n • Obtain Si(x) = aix + (yi − aiti), ai = yi+1 − yi ti+1 − ti , i = 0, . . . , n − 1 Copyright c 2011 NA Yin 10