
△E=RH( 当n=1,n2=o时,△E=2.179×10-18J, AE-the ionization energy of a hydrogen atom (氢原子的电离能)》 AB-hrv-3289x10(日-) 3.289×1015=2.179×10-18 h It can be seen that the meaning of the constant is the quotient of ionization energy divided by the Planck constant
) 11 ( 2 2 2 1 H nn RE −=Δ h 18 15 10179.2 10289.3 − × =× ) 1 11(10289.3 22 15 ∞ Δ = hE ν ν −×= ΔE − hydrogen a ofenergy ionization the atom. 1 1,当 nn 2 时, E ×=Δ∞== −18 J10179.2 , (氢原子的电离能 ) the quotient of ionization energy divided by the Planck constant。 It can be seen that the meaning of the constant is

The difference in energy (AE)between energy levels n and △En=E。-En=hm=h×3.289x105 2.179×1018 Eorbital energy of the orbital for an electron Regulation(规定):(E.x=0) 一quantum number(量子数) En=-2.179×1018 Calculation of energy of the specified orbit of H atom E.g:the transfer energy between 4th orbital and 2ed orbital: E=5-6=-2179x10“年京=4086x107 He △E4.086×1019 =e=3.0x107 ↑ =486.5nm h 6.626x104=6.167x104g1 6.167×104
Δ n = ∞ − n = hvEEE 1- 22 15 s 11 10289.3 ⎟⎠⎞ ⎜⎝⎛ ∞−××= n h En—— orbital energy of the orbital for an electron n——quantum number (量子数) E.g: the transfer energy between 4th orbital and 2ed orbital: 114 34 19 10167.6 10626.6 10086.4 − − − ×= × × = Δ = s h E v nm5.486 10167.6 100.3 14 17 = × × == v c λ Hβ The difference in energy (ΔEn) between energy levels n and ∝ Regulation(规定):(E∝= 0) Calculation of energy of the specified orbit of H atom J .2 10179 2 18 n − × = EEE J 19 22 18 24 .4) 10086 21 41 .2- 10179 ( − − ×=−×−==Δ J .2 10179 2 18 n En − × =
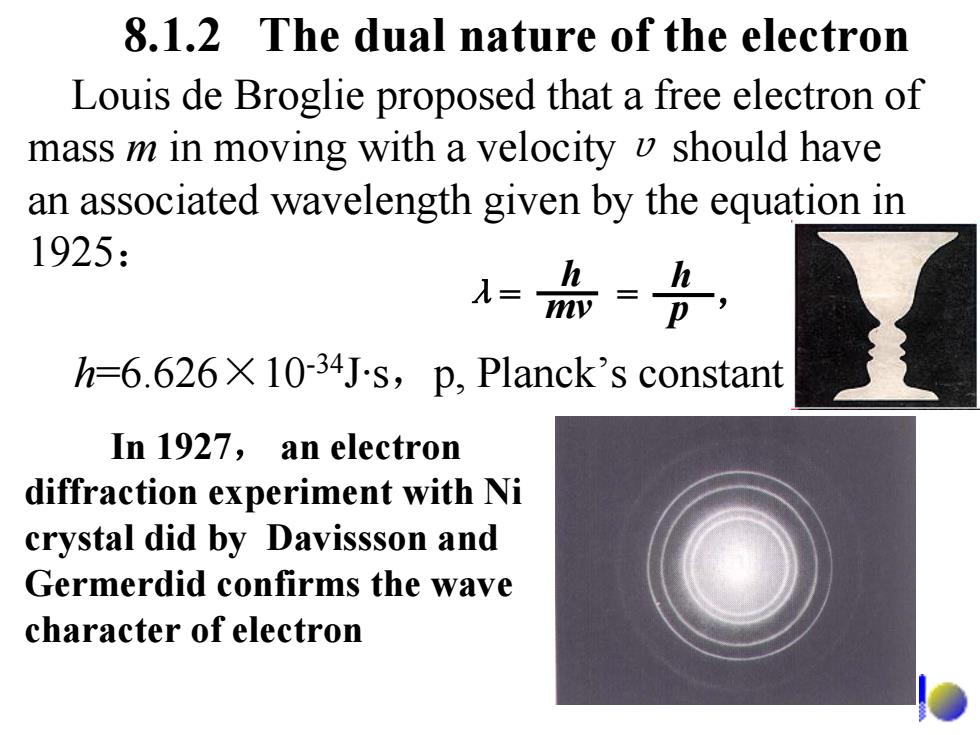
8.1.2 The dual nature of the electron Louis de Broglie proposed that a free electron of mass m in moving with a velocity v should have an associated wavelength given by the equation in 1925: -流=各, h=6.626X 10-34J-s,p,Planck's constant In 1927,an electron diffraction experiment with Ni crystal did by Davissson and Germerdid confirms the wave character of electron
Louis de Broglie proposed that a free electron of mass m in moving with a velocity υ should have an associated wavelength given by the equation in 1925 : 8.1.2 The dual nature of the electron In 1927 , an electron diffraction experiment with Ni crystal did by Davissson and Germerdid confirms the wave character of electron h=6.626 ×10-34J·s ,p, Planck’s constant λ= = , h mv h p

Statistical combination of character of wave and particle of electrons results in the following experimental fact: the wave character of electrons comes from movement of large amount of electrons,i.e.,probability wave in statistics
Statistical combination of character of wave and particle of electrons results in the following experimental fact : the wave character of electrons comes from movement of large amount of electrons, i.e., probability wave in statistics
Supplementary uncertainty principle According to Heisenberg it is impossible to determine simultaneously both the position and velocity of an electron or any other particle with any great degree of accuracy or certainty △x·px=x.m△v≈h So a more accurate position measurement-by adding together more waves-means the momentum measurement becomes less accurate(and vice versa)
According to Heisenberg it is impossible to determine simultaneously both the position and velocity of an electron or any other particle with any great degree of accuracy or certainty So a more accurate position measurement – by adding together more waves – means the momentum measurement becomes less accurate (and vice versa). z uncertainty principle Δx ⋅ Δpx = Δx ⋅ m Δ v ≈ h Supplementary