
0mt=0 +6 +67 The electric field outside is therefore zero.The potential is therefore constant outside the outer sphere and it is zero at infinity,it is also zero on the surface of the outer sphere, V(R2)=0. The electric field between the spheres is given just the field due to a negative charge-O so E=-k2p So the potential difference between the two spheres is given by vR)-vR)=-6j9ri=-t0及尼) Since V(R,)=0,the potential on the inner surface is vR)=e名发)
The electric field outside is therefore zero. The potential is therefore constant outside the outer sphere and it is zero at infinity, it is also zero on the surface of the outer sphere, V(R2 ) = 0 . The electric field between the spheres is given just the field due to a negative charge !Q so ! E = !ke Q r 2 rˆ So the potential difference between the two spheres is given by V(R1 ) ! V(R2 ) = !ke !Q r 2 rˆ " dr rˆ R2 R1 # = !ke Q 1 R1 ! 1 R2 $ % & ' ( ) Since V(R2 ) = 0 , the potential on the inner surface is V(R1 ) = ke Q 1 R2 ! 1 R1 " # $ % & '

Problem 6:Faraday Cage Consider two nested,spherical conducting shells.The first has inner radius a and outer radius b.The second has inner radius c and outer radius d. In the following four situations,determine the total charge on each of the faces of the conducting spheres (inner and outer for each),as well as the electric field and potential everywhere in space (as a function of distance r from the center of the spherical shells). In all cases the shells begin uncharged,and a charge is then instantly introduced somewhere. a)Both shells are not connected to any other conductors(floating)-that is,their net charge will remain fixed.A positive charge +o is introduced into the center of the inner spherical shell.Take the zero of potential to be at infinity. b)The inner shell is floating but the outer shell is grounded-that is,it is fixed at V=0 and has whatever charge is necessary on it to maintain this potential.A negative charge-O is introduced into the center of the inner spherical shell. c)The inner shell is grounded but the outer shell is floating.A positive charge +o is introduced into the center of the inner spherical shell. d)Finally,the outer shell is grounded and the inner shell is floating.This time the positive charge +o is introduced into the region in between the two shells.In this case the questions "What are E(r)and V(r)?"cannot be answered analytically in some regions of space.In the regions where these questions can be answered analytically,give answers.In the regions where they can't be answered analytically,explain why,but try to draw what you think the electric field should look like and give as much information about the potential as possible (is it positive or negative,for example). Solution a)Both shells are not connected to any other conductors (floating)-that is,their net charge will remain fixed.A positive charge +0 is introduced into the center of the inner spherical shell.Take the zero of potential to be at infinity
Problem 6: Faraday Cage Consider two nested, spherical conducting shells. The first has inner radius a and outer radius b. The second has inner radius c and outer radius d. In the following four situations, determine the total charge on each of the faces of the conducting spheres (inner and outer for each), as well as the electric field and potential everywhere in space (as a function of distance r from the center of the spherical shells). In all cases the shells begin uncharged, and a charge is then instantly introduced somewhere. a) Both shells are not connected to any other conductors (floating) – that is, their net charge will remain fixed. A positive charge +Q is introduced into the center of the inner spherical shell. Take the zero of potential to be at infinity. b) The inner shell is floating but the outer shell is grounded – that is, it is fixed at V = 0 and has whatever charge is necessary on it to maintain this potential. A negative charge !Q is introduced into the center of the inner spherical shell. c) The inner shell is grounded but the outer shell is floating. A positive charge +Q is introduced into the center of the inner spherical shell. d) Finally, the outer shell is grounded and the inner shell is floating. This time the positive charge +Q is introduced into the region in between the two shells. In this case the questions “What are ! E(r) and V(r) ?” cannot be answered analytically in some regions of space. In the regions where these questions can be answered analytically, give answers. In the regions where they can’t be answered analytically, explain why, but try to draw what you think the electric field should look like and give as much information about the potential as possible (is it positive or negative, for example). Solution a) Both shells are not connected to any other conductors (floating) – that is, their net charge will remain fixed. A positive charge +Q is introduced into the center of the inner spherical shell. Take the zero of potential to be at infinity
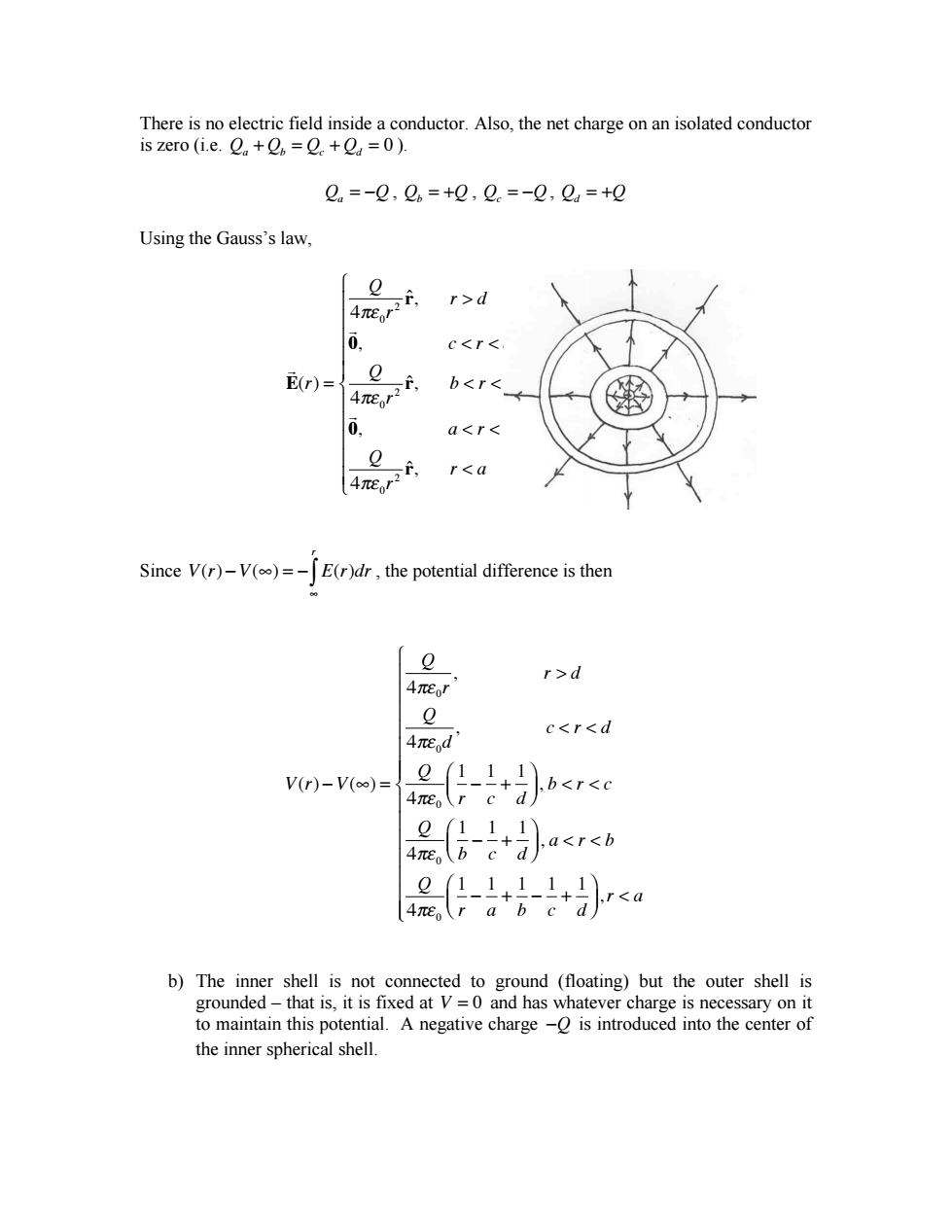
There is no electric field inside a conductor.Also,the net charge on an isolated conductor is zero (i.e.+=+=0). Q.=-Q,Q。=+Q,Q0.=-Q,0a=+Q Using the Gauss's law, 4e,R氏 r>d 0. c<r<. (r)= Q 天 b<r< 0, a<r< 2 4nE,0 r<a Since V(r)-V()=-E(r)dr,the potential difference is then 2 r>d 4πeor Q c<r<d 4πed V(r)-V(∞)= 2111) (b c+apa<r<b 一一十 b)The inner shell is not connected to ground (floating)but the outer shell is grounded-that is,it is fixed at V=0 and has whatever charge is necessary on it to maintain this potential.A negative charge-O is introduced into the center of the inner spherical shell
There is no electric field inside a conductor. Also, the net charge on an isolated conductor is zero (i.e. Qa + Qb = Qc + Qd = 0 ). Qa = !Q , Qb = +Q , Qc = !Q , Qd = +Q Using the Gauss’s law, ! E(r) = Q 4!" 0r 2 rˆ, r > d ! 0, c < r < d Q 4!" 0r 2 rˆ, b < r < c ! 0, a < r < b Q 4!" 0r 2 rˆ, r < a # $ % % % % % & % % % % % Since V(r) ! V(") = ! E(r)d " r # r , the potential difference is then V(r) ! V(") = Q 4#$ 0r , r > d Q 4#$ 0d , c < r < d Q 4#$ 0 1 r ! 1 c + 1 d % & ' ( ) *, b < r < c Q 4#$ 0 1 b ! 1 c + 1 d % & ' ( ) *, a < r < b Q 4#$ 0 1 r ! 1 a + 1 b ! 1 c + 1 d % & ' ( ) *,r < a + , - - - - - -- . - - - - - - - b) The inner shell is not connected to ground (floating) but the outer shell is grounded – that is, it is fixed at V = 0 and has whatever charge is necessary on it to maintain this potential. A negative charge !Q is introduced into the center of the inner spherical shell

Since the outer shell is now grounded,=0 to maintain E(r)=0 outside the outer shell.We have. Q。=Q,9。=-Q,Q.=+Q,0=0. Again using Gauss's Law yields 0, r>c ,b<r<c r)0, 4πer1 a<r<b Q -i,r<a 4e The potential difference is then 0, r>C b<r<c V(r)-V(∞)= 911 4e6e}月 a<r<b 4。+6e r<a c)The inner shell is grounded but the outer shell is not connected to ground.A positive charge +o is introduced into the center of the inner spherical shell. The inner shell is grounded and =0 to maintain E(r)=0 outside the inner shell. Because there is no electric field on the outer shell,==0. Q。=-0,0。=Q.=Q=0 Gauss's Law then yields 0, r>a (r)= Q f,r<a 4πer 露
Since the outer shell is now grounded, Qd = 0 to maintain ! E(r) = ! 0 outside the outer shell. We have. Qa = Q , Qb = !Q , Qc = +Q , Qd = 0 . Again using Gauss’s Law yields ! E(r) = ! 0 , r > c ! Q 4"# 0 r 2 rˆ, b < r < c ! 0, a < r < b ! Q 4"# 0 r 2 rˆ, r < a $ % & & && ' & & & & The potential difference is then V (r) !V (") = 0, r > c ! Q 4#$ 0 1 r ! 1 c % & ' ( ) * , b < r < c ! Q 4#$ 0 1 b ! 1 c % & ' ( ) * , a < r < b ! Q 4#$ 0 1 r ! 1 a + 1 b ! 1 c % & ' ( ) * , r < a + , - - - - . - - - - c) The inner shell is grounded but the outer shell is not connected to ground. A positive charge +Q is introduced into the center of the inner spherical shell. The inner shell is grounded and Qb = 0 to maintain ! E(r) = ! 0 outside the inner shell. Because there is no electric field on the outer shell, Qc = Qd = 0 . Qa = !Q , Qb = Qc = Qd = 0 Gauss’s Law then yields ! E(r) = ! 0, r > a Q 4!" 0r 2 rˆ, r < a # $ % & %