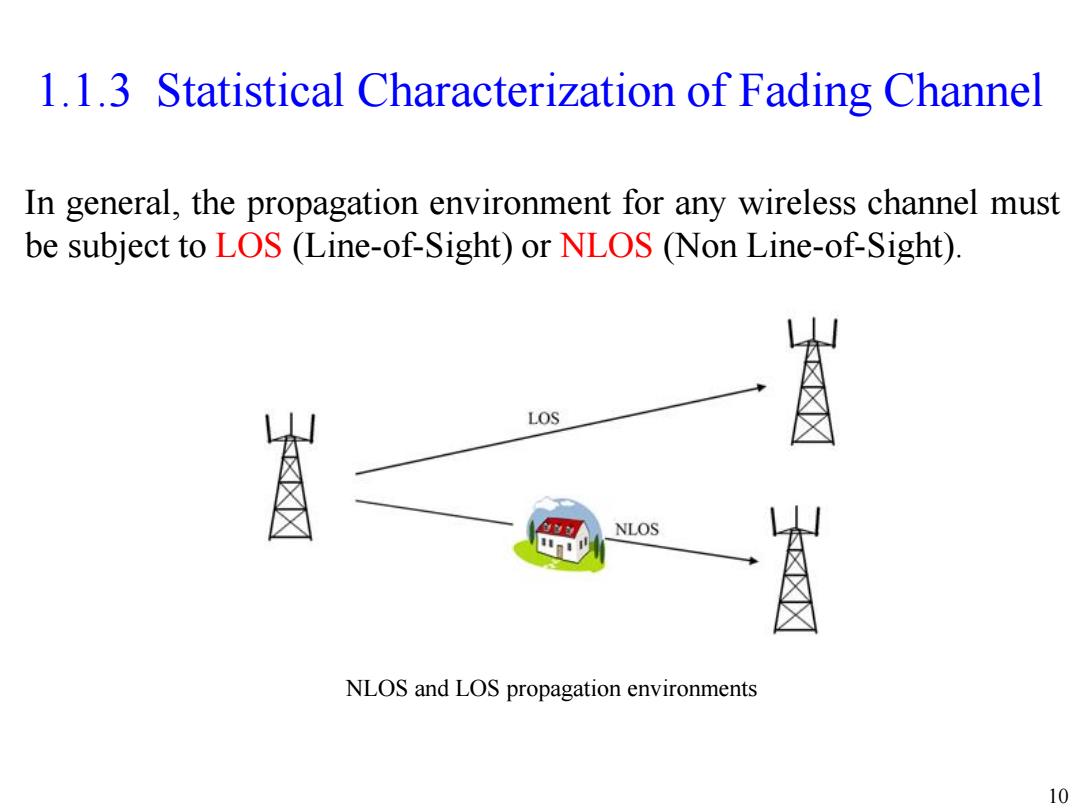
1.1.3 Statistical Characterization of Fading Channel In general,the propagation environment for any wireless channel must be subject to LOS (Line-of-Sight)or NLOS (Non Line-of-Sight). LOS NLOS NLOS and LOS propagation environments 10
10 1.1.3 Statistical Characterization of Fading Channel In general, the propagation environment for any wireless channel must be subject to LOS (Line-of-Sight) or NLOS (Non Line-of-Sight). NLOS and LOS propagation environments
1.1.3 Statistical Characterization of Fading Channel 1.NLOS propagation environments Any received signal in NLOS environment is the sum of the received signals from an infinite number of scatters. By central limit theorem,the received signal can be represented by a Gaussian random variable: W+jW2 where W and W2 are the independent and identically-distributed (i.i.d.)Gaussian random variables with a zero mean and variance 8? 11
11 1.1.3 Statistical Characterization of Fading Channel 1. NLOS propagation environments Any received signal in NLOS environment is the sum of the received signals from an infinite number of scatters. By central limit theorem, the received signal can be represented by a Gaussian random variable: where and are the independent and identically-distributed (i.i.d.) Gaussian random variables with a zero mean and variance . W +jW 1 2 W1 W2 2

1.1.3 Statistical Characterization of Fading Channel 1.NLOS propagation environments Let X denote the amplitude of the complex Gaussian random variable: X-/W+W So,X is a Rayleigh random variable with the following probability density function(PDF): f.)=e 12
12 1. NLOS propagation environments Let X denote the amplitude of the complex Gaussian random variable: So, X is a Rayleigh random variable with the following probability density function (PDF): 1.1.3 Statistical Characterization of Fading Channel 2 2 X= W +W 1 2 2 2 2 2 ( ) x X x f x e
1.1.3 Statistical Characterization of Fading Channel 2.LOS propagation environments In the LOS environment where exists a strong path which is not subject to any loss due to reflection,diffraction,and scattering,the amplitude of the received signal can be expressed as X-C+W+jW, where C represents the LOS component while W and W,are the i.i.d.Gaussian random variables.It has been known that X is the Rician random variable with the PDF: where 1 is the modified zeroth-order Bessel function. 13
13 1.1.3 Statistical Characterization of Fading Channel 2. LOS propagation environments In the LOS environment where exists a strong path which is not subject to any loss due to reflection, diffraction, and scattering, the amplitude of the received signal can be expressed as 2 2 2 2 2 2 0 ( ) x c X x xc f x e I X=C+W +jW 1 2 where represents the LOS component while and are the i.i.d. Gaussian random variables. It has been known that X is the Rician random variable with the PDF: C W1 W2 where is the modified zeroth -order Bessel function. 0 I
1.2 Single Antenna Channels Let us consider the additive white Gaussian noise (AWGN)channel: Y=HX+Z where k∈{I,…,is the time index and ·(X,,Xw)is the input vector satisfying s EsP (Z...,ZN)is the noisy vector,independent of (.....N) .(Y,...,Y)is the output vector; .H,...,H are the fading coefficients. 14
14 1.2 Single Antenna Channels Let us consider the additive white Gaussian noise (AWGN) channel: Y H X Z k k k k = + where is the time index and k 1, N , • (X1 ,…,X N ) is the input vector satisfying 2 1 1 P N k k X N • (Z1 ,…,Z N ) is the noisy vector, independent of (X1 ,…,X N ) • (Y1 ,…,Y N ) is the output vector; • H1 ,…,H N are the fading coefficients