
5. Free Probability Definition 1:Assume that A has an eigenvalue distribution which converges to Y,and A2 has an eigenvalue distribution which converges to Y2,the eigenvalue distribution of A+A2 converges to y that depends only on Y and Y2.Then the measure y is called additive free convolution of y and y2 and it is denoted by YY2. Definition 2:Assume that A has an eigenvalue distribution which converges to Y and A2 has an eigenvalue distribution which converges to Y2,the eigenvalue distribution of A A2 converges to y that depends only on Y and Y2.Then the measure y is termed as multiplicative free convolution of y and Y2,which is denoted by YY2. 6
6 5. Free Probability Definition 1: Assume that A1 has an eigenvalue distribution which converges to γ1,and A2 has an eigenvalue distribution which converges to γ2 , the eigenvalue distribution of A1 + A2 converges to γ that depends only on γ1 and γ2 . Then the measure γ is called additive free convolution of γ1 and γ2 and it is denoted by γ1 γ2 . Definition 2: Assume that A1 has an eigenvalue distribution which converges to γ1 and A2 has an eigenvalue distribution which converges to γ2 , the eigenvalue distribution of A1A2 converges to γ that depends only on γ1 and γ2 . Then the measure γ is termed as multiplicative free convolution of γ1 and γ2 , which is denoted by γ1 γ2

5. Free Probability Associated with the definition 2,multiplicative free deconvolution is defined to deal with the situation where if a unique probability measure y=YY2 is given for the product of two random variables and the probability measure of one of the variables is known,such as Y,then the probability measure of the other random variable y2 can be acquired by the multiplicative free deconvolution,which is denoted by Y2=YㄖYi· Similarly related to definition 1,additive free deconvolution is defined to get Y2 if a unique probability measure y =Y1 Y2 is given for the sum of two random variables and the probability measure of one of the variables y is known.The operation is designated by Y日Y1· 7
7 5. Free Probability Associated with the definition 2, multiplicative free deconvolution is defined to deal with the situation where if a unique probability measure γ = γ1 γ2 is given for the product of two random variables and the probability measure of one of the variables is known, such as γ1 , then the probability measure of the other random variable γ2 can be acquired by the multiplicative free deconvolution, which is denoted by γ2 = γ γ1 . Similarly related to definition 1, additive free deconvolution is defined to get γ2 if a unique probability measure γ = γ1 γ2 is given for the sum of two random variables and the probability measure of one of the variables γ1 is known. The operation is designated by γ γ1
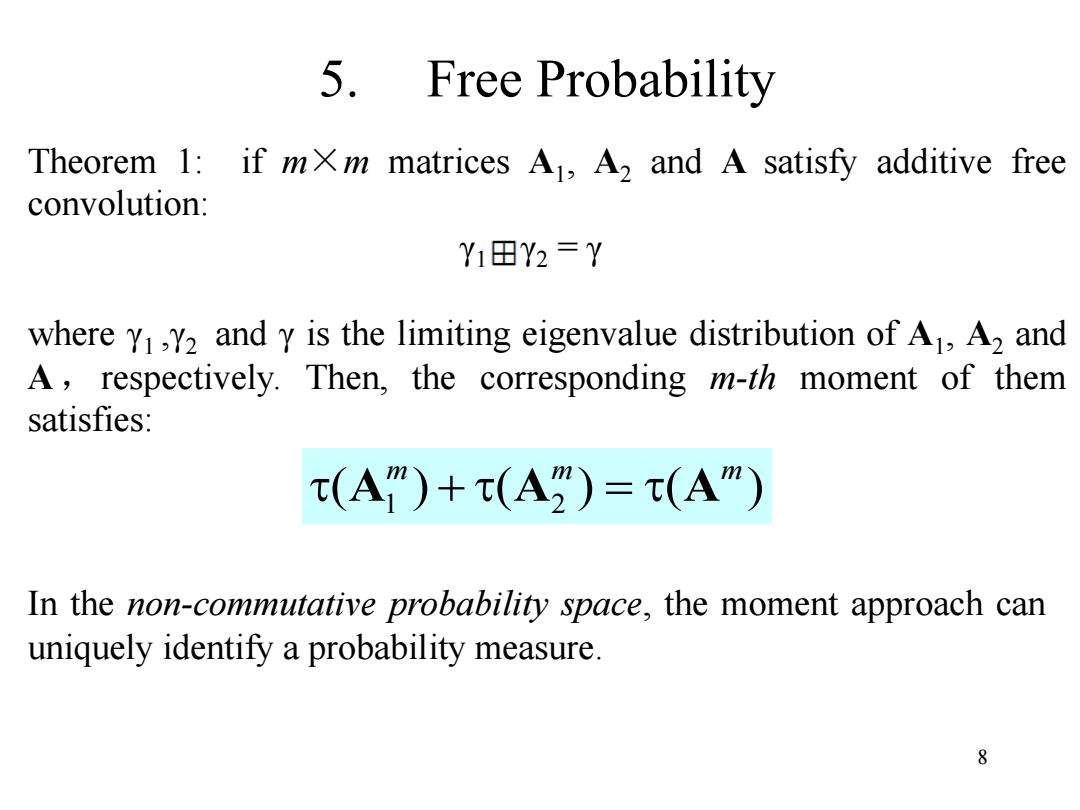
5.Free Probability Theorem 1:if mXm matrices A1,A2 and A satisfy additive free convolution: Y1田Y2=Y where Y1,Y2 and y is the limiting eigenvalue distribution of A,A2 and A,respectively.Then,the corresponding m-th moment of them satisfies: (A")+(A)=(A") In the non-commutative probability space,the moment approach can uniquely identify a probability measure. 8
8 5. Free Probability Theorem 1: if m×m matrices A1 , A2 and A satisfy additive free convolution: γ1 γ2 = γ where γ1 ,γ2 and γ is the limiting eigenvalue distribution of A1 , A2 and A,respectively. Then, the corresponding m-th moment of them satisfies: 1 2 ( ) ( ) ( ) m m m A A A In the non-commutative probability space, the moment approach can uniquely identify a probability measure