电子科技大学：《贝叶斯学习与随机矩阵及在无线通信中的应用 BI-RM-AWC》课程教学资源（课件讲稿）06 Non-asymptotic Analysis for Large Random Matrix

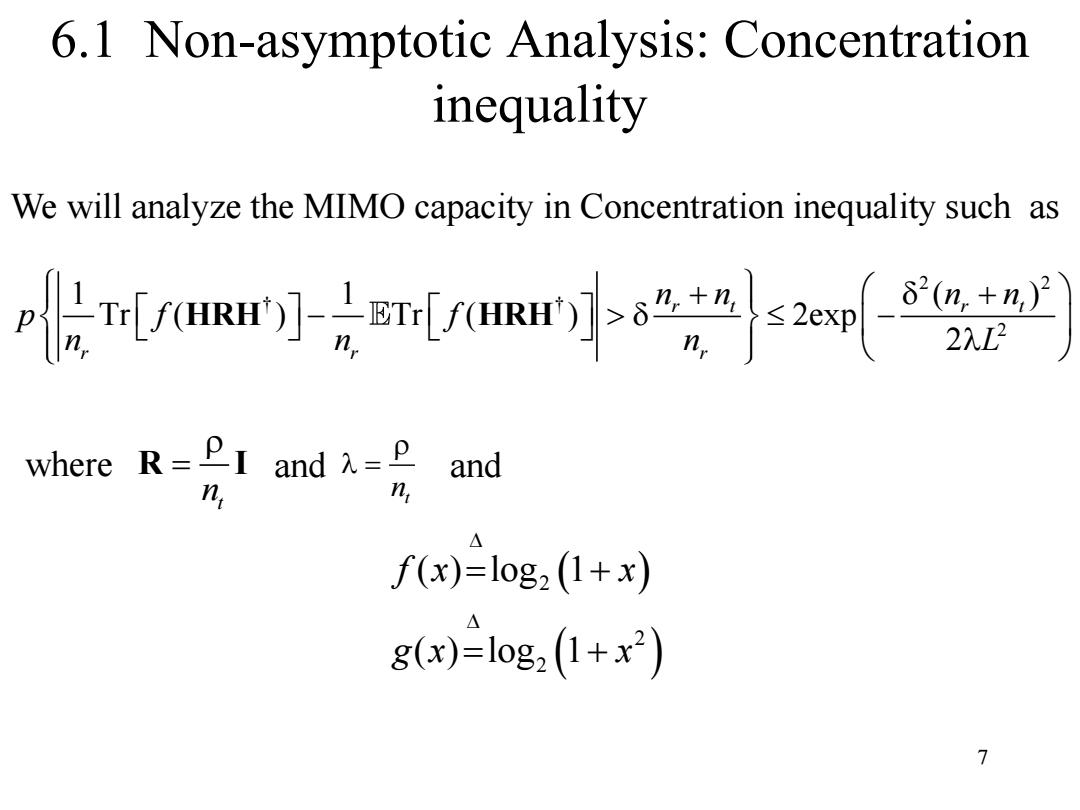
6.1 Non-asymptotic Analysis:Concentration inequality We will analyze the MIMO capacity in Concentration inequality such as P传w.k是n时iy小&sa(s) where R-PI and元-P and n n, f(x)=log2(1+x) g(x)=l1og2(1+x2) 7

7 6.1 Non-asymptotic Analysis: Concentration inequality We will analyze the MIMO capacity in Concentration inequality such as 2 2 2 † † 1 1 ( ) Tr ( ) Tr ( ) 2exp 2 r t r t r r r n n n n p n f n n L f     +  +             −    −         HRH HRH t n  where R I = and t n   = and ( ) ( ) 2 2 2 ( ) log 1 ( ) log 1 f x x g x x   = + = +

6.1 Non-asymptotic Analysis:Concentration inequality The Lipschitz norm of g(x) L=sup g(x)-8(y)-d2g(x)-1 x≠y x-y In 2 And we can get Tr[f(XRX')]=Tr[/(HRH)]->[(HRH)]=I0g.det(I+HRH)=C. The Concentration inequality of MIMO capacity can be written as δ2(n,+n,)尸n plC-[C]>δ(n,+n,)}≤2e2osd 8

8 6.1 Non-asymptotic Analysis: Concentration inequality The Lipschitz norm of g x( ) 2 2 ( ) ( ) ( ) 1 sup x y ln 2 g x g y d g x L  x y dx − = = = − ( ) ( ) ( ) 2 ( ) 1 † † † Tr =Tr log det . r n i i f f f C =       =  = + =     XRX HRH HRH I HRH    And we can get The Concentration inequality of MIMO capacity can be written as    ( ) 2 2 2 ( ) 2 2 t r t LS n n n c L t r p C C n n e  + −  −   + 

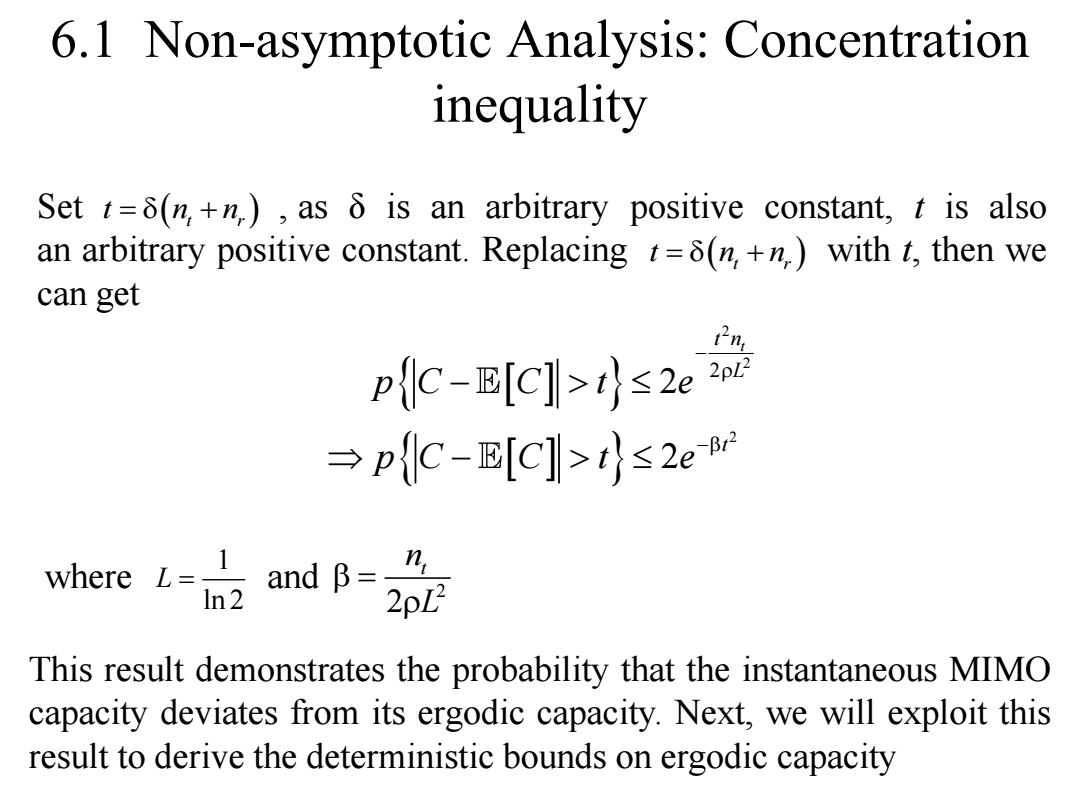
6.1 Non-asymptotic Analysis:Concentration inequality Sett=δ(n,+n,),asδis an arbitrary positive constant,t is also an arbitrary positive constant.Replacing t=5(n,+n)with t,then we can get 1'n, pC-E[C]>2e 2ot →plc-[C]>ts2er where im and B- n This result demonstrates the probability that the instantaneous MIMO capacity deviates from its ergodic capacity.Next,we will exploit this result to derive the deterministic bounds on ergodic capacity

6.1 Non-asymptotic Analysis: Concentration inequality Set , as δ is an arbitrary positive constant, t is also an arbitrary positive constant. Replacing with t, then we can get t n n =  + ( t r ) t n n =  + ( t r )         2 2 2 2 2 2 t t n L t p C C t e p C C t e −  −  −    −   where and 1 ln 2 L = 2 2 t n L  =  This result demonstrates the probability that the instantaneous MIMO capacity deviates from its ergodic capacity. Next, we will exploit this result to derive the deterministic bounds on ergodic capacity

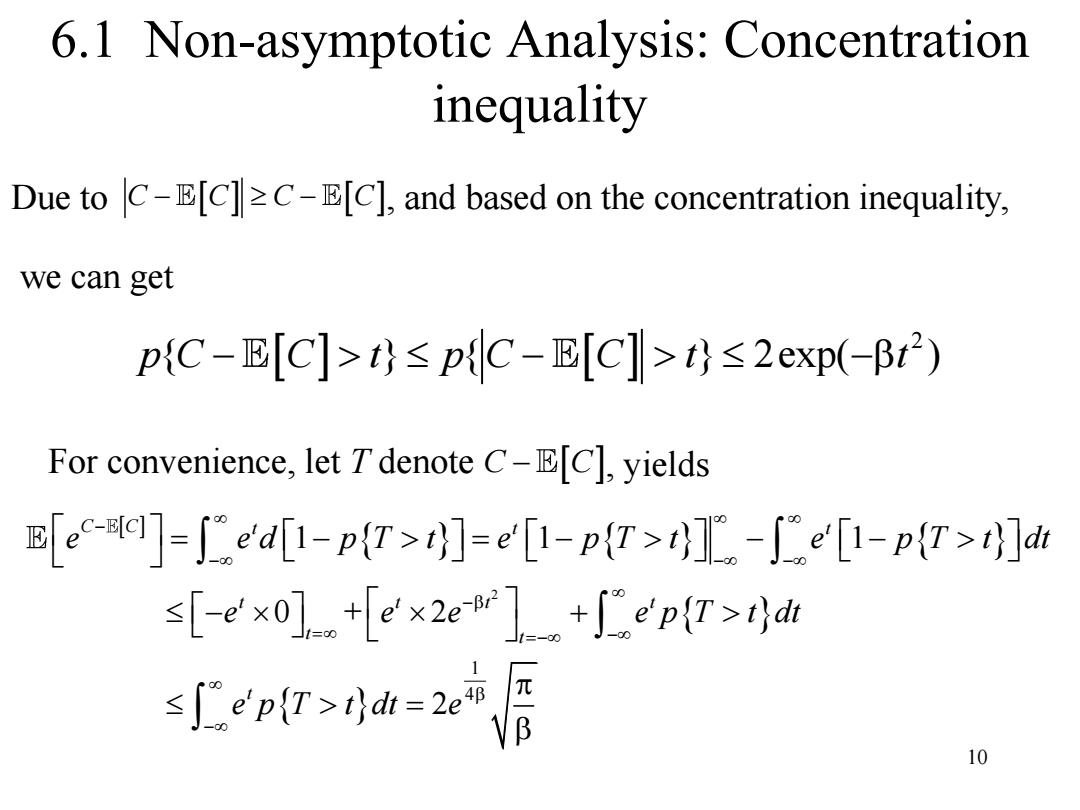
6.1 Non-asymptotic Analysis:Concentration inequality Due to C-E[C]=C-E[C],and based on the concentration inequality, we can get pC-[C]>≤plC-[C]>≤2exp(-Bt2) For convenience,let T denote C-E[C],yields [e-]=∫e'd1-p(T>]=e'[1-pT>]P-∫e1-pT>]d s[-e'xo]ex2ene'p(T>1di sfn7>=28 10

10 6.1 Non-asymptotic Analysis: Concentration inequality Due to C C C C −  −     , and based on the concentration inequality, we can get     2 p C C t p C C t t { } { } 2exp( ) −   −   − For convenience, let T denote C C −   , yields             2 1 4 1 + 1 1 0 2 2 C C t t t t t t t t t t e e d p T t e e p T t dt e e e e p T t dt e p p T t d T e t t   − − −  −  =  =− −  −  −      =  −   =  −          −     −   −     +         =     

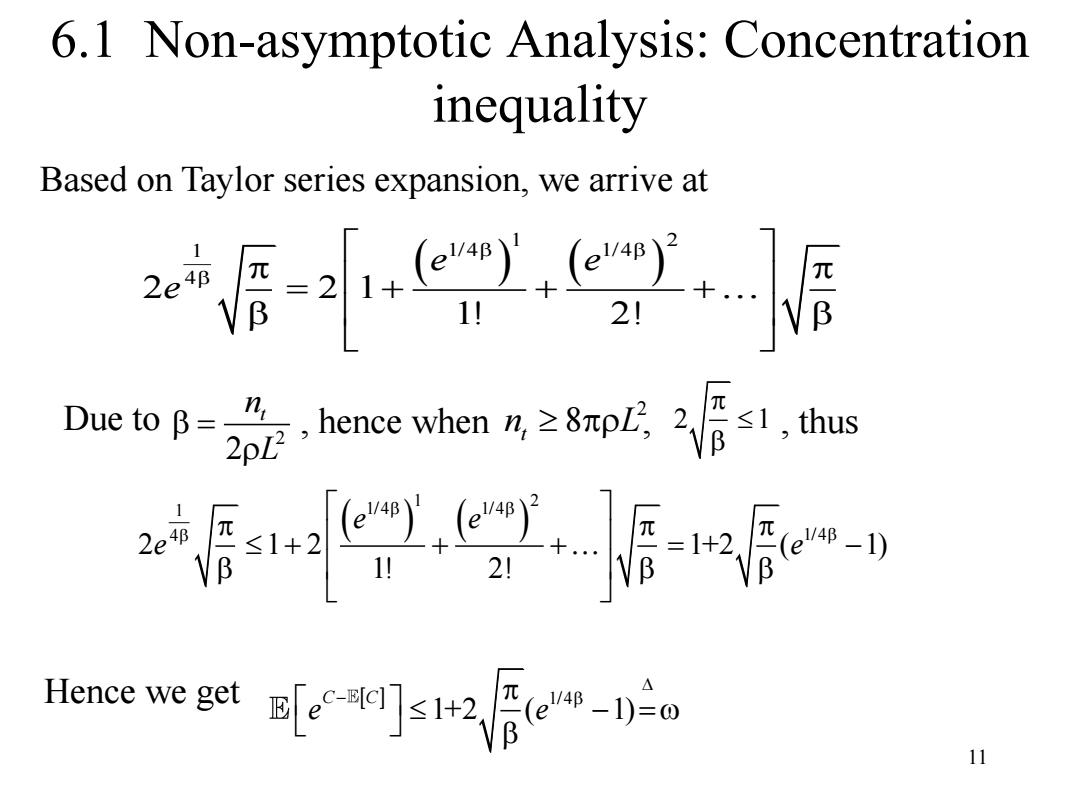
6.1 Non-asymptotic Analysis:Concentration inequality Based on Taylor series expansion,we arrive at -]小 DeoB=,henee wen%≥8p，叱2作1，his 5.学 Hnce ege[ew]s2辰e-2a 11

11 Based on Taylor series expansion, we arrive at 6.1 Non-asymptotic Analysis: Concentration inequality ( ) ( ) 1 2 1 1/4 1/4 4 2 2 1 1! 2! e e e        = + + +         Due to 2 2 t n L  =  , hence when , 2 8 t n L   2 1    , thus   1/4 1+2 ( 1) C C e e  −      − =    Hence we get ( ) ( ) 1 2 1 1/4 1/4 4 1/4 2 1 2 1+2 ( 1) 1! 2! e e e e           + + + = −         