
第四章级数 历安毛子代枝大学 XIDIAN UNIVERSITY Series 绝对收敛 若∑a,收敛,则称∑an为绝对收敛 n- 条件收敛 非绝对收敛的收敛级数称为条件收敛 注 Va+b≤a+b 若2,,绝对收敛,则2a,也绝对收敛 P 故: ∑a绝对收敛台∑4,∑b绝对收敛 场论与复变函数Field Theory and Complex Variable Functions 22
场论与复变函数 Field Theory and Complex Variable Functions 22 绝对收敛 1 1 若 收敛 则称 为绝对收敛 n n n n , 条件收敛 非绝对收敛的收敛级数称为条件收敛. 注 2 2 a b a b n n n n 1 1 1 n n n n n n a , b 若 绝对收敛,则 也绝对收敛 1 n n n n a , b 故: 绝对收敛 绝对收敛 第四章 级数 Series

第四章级数 历安毛子代枚大学 XIDIAN UNIVERSITY Series 判断复数项级数∑αn是否绝对收敛的方法: 方法一:判断正项级数∑1α,是否收敛, h- 方法二:分出a的实部an与虚部bn, 判断级数∑a,与∑b,是否绝对收敛 n= 场论与复变函数Field Theory and Complex Variable Functions 23
场论与复变函数 Field Theory and Complex Variable Functions 23 1 : n n 判断复数项级数 是否绝对收敛的方法 1 | | n n 方法一:判断正项级数 是否收敛, 1 1 : , . n n n n n n n a b a b 方法二 分出 的实部 与虚部 判断级数 与 是否绝对收敛 第四章 级数 Series
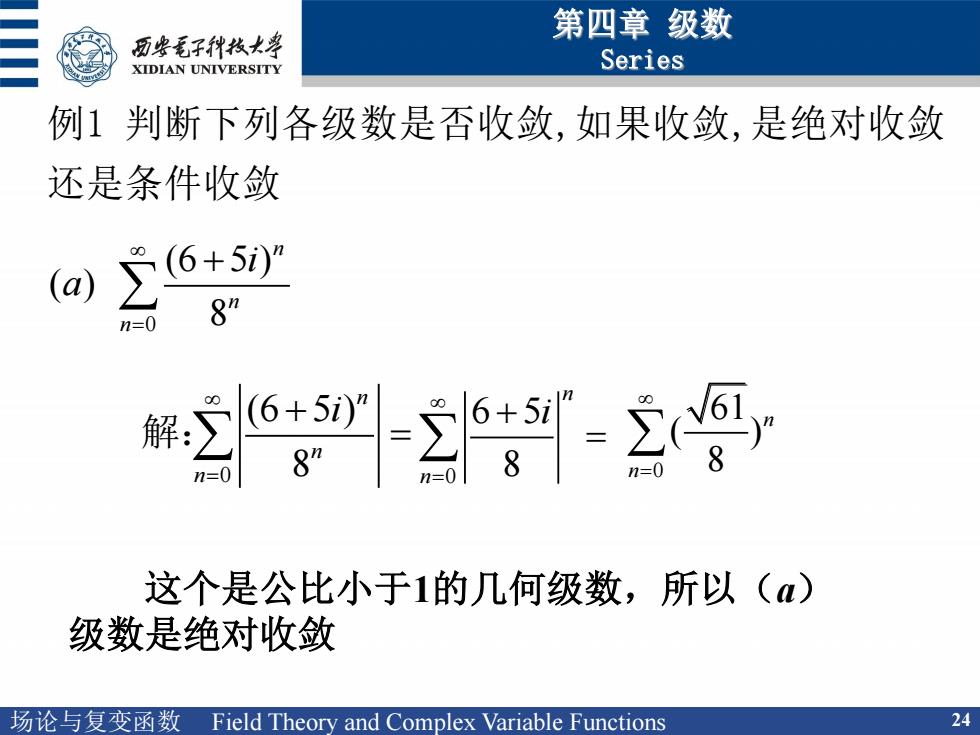
第四章级数 历些毛子代枝大学 XIDIAN UNIVERSITY Series 例1判断下列各级数是否收敛,如果收敛,是绝对收敛 还是条件收敛 (a) 6 n=0 8” 含- 这个是公比小于1的几何级数,所以() 级数是绝对收敛 场论与复变函数Field Theory and Complex Variable Functions 24
场论与复变函数 Field Theory and Complex Variable Functions 24 0 (6 5 ) ( ) 8 n n n i a 0 (6 5 ) 8 n n n i 解: 这个是公比小于1的几何级数,所以(a) 级数是绝对收敛 0 6 5 8 n n i 0 61 ( ) 8 n n 例1 判断下列各级数是否收敛,如果收敛,是绝对收敛 还是条件收敛 第四章 级数 Series
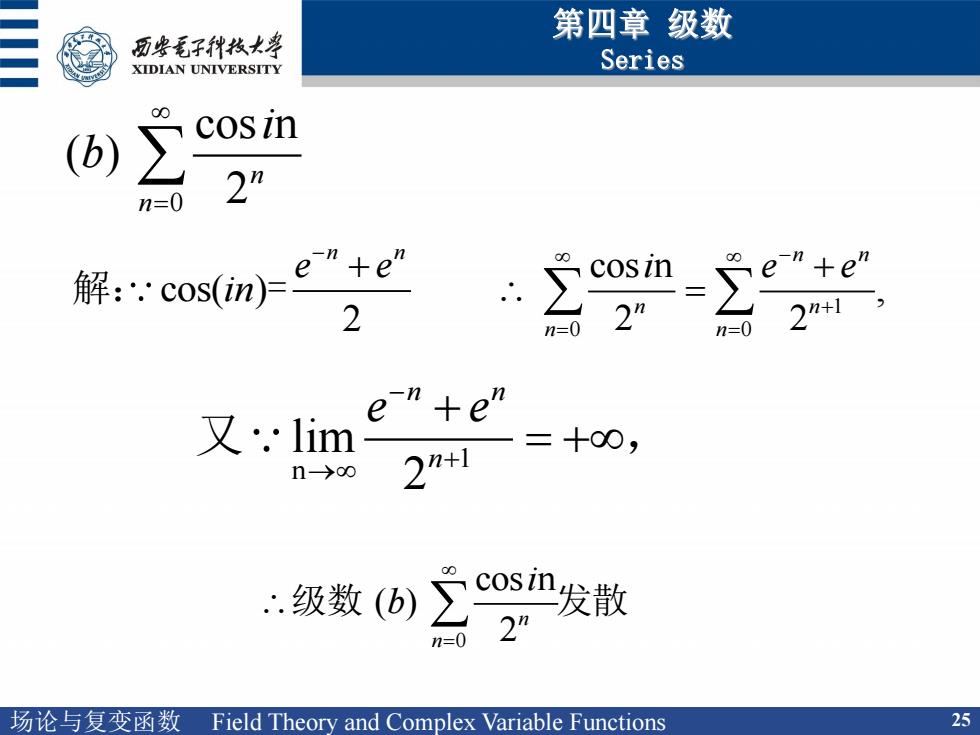
第四章级数 历安毛子代枚大学 XIDIAN UNIVERSITY Series 00 cosin (b) n=0 2" 解:cos(ime”+e 0 cosm-e"+e 0 2 2” 2n+1 又.lim e"+e -=十00, n->0 2n+1 :级数立发故 n=0 场论与复变函数Field Theory and Complex Variable Functions 25
场论与复变函数 Field Theory and Complex Variable Functions 25 0 cos n ( ) 2 n n i b cos( ) 2 n n e e in 解: = 1 0 0 cos n , 2 2 n n n n n n i e e 1 n lim 2 n n n e e 又 , 0 cos n ( ) 2 n n i b 级数 发散 第四章 级数 Series
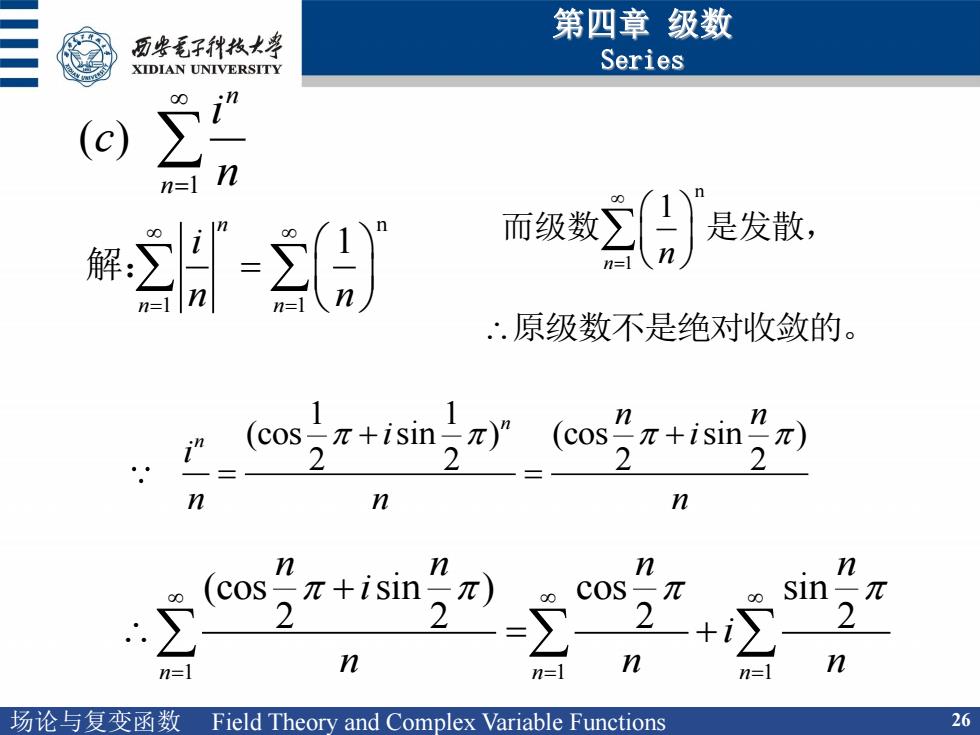
第四章级数 历安毛子代枝大学 XIDIAN UNIVERSITY Series 00 (c) n=I n ¥-2 而级数 是发散, n=1 n ·.原级数不是绝对收敛的。 1 1 次 (cos+isin () n n n (co n n n π+isin -元 00 sin =习 n= n n= n 场论与复变函数Field Theory and Complex Variable Functions 26
场论与复变函数 Field Theory and Complex Variable Functions 26 1 ( ) n n i c n 1 1 (cos sin ) (cos sin ) 2 2 2 2 n n n n i i i n n n n 1 1 1 n n n i n n 解: n 1 1 n n 而级数 是发散, 原级数不是绝对收敛的。 1 1 1 (cos sin ) cos sin 2 2 2 2 n n n n n n n i i n n n 第四章 级数 Series