FD for arbitrary loading The procedure is the same for periodic and non-periodic excitation: Express f(t)into terms of harmonic components Evaluate the response of each component Superpose the harmonic response to get total res. For non-periodic excitation,however Extend the Fourier series concept to non-periodic excitation! 闺 土本程李悦
FD for arbitrary loading • The procedure is the same for periodic and non-periodic excitation: Express f(t) into terms of harmonic components Evaluate the response of each component Superpose the harmonic response to get total res. • For non-periodic excitation, however Extend the Fourier series concept to non-periodic excitation!
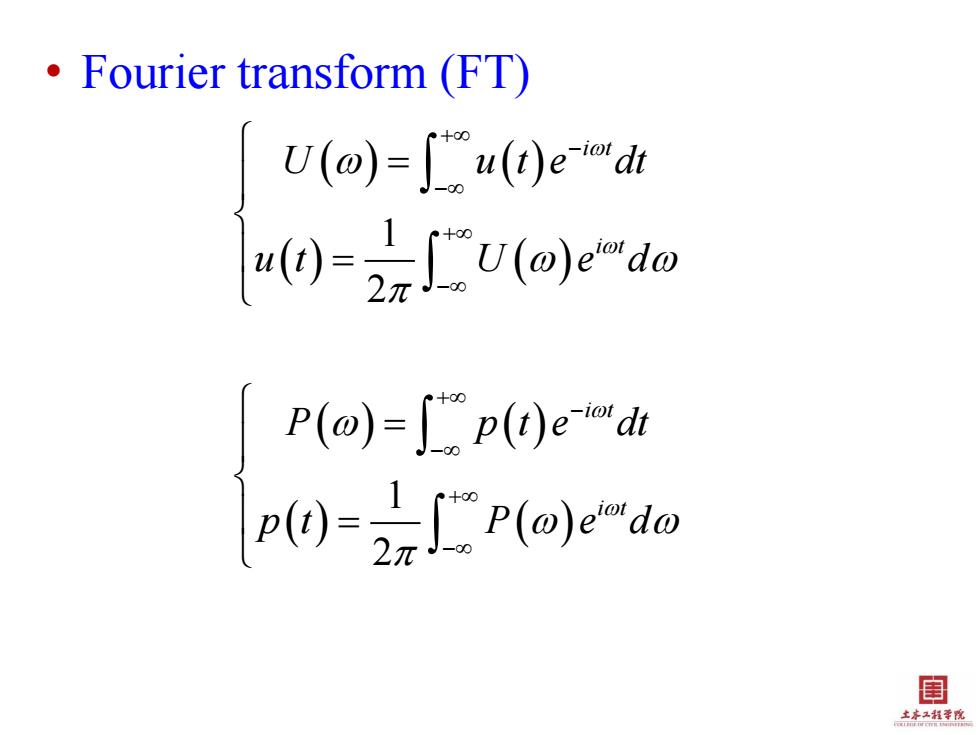
Fourier transform(FT) U()=u(t)edi u-()do P()=p(t)edi p回=2tJrP(o)eda 闺 土水2程季院
• Fourier transform (FT) 1 2 i t i t U u t e dt u t U e d 1 2 i t i t P p t e dt p t P e d
Complex expression of Fourier series 目 土本程李悦
• Complex expression of Fourier series
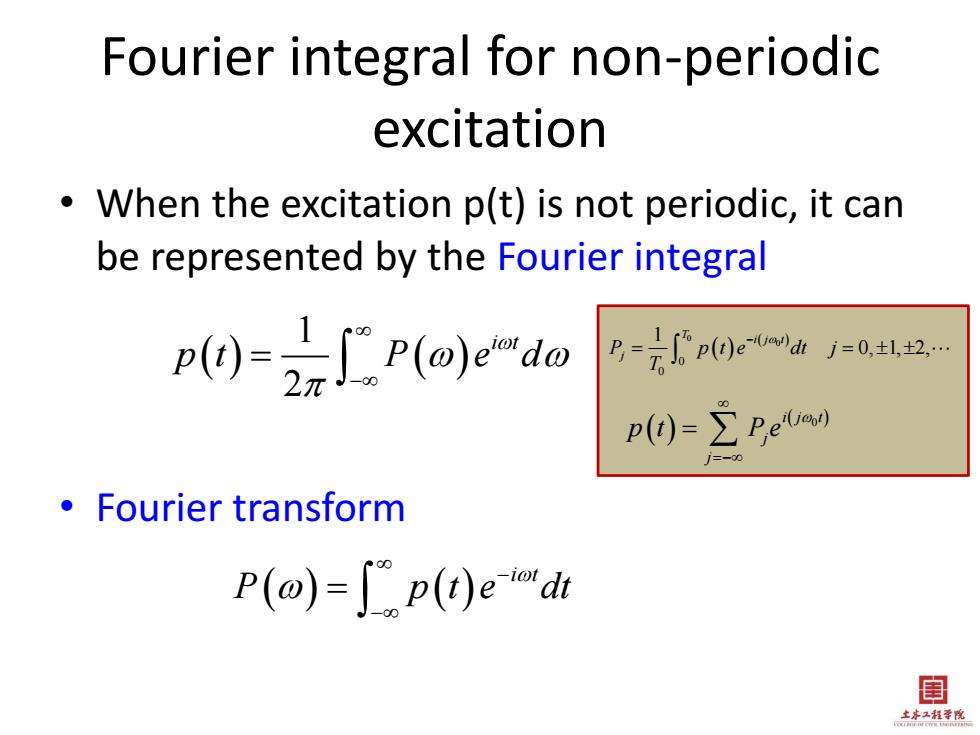
Fourier integral for non-periodic excitation When the excitation p(t)is not periodic,it can be represented by the Fourier integral p0=2元P(o)eda 月=p0ewhj=0+l2 pd=∑Pea Fourier transform P(o)=p(t)e dt 目 主茅2相季院
Fourier integral for non-periodic excitation • When the excitation p(t) is not periodic, it can be represented by the Fourier integral • Fourier transform 0 0 0 0 1 0, 1, 2, T i j t P p t e dt j j T i j t 0 j j p t P e 1 2 i t p t P e d i t P p t e dt
Treating the non-period function as a function with infinity period,the frequency response analysis method can then be expanded to arbitrary excitation. Fourier series vs.Fourier transform? Discrete function vs.continues function? Periodic excitation vs.non-periodic excitation what's the relationship between them?! 闺 土水2鞋学悦
• Treating the non-period function as a function with infinity period, the frequency response analysis method can then be expanded to arbitrary excitation. • Fourier series vs. Fourier transform? • Discrete function vs. continues function? • Periodic excitation vs. non-periodic excitation what’s the relationship between them?!