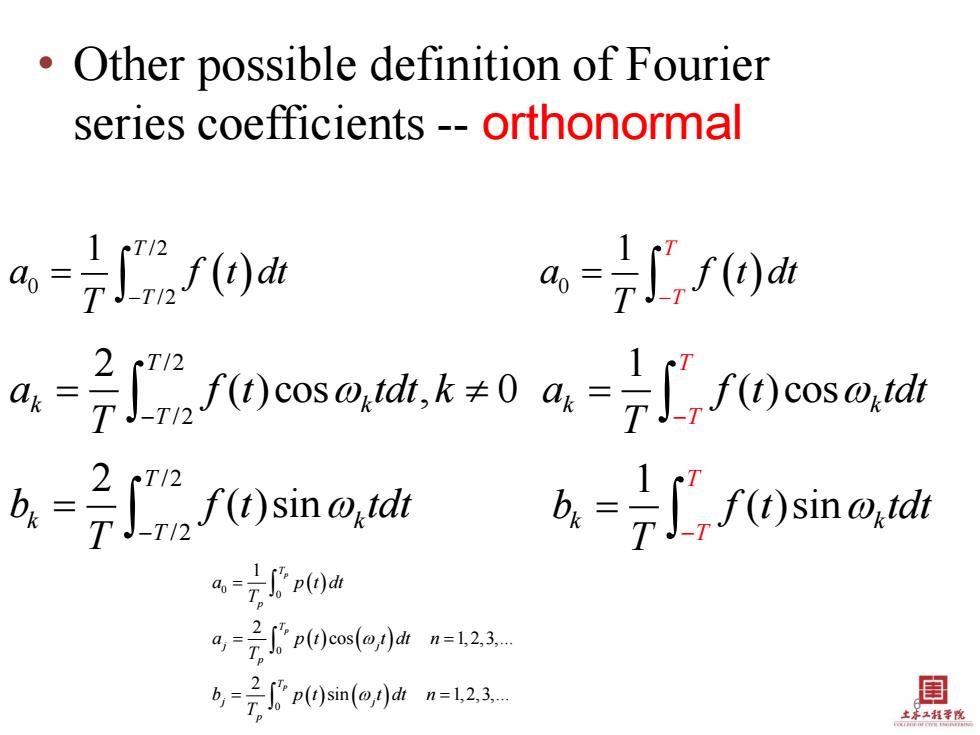
Other possible definition of Fourier series coefficients--orthonormal a=/)边 a-IS,f()d a-2f0esu灿k0&=7,Wosh ()sino.dr b,=7J,f④)sinw,d a=元p0 p()coste)d1.2. ()sin(.3 国 士系2鞋学院
6 / 2 / 2 2 ( )cos , 0 T k k T a f t tdt k T / 2 / 2 2 ( )sin T k k T b f t tdt T / 2 0 / 2 1 T T a f t dt T • Other possible definition of Fourier series coefficients -- orthonormal 0 1 T T a f t dt T 1 ( )cos k k T T a f t tdt T 1 ( )sin k k T T b f t tdt T 0 0 0 0 1 2 cos 1,2,3,... 2 sin 1,2,3,... p p p T p T j j p T j j p a p t dt T a p t t dt n T b p t t dt n T

Steady state response to each harmonic component Frequency ratio B=n ao uo=ao /k a,coso4268,sino,+(1-Bcos (1-P,2+(2B,) smo冬下gmo1-2 (1-B,2)+(2B,) 闺 土本程李悦
0 a 0 0 u a k / / j j n cos j j a t 2 2 2 2 2 sin 1 cos 1 2 j j j j c j j j j a t t u k sin j j b t 2 2 2 2 1 sin 2 cos 1 2 j j j j s j j j j b t t u k : , : • Steady state response to each harmonic component Frequency ratio

The total steady state response to the periodic excitation u(0)=4+∑(g,+%,) k a248,)+b1-B1mo 合k(1-B2+(2B,)j 分1a1-B)-b(23, cos@t 合k((1-B,)+(2β,) 闺 土本之程季院
0 1 0 2 2 2 2 1 2 2 2 2 1 1 2 1 sin 1 2 1 1 2 cos 1 2 c s j j j j j j j j j j j j j j j j j j j u t u u u a k a b t k a b t k • The total steady state response to the periodic excitation
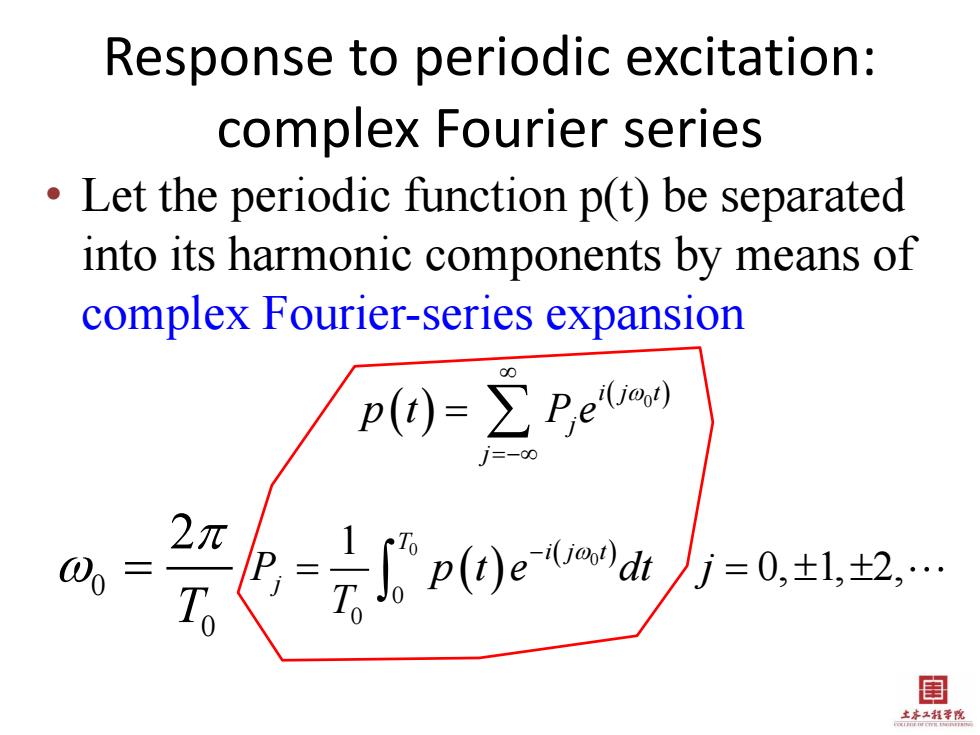
Response to periodic excitation: complex Fourier series Let the periodic function p(t)be separated into its harmonic components by means of complex Fourier-series expansion p()=∑PeaW 2 00= 7p0em-01*2 T 闺 土本程李悦
Response to periodic excitation: complex Fourier series • Let the periodic function p(t) be separated into its harmonic components by means of complex Fourier-series expansion i j t 0 j j p t P e 0 0 2 T 0 0 0 0 1 0, 1, 2, T i j t P p t e dt j j T
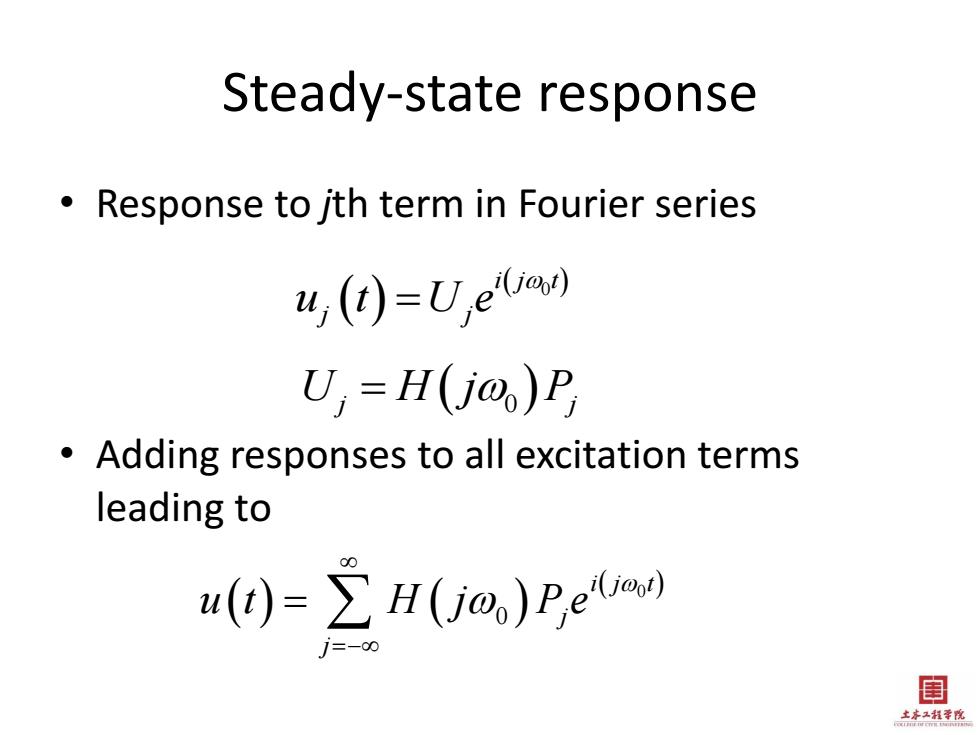
Steady-state response Response to jth term in Fourier series 4,())=U,eUa U;=H(j)P, Adding responses to all excitation terms leading to ()=∑H(Uo)PeUa 闺 土本2程学院
Steady-state response • Response to jth term in Fourier series • Adding responses to all excitation terms leading to i j t 0 j j u t U e U H j P j j 0 0 0 i j t j j u t H j P e