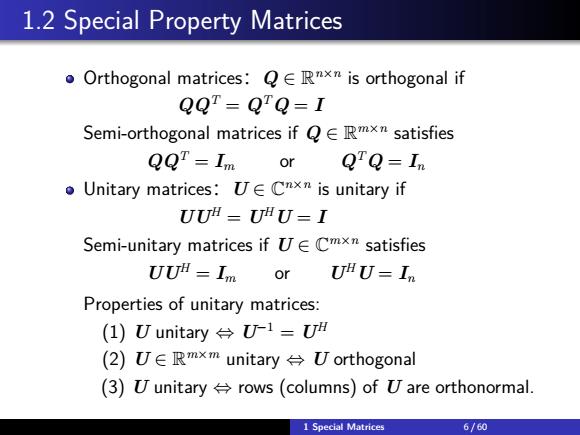
1.2 Special Property Matrices Orthogonal matrices:QE Rnxn is orthogonal if QQT-QTQ-I Semi-orthogonal matrices if QE Rmxn satisfies QQT=Ii or QTQ-In Unitary matrices:UE Cnxn is unitary if UUH-UHU=I Semi-unitary matrices if UE Cmxm satisfies UUH=Im or UHU=In Properties of unitary matrices: (1)U unitary台U1=UH (2)U∈Rmxm unitary÷U orthogonal (3)U unitary rows(columns)of U are orthonormal. 1 Special Matrices 6/60
1.2 Special Property Matrices Orthogonal matrices:Q ∈ R n×n is orthogonal if QQT = QTQ = I Semi-orthogonal matrices if Q ∈ R m×n satisfies QQT = Im or QTQ = In Unitary matrices:U ∈ C n×n is unitary if UUH = UHU = I Semi-unitary matrices if U ∈ C m×n satisfies UUH = Im or UHU = In Properties of unitary matrices: (1) U unitary ⇔ U−1 = UH (2) U ∈ R m×m unitary ⇔ U orthogonal (3) U unitary ⇔ rows (columns) of U are orthonormal. 1 Special Matrices 6 / 60
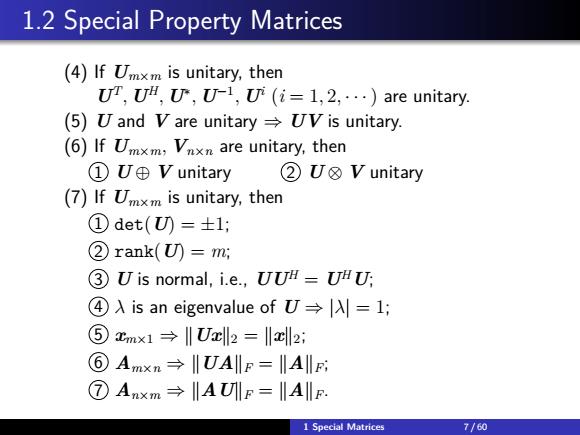
1.2 Special Property Matrices (4)If Umxm is unitary,then UT,UH,U,U-1,Ui (i=1,2,...)are unitary. (5)U and V are unitary=UV is unitary. (6)If Umxm,Vnxn are unitary,then ①U⊕V unitary ②U⑧V unitary (7)If Umxm is unitary,then ①det(U0=±l: ②rank(U)=m: 3 U is normal,i.e.,UU=UHU: ④入is an eigenvalue of U→lN=l; ⑤xmx1→‖Ux2=‖l2; ⑥Amxn→‖UAlF=‖AlF ⑦Anxm→‖AUlF=‖A‖lF. 1 Special Matrices 7/60
1.2 Special Property Matrices (4) If Um×m is unitary, then UT, UH, U∗ , U−1 , Ui (i = 1, 2, · · ·) are unitary. (5) U and V are unitary ⇒ UV is unitary. (6) If Um×m, Vn×n are unitary, then 1 U ⊕ V unitary 2 U ⊗ V unitary (7) If Um×m is unitary, then 1 det(U) = ±1; 2 rank(U) = m; 3 U is normal, i.e., UUH = UHU; 4 λ is an eigenvalue of U ⇒ |λ| = 1; 5 xm×1 ⇒ kUxk2 = kxk2; 6 Am×n ⇒ kUAkF = kAkF; 7 An×m ⇒ kAUkF = kAkF. 1 Special Matrices 7 / 60

1.2 Special Property Matrices Unitary transformations:If U unitary,the linear transformation Ux is called an unitary transformation of 2. Unitary equivalence:If U unitary,the matrices B=UHAU and A are unitary equivalent.In particular,if U orthogonal,B and A are orthogonal equivalent. Application:Givens or Householder transformation, eigen-decomposition. ●Normal matrices: A E Cnxn satisfies AHA=AAH. Properties:Normal matrices can be unitary diagonal,i.e.,if A is normal,then there is an unitary matrix U such that UHAU=D where D is diagonal. 1 Special Matrices 8/60
1.2 Special Property Matrices Unitary transformations: If U unitary, the linear transformation Ux is called an unitary transformation of x. Unitary equivalence: If U unitary, the matrices B = UHAU and A are unitary equivalent. In particular, if U orthogonal, B and A are orthogonal equivalent. Application: Givens or Householder transformation, eigen-decomposition. Normal matrices: A ∈ C n×n satisfies AHA = AAH. Properties: Normal matrices can be unitary diagonal, i.e., if A is normal, then there is an unitary matrix U such that UHAU = D where D is diagonal. 1 Special Matrices 8 / 60
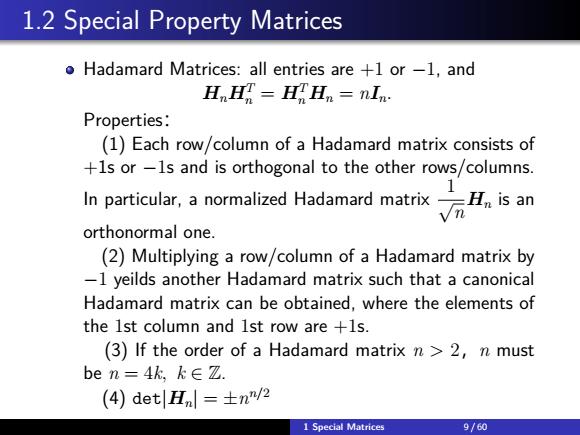
1.2 Special Property Matrices o Hadamard Matrices:all entries are +1 or-1,and HnHT=HTHn nIn. Properties: (1)Each row/column of a Hadamard matrix consists of +1s or-1s and is orthogonal to the other rows/columns. In particular,a normalized Hadamard matrix-H is an Vn orthonormal one. (2)Multiplying a row/column of a Hadamard matrix by -1 yeilds another Hadamard matrix such that a canonical Hadamard matrix can be obtained,where the elements of the lst column and 1st row are +1s (3)If the order of a Hadamard matrix n>2,n must ben=4k,k∈Z (4)det H=±n/2 1 Special Matrices 9/60
1.2 Special Property Matrices Hadamard Matrices: all entries are +1 or −1, and HnHT n = HT n Hn = nIn. Properties: (1) Each row/column of a Hadamard matrix consists of +1s or −1s and is orthogonal to the other rows/columns. In particular, a normalized Hadamard matrix 1 √ n Hn is an orthonormal one. (2) Multiplying a row/column of a Hadamard matrix by −1 yeilds another Hadamard matrix such that a canonical Hadamard matrix can be obtained, where the elements of the 1st column and 1st row are +1s. (3) If the order of a Hadamard matrix n > 2,n must be n = 4k, k ∈ Z. (4) det|Hn| = ±n n/2 1 Special Matrices 9 / 60

1.2 Special Property Matrices o Similar matrices:a nonsingular S Cnx"such that B=S-1AS,denoted by BA. AS-AS is called a similar transformation of A. Properites: (1)Reflexivity:A~A. (2)Symmetry:If A~B,then B~A (3)Transitivity:If A~B and B~C,then A~C. (4)If B A,then Bl Ak,kEN+. (5)If invertible B~A,then B-1~A-1. (6)If B~A,then det(B)det(A). det(B)=det(S-1AS)=det(S-1)det(A)det(S)=det(A) (7)If BA,then tr(B)tr(A). tr(B)=tr(S-1AS)=tr(ASS-1)=tr(A) 1 Special Matrices 10/60
1.2 Special Property Matrices Similar matrices: ∃ a nonsingular S ∈ C n×n such that B = S −1AS, denoted by B ∼ A. A 7→ S −1AS is called a similar transformation of A. Properites: (1) Reflexivity: A ∼ A. (2) Symmetry: If A ∼ B, then B ∼ A. (3) Transitivity: If A ∼ B and B ∼ C, then A ∼ C. (4) If B ∼ A, then Bk ∼ Ak , k ∈ N +. (5) If invertible B ∼ A, then B−1 ∼ A−1 . (6) If B ∼ A, then det(B) = det(A). det(B) = det(S −1AS) = det(S −1 ) det(A) det(S) = det(A) (7) If B ∼ A, then tr(B) = tr(A). tr(B) = tr(S −1AS) = tr(ASS−1 ) = tr(A) 1 Special Matrices 10 / 60