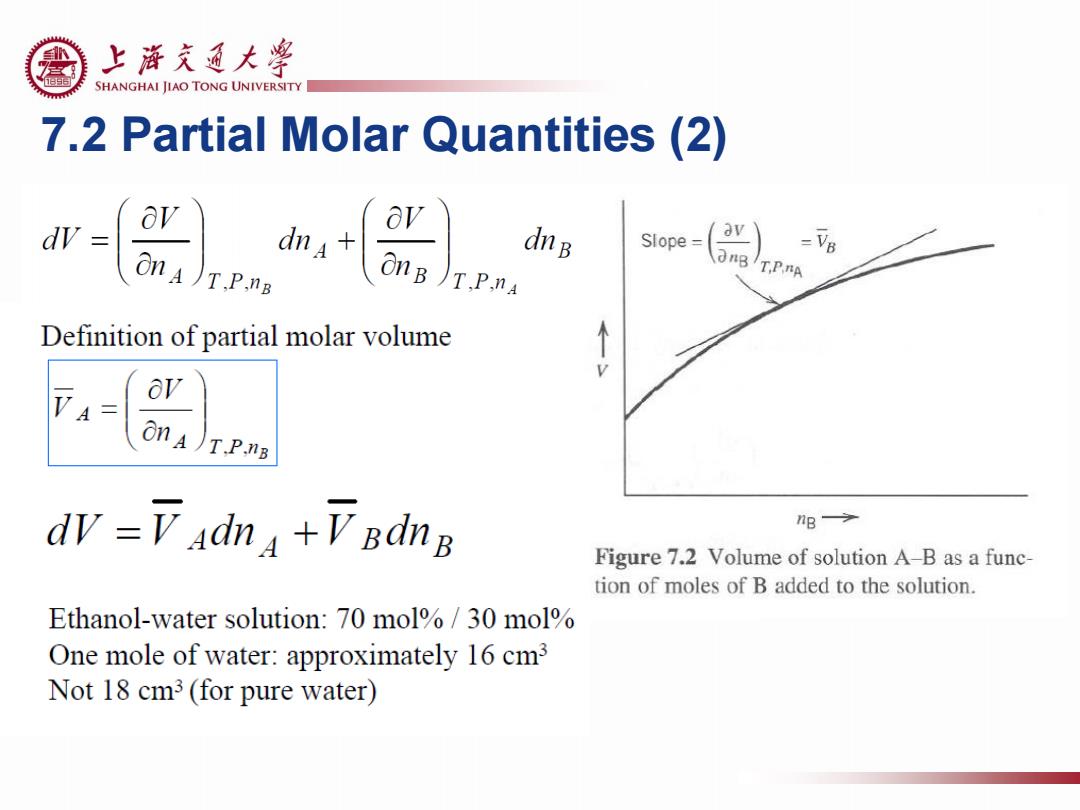
上游充通大¥ SHANGHAI JIAO TONG UNIVERSITY 7.2 Partial Molar Quantities (2) dV av Slope= = onA)T.P.nB dna dnB OnB )T.P.na T.P.nA Definition of partial molar volume ov VA on A)T.P.nB dv =V adna +V BdnB nB Figure 7.2 Volume of solution A-B as a func- tion of moles of B added to the solution. Ethanol-water solution:70 mol%/30 mol One mole of water:approximately 16 cm3 Not 18 cm3 (for pure water)
7.2 Partial Molar Quantities (2)
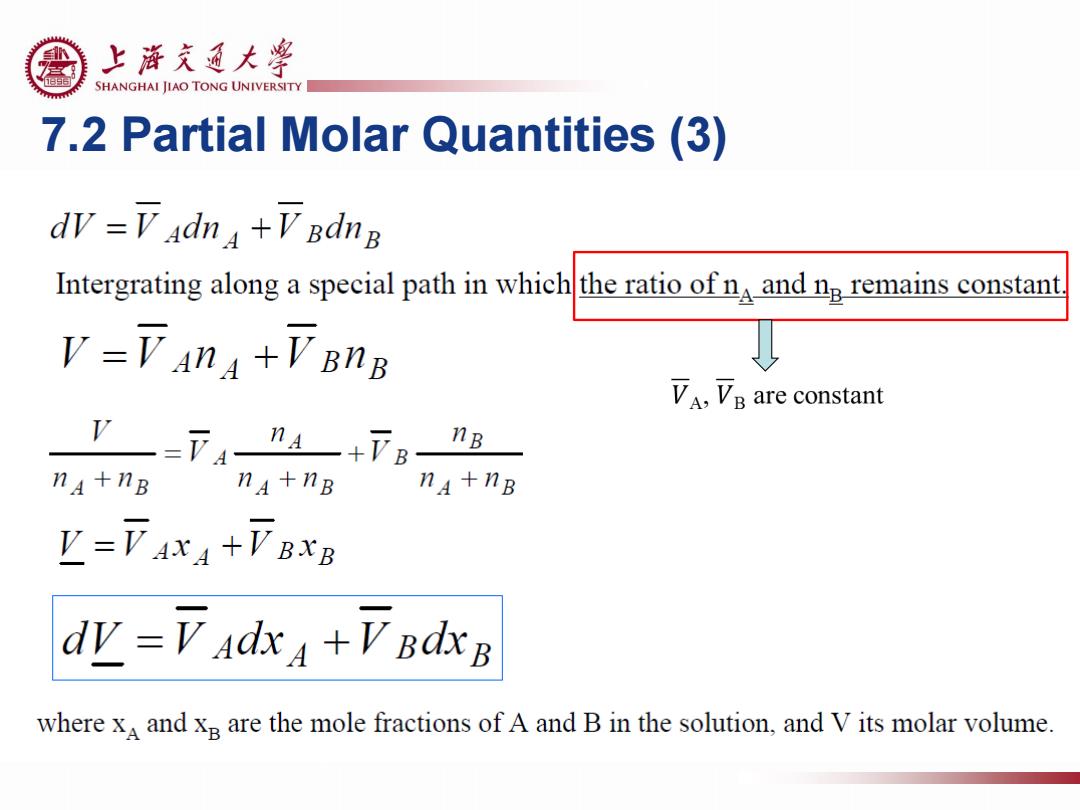
上游充通大粤 SHANGHAI JIAO TONG UNIVERSITY 7.2 Partial Molar Quantities (3) dW=下4dn4+V Bdng Intergrating along a special path in whichthe ratio of n and n remains constant V=下An4+VBnB V,Ve are constant nB na+nB na+nB na+nB V=VAXA+VBXB dv=V adxa+VBdxB where x and xB are the mole fractions of A and B in the solution,and V its molar volume
7.2 Partial Molar Quantities (3)
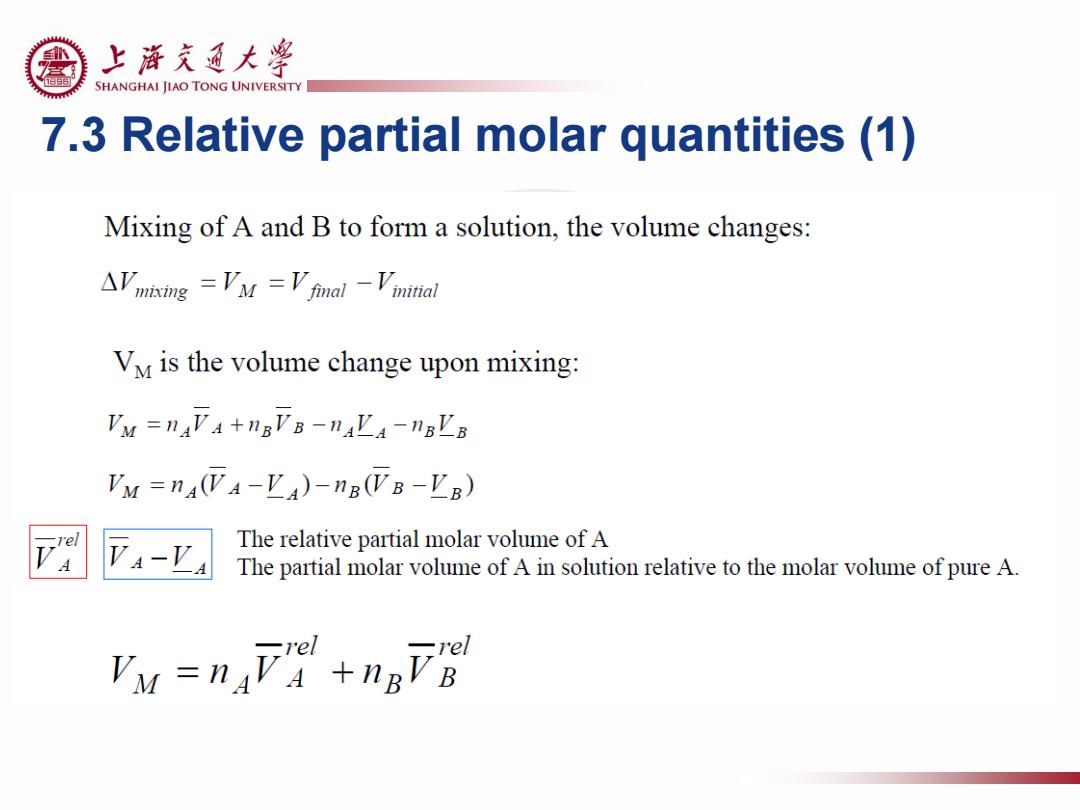
上游充通大粤 SHANGHAI JIAO TONG UNIVERSITY 7.3 Relative partial molar quantities (1) Mixing of A and B to form a solution,the volume changes: AVmkmng =V'M =Vfmal -Vmital Vy is the volume change upon mixing: Vu =nAVA+nBVB-naLa-nBLB Vu =nA(VA-VA)-nB(VB-EB) 4-'a The relative partial molar volume of a The partial molar volume of a in solution relative to the molar volume of pure A. rel rel VM =nav+nBVB
7.3 Relative partial molar quantities (1)

上游充通大粤 SHANGHAI JIAO TONG UNIVERSITY 7.3 Relative partial molar quantities (2) 乃M=nF+nFB For one mole of solution. 卫M=xAF+aFB U,F,G,H,S,V The equations for variations of thermodynamic properties with temperature, pressure,and so on apply to solutions as well as to pure components. dGr=-SdT G=G4-G=RTIn@A
7.3 Relative partial molar quantities (2)
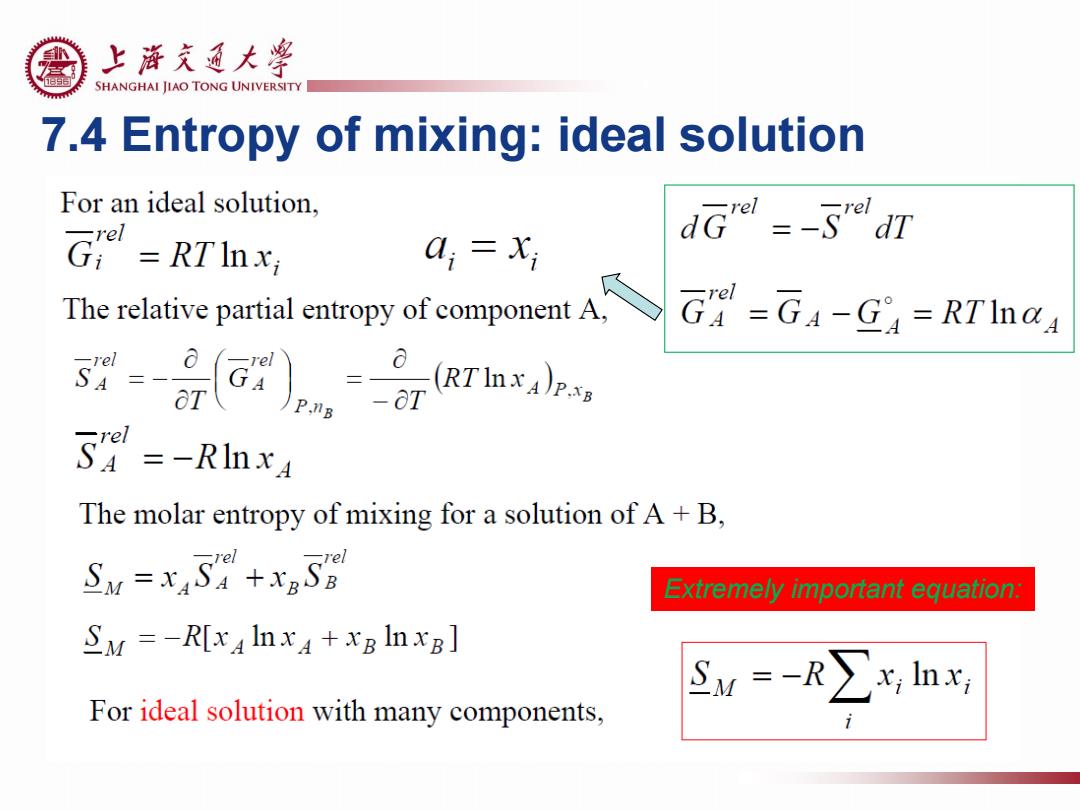
上浒充通大警 SHANGHAI JIAO TONG UNIVERSITY 7.4 Entropy of mixing:ideal solution For an ideal solution, Gr =RTInx: dG =-5"dT 0,=X; The relative partial entropy of component A, G=G4-G=RTInaA 54=-RInxA The molar entropy of mixing for a solution of A+B, SN =xAS+x5 Extremely important equation: SM=-R[x4Inx4+xBInxB] SM=-R∑x,lnx For ideal solution with many components
7.4 Entropy of mixing: ideal solution Extremely important equation: