Example In the configuration in Fig.5.1.1,the first spring is stretched x units and the second by y-x units. 4口1y1至,12000 Linear of Differential Equations
Example In the configuration in Fig.5.1.1, the first spring is stretched x units and the second by y−x units. We apply Newton’s law of motion to the two “free body diagrams” shown in Fig.5.1.2; we thereby obtain the system m1x 00 = −k1x+k2(y−x) (1) m2y 00 = −k2(y−x) +f(t) of differential equations that the position function x(t) and y(t) must satisfy. For instance, if m1 = 2,m2 = 1, k1 = 4, k2 = 2, and f(t) = 40 sin 3t in appropriate physical units, then the system in (1) reduces to 2x 00 = −6x+2y (2) y 00 = 2x−2y+40 sin 3t. Linear Systems of Differential Equations
Example In the configuration in Fig.5.1.1,the first spring is stretched x units and the second by y-x units.We apply Newton's law of motion to the two "free body diagrams"shown in Fig.5.1.2;we thereby obtain the system m1x”=-k1x+k20y-) (1) m2y”=-k20y-x)+ft) of differential equations that the position function x(t)and y(t) must satisfy. 4口10,1至,1无13000 Linear of Differential Equations
Example In the configuration in Fig.5.1.1, the first spring is stretched x units and the second by y−x units. We apply Newton’s law of motion to the two “free body diagrams” shown in Fig.5.1.2; we thereby obtain the system m1x 00 = −k1x+k2(y−x) (1) m2y 00 = −k2(y−x) +f(t) of differential equations that the position function x(t) and y(t) must satisfy. For instance, if m1 = 2,m2 = 1, k1 = 4, k2 = 2, and f(t) = 40 sin 3t in appropriate physical units, then the system in (1) reduces to 2x 00 = −6x+2y (2) y 00 = 2x−2y+40 sin 3t. Linear Systems of Differential Equations

Example In the configuration in Fig.5.1.1,the first spring is stretched x units and the second by y-x units.We apply Newton's law of motion to the two "free body diagrams"shown in Fig.5.1.2;we thereby obtain the system m1x”=-k1x+k2y-x) (1) m2y”=-k20y-x)+f(t) of differential equations that the position function x()and y(t) must satisfy.For instance,if m=2,m2=1,k1=4,k2 =2,and f(t)=40sin3t in appropriate physical units,then the system in (1)reduces to 2”=-6x+2y (2) y”=2x-2y+40sin3t. Linear of Differential Equations
Example In the configuration in Fig.5.1.1, the first spring is stretched x units and the second by y−x units. We apply Newton’s law of motion to the two “free body diagrams” shown in Fig.5.1.2; we thereby obtain the system m1x 00 = −k1x+k2(y−x) (1) m2y 00 = −k2(y−x) +f(t) of differential equations that the position function x(t) and y(t) must satisfy. For instance, if m1 = 2,m2 = 1, k1 = 4, k2 = 2, and f(t) = 40 sin 3t in appropriate physical units, then the system in (1) reduces to 2x 00 = −6x+2y (2) y 00 = 2x−2y+40 sin 3t. Linear Systems of Differential Equations
The Method of Elimination Example Find the particular solution of the system x=4x-3y,y=6x-7y (3) that satisfies the initial conditions x(0)=2,y(0)=-1. 4口14①yt至2000 Linear of Differential Equations
The Method of Elimination Example Find the particular solution of the system x 0 = 4x−3y, y 0 = 6x−7y (3) that satisfies the initial conditions x(0) = 2, y(0) = −1. Solution: If we solve the second equation in (3) for x, we get x = 1 6 y 0 + 7 6 y, (4) so that x 0 = 1 6 y 00 + 7 6 y 0 . (5) Linear Systems of Differential Equations
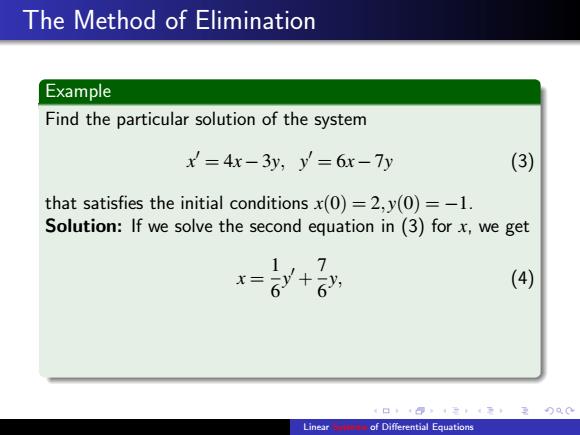
The Method of Elimination Example Find the particular solution of the system X=4x-3y,y=6x-7y (3) that satisfies the initial conditions x(0)=2,y(0)=-1 Solution:If we solve the second equation in(3)for x,we get (4) 4口10y至,元2000 Linear of Differential Equations
The Method of Elimination Example Find the particular solution of the system x 0 = 4x−3y, y 0 = 6x−7y (3) that satisfies the initial conditions x(0) = 2, y(0) = −1. Solution: If we solve the second equation in (3) for x, we get x = 1 6 y 0 + 7 6 y, (4) so that x 0 = 1 6 y 00 + 7 6 y 0 . (5) Linear Systems of Differential Equations